On the Stochastic Cahn-Hilliard/Allen-Cahn equation - Isaac Newton ...
On the Stochastic Cahn-Hilliard/Allen-Cahn equation - Isaac Newton ...
On the Stochastic Cahn-Hilliard/Allen-Cahn equation - Isaac Newton ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
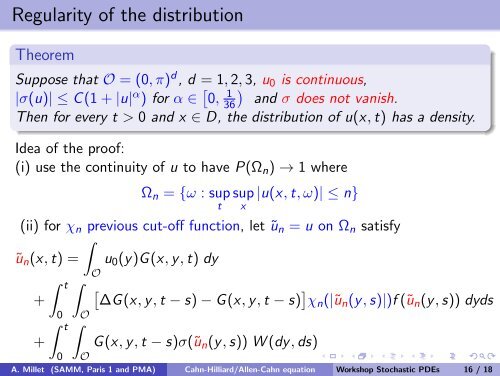
Regularity of <strong>the</strong> distribution<br />
Theorem<br />
Suppose that O = (0, π) d , d = 1, 2, 3, u0 is continuous,<br />
|σ(u)| ≤ C(1 + |u| α ) for α ∈ � 0, 1<br />
�<br />
36 and σ does not vanish.<br />
Then for every t > 0 and x ∈ D, <strong>the</strong> distribution of u(x, t) has a density.<br />
Idea of <strong>the</strong> proof:<br />
(i) use <strong>the</strong> continuity of u to have P(Ωn) → 1 where<br />
Ωn = {ω : sup<br />
t<br />
sup |u(x, t, ω)| ≤ n}<br />
x<br />
(ii) for χn previous cut-off function, let ũn = u on Ωn satisfy<br />
�<br />
ũn(x, t) = u0(y)G(x, y, t) dy<br />
O<br />
� t �<br />
� �<br />
+ ∆G(x, y, t − s) − G(x, y, t − s) χn(|ũn(y, s)|)f (ũn(y, s)) dyds<br />
0 O<br />
� t �<br />
+ G(x, y, t − s)σ(ũn(y, s)) W (dy, ds)<br />
0 O<br />
A. Millet (SAMM, Paris 1 and PMA) <strong>Cahn</strong>-<strong>Hilliard</strong>/<strong>Allen</strong>-<strong>Cahn</strong> <strong>equation</strong> Workshop <strong>Stochastic</strong> PDEs 16 / 18