On the Stochastic Cahn-Hilliard/Allen-Cahn equation - Isaac Newton ...

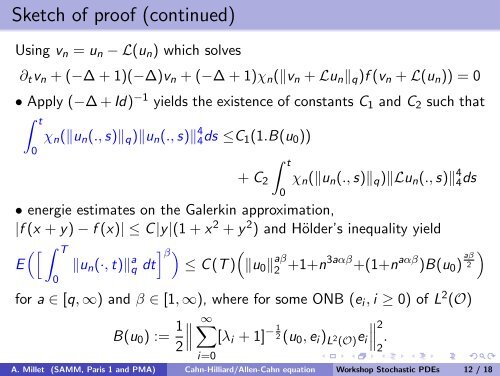
Sketch of proof (continued)
Using vn = un − L(un) which solves
∂tvn + (−∆ + 1)(−∆)vn + (−∆ + 1)χn(�vn + Lun�q)f (vn + L(un)) = 0
• Apply (−∆ + Id) −1 yields the existence of constants C1 and C2 such that
� t
0
χn(�un(., s)�q)�un(., s)� 4 4ds ≤C1(1.B(u0))
+ C2
� t
0
χn(�un(., s)�q)�Lun(., s)� 4 4ds
• energie estimates on the Galerkin approximation,
|f (x + y) − f (x)| ≤ C|y|(1 + x 2 + y 2 ) and Hölder’s inequality yield
��
E
� T
�un(·, t)� a �β� �
q dt ≤ C(T )
0
�u0� aβ
2 +1+n3aαβ +(1+n aαβ )B(u0) aβ
2
for a ∈ [q, ∞) and β ∈ [1, ∞), where for some ONB (ei, i ≥ 0) of L2 (O)
B(u0) := 1
� ∞�
�
�
1
− �
� [λi + 1] 2 (u0, ei) L2 (O)ei �
2
2
2 .
i=0
A. Millet (SAMM, Paris 1 and PMA) Cahn-Hilliard/Allen-Cahn equation Workshop Stochastic PDEs 12 / 18
�