unit 1 differential calculus - IGNOU

Remark
f ( 0 + h)
− f ( 0)
h − 0
Further = = 1 when h is positive.
h
h
f ( 0 + h)
− f ( 0)
∴ = 1
lim
+
h →0 h
f
( 0
+ h)
− f
lim
−
h →0 h
i.e. f ′ (0) does not exist.
− h − 0
= = − 1 when h is negative.
h
( 0)
= − 1
The above example will help you to conclude that a function can never have
derivative at a point of discontinuity. In the light of this remark, look at the
following example.
Example 1.23
Solution
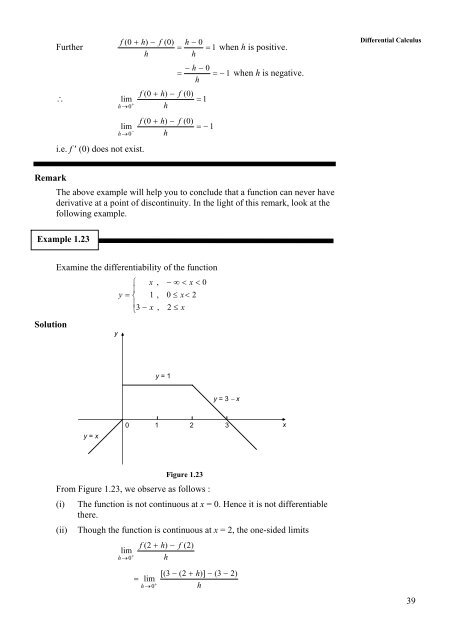
Examine the differentiability of the function
y = x
y
⎧ x ,
⎪
y = ⎨ 1 ,
⎪
⎩3
− x ,
y = 1
− ∞ < x < 0
0 ≤ x<
2
2 ≤ x
y = 3 − x
0 1 2 3
Figure 1.23
From Figure 1.23, we observe as follows :
(i) The function is not continuous at x = 0. Hence it is not differentiable
there.
(ii) Though the function is continuous at x = 2, the one-sided limits
lim
+
h →0
=
f
( 2 + h)
− f ( 2)
h
lim
+
h →0
[( 3
− ( 2 + h)
] − ( 3 −
h
2)
x
Differential Calculus
39