unit 1 differential calculus - IGNOU

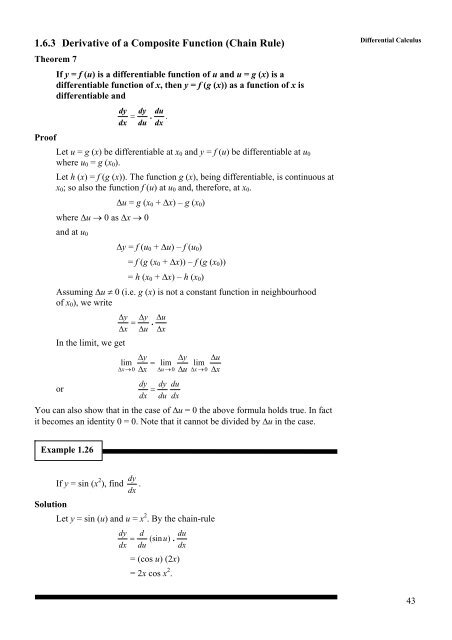
1.6.3 Derivative of a Composite Function (Chain Rule)
Theorem 7
Proof
If y = f (u) is a differentiable function of u and u = g (x) is a
differentiable function of x, then y = f (g (x)) as a function of x is
differentiable and
dy
dx
dy du
= . .
du dx
Let u = g (x) be differentiable at x0 and y = f (u) be differentiable at u0
where u0 = g (x0).
Let h (x) = f (g (x)). The function g (x), being differentiable, is continuous at
x0; so also the function f (u) at u0 and, therefore, at x0.
where Δu → 0 as Δx → 0
and at u0
Δu = g (x0 + Δx) – g (x0)
Δy = f (u0 + Δu) – f (u0)
= f (g (x0 + Δx)) – f (g (x0))
= h (x0 + Δx) – h (x0)
Assuming Δu ≠ 0 (i.e. g (x) is not a constant function in neighbourhood
of x0), we write
In the limit, we get
or
Δy
Δy
Δu
= .
Δx
Δu
Δx
Δy
Δy
Δu
lim = lim lim
x →0
Δx
Δu
→0
Δu
Δx
→ Δx
Δ 0
dy
=
dx
dy
du
du
dx
You can also show that in the case of Δu = 0 the above formula holds true. In fact
it becomes an identity 0 = 0. Note that it cannot be divided by Δu in the case.
Example 1.26
Solution
If y = sin (x 2 dy
), find .
dx
Let y = sin (u) and u = x 2 . By the chain-rule
dy
dx
=
d
du
(sin u)
.
= (cos u) (2x)
= 2x cos x 2 .
du
dx
Differential Calculus
43