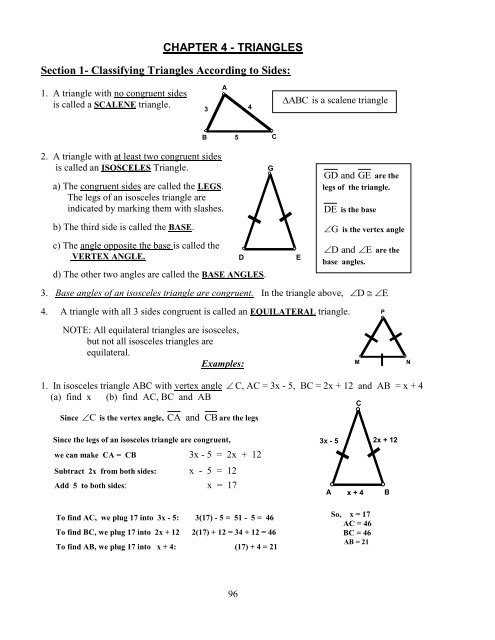
CHAPTER 4 - TRIANGLES Section 1- Classifying ... - Willets Geometry

CHAPTER 4 - TRIANGLES 

Section 1- Classifying Triangles According to Sides: 

1. A triangle with no congruent sides 

is called a SCALENE triangle. 

2. A triangle with at least two congruent sides 

is called an ISOSCELES Triangle. 

a) The congruent sides are called the LEGS. 

The legs of an isosceles triangle are 

indicated by marking them with slashes. 

b) The third side is called the BASE. 

c) The angle opposite the base is called the 

VERTEX ANGLE. 

d) The other two angles are called the BASE ANGLES. 

3 

B 

A 

5 

96 

4 

G 

C 

D E 

3. Base angles of an isosceles triangle are congruent. In the triangle above, D E 

4. A triangle with all 3 sides congruent is called an EQUILATERAL triangle. 

NOTE: All equilateral triangles are isosceles, 

but not all isosceles triangles are 

equilateral. 

Examples: 

P 

M N 

1. In isosceles triangle ABC with vertex angle C, AC = 3x - 5, BC = 2x + 12 and AB = x + 4 

(a) find x (b) find AC, BC and AB 

Since C 

is the vertex angle, CA and CB are the legs 

Since the legs of an isosceles triangle are congruent, 

we can make CA = CB 3x - 5 = 2x + 12 

Subtract 2x from both sides: x - 5 = 12 

Add 5 to both sides: x = 17 

To find AC, we plug 17 into 3x - 5: 3(17) - 5 = 51 - 5 = 46 

To find BC, we plug 17 into 2x + 12 2(17) + 12 = 34 + 12 = 46 

To find AB, we plug 17 into x + 4: (17) + 4 = 21 

ABC is a scalene triangle 

GD and GE are the 

legs of the triangle. 

DE is the base 

G is the vertex angle 

D and E 

are the 

base angles. 

3x - 5 

C 

2x + 12 

A x + 4 B 

So, x = 17 

AC = 46 

BC = 46 

AB = 21

2. In isosceles triangle PQR, with legs PQ and QR , 

mP 4x 5, mQ 3x 5 and mR 6x - 25 

(a) find x (b) find mP, mQ and m R 

Since PQ and QR are the legs of isosceles PQR , 

Pand R are the base angles. 

Since base angles of an isosceles triangle are congruent, 

mP m R 

: 4x + 5 = 6x - 25 

Subtract 4x from both sides: 5 = 2x - 25 

Add 25 to both sides: 30 = 2x 

Divide by 2: 15 = x 

To find mP, plug 15 into 4x + 5: 4(15) + 5 = 60 + 5 = 65 

To find m R plug 15 into 6x - 25: 6(15) - 25 = 90 - 25 = 65 

To find m Q, plug 15 into 3x + 5: 3(15) + 5 = 45 + 5 = 50 

3. Given isosceles triangle ABC with base AB . 

AC = 4x + 2, BC = 2x + 26 and AB = 3x + 14 

(a) find x (b) find AC, BC and AB 

Since AB is the base, AC and BC are the legs. 

Since the legs of an isosceles triangle are congruent, 

AC = BC: 4x + 2 = 2x + 26 

Subtract 2x from both sides: 2x + 2 = 26 

Subtract 2 from both sides: 2x = 24 

Divide by 2: x = 12 

To find AC, plug 12 into 4x + 2: 4(12) + 2 = 48 + 2 = 50 

97 

A 

P 

4x + 2 

To find BC, plug 12 into 2x + 26: 2(12) + 26 = 24 + 26 = 50 

To find AB, plug 12 into 3x + 14: 3(12) + 14 = 36 + 14 = 50 

Note: Since all three sides are equal to 50, then we now know 

that the triangle is an equilateral triangle. 

Q 

3x + 5 

4x + 5 6x - 25 

C 

3x + 14 

So, x = 15 

mP 65 

mR 65 

mQ 50 

2x + 26 

B 

So, x = 12 

AC = 50 

BC = 50 

AB = 50 

R

4. Given a triangle whose vertices are D(-1, 2), E(3, 1) and F(4, -3). Use the distance formula 

to determine whether the triangle is scalene, isosceles or equilateral. 

Recall : Distance x y 

Assignment: Section 1 

1. A triangle that has no sides congruent is called a (an) _______________ triangle. 

2. A triangle that has at least two congruent sides is called a (an) ________________ triangle. 

3. A triangle that has three congruent sides is called a (an) __________________ triangle. 

4. Given ∆ABC as marked: 

D 

2 2 

 

DE 

E 

 

 

3 4 1 3 

a) ∆ ABC is a (an) ___________________ triangle. 

b) ACand BC are called _________________ . 

c) AB is called the _________________ . 

d) AandBare called __________________ . 

e) C is called the ____________________ . 

F 

3 4 1 3 

14 2 2 

EF 

2 2 

 

2 2 

 

 

116 

17 

2 2 

98 

A 

 

1 3 2 1 1 3 2 1 

41 16 1 

17 

C 

2 2 

 

2 2 

 

2 2 

 

 

 

2 2 

 

 

1 4 2 3 

1 4 2 3 

55 2 2 

DF 

2 2 

 

25 25 

50 

2 2 

 

 

2 2 

Since DE EF , two sides of the triangle are congruent and the triangle is an isosceles triangle. 

 

B

5. A triangle with no congruent sides is called (a) isosceles (b) scalene (c) equilateral. 

6. An equilateral triangle has 

(a) no congruent sides (b) two congruent sides (c) three congruent sides. 

7. Draw isosceles triangle ABC with legs AB and BC. Mark the diagram. 

8. Draw equilateral triangle ABC. Mark the diagram. 

9. Isosceles triangle PQR has legs PQ and PR . Which of the following is true? 

(a) mP = mQ (b) mP = mR (c) mQ = m R 

10. Isosceles triangle XYZ has base angles X and Y . Which of the following is true? 

(a) XY = XZ (b) XY = YZ (c) XZ = YZ 

11. (TF) The base angle of an isosceles triangle are congruent. 

12. (TF) An equilateral triangle is always isosceles. 

13. (TF) An isosceles triangle is always equilateral. 

14. Given isosceles triangle ABC with legs AB and AC. 

AB = 3x + 5, AC = 2x + 17 and BC = x + 12. 

(a) Find x (b) find AB, AC and BC. 

15. Given isosceles triangle PQR with base angles P and R . 

PQ = 2x + 7, PR = 3x + 2 and QR = 5x - 2. (a) find x 

(b) which of the following is true? (1) PQ > QR (2) PQ > PR (3) PQ < PR 

16. Given isosceles triangle ABC with vertex B. 

mA = 23x - 11, mB = 50x + 10 and m C = x + 33. 

(a) find x (b) find mA, mB and m C 

17. Given isosceles triangle DEF with legs DE and DF . 

DE = 5x - 3, DF = 2x + 12 and EF = 4x + 2. 

(a) find x (b) find DE, DF and EF 

(c) which of the following is not true? 

(1) mE = m F (2) ∆ DEF is equilateral (3) FE > DE 

18. Given a triangle whose vertices are A(2, -4), B(5, 0) and C(1, 3). 

Use the distance formula to determine whether the triangle is scalene, isosceles or equilateral. 

19. Given a triangle whose vertices are A(-1, -2), B(-3, 2) and C(4, 1). 


20. Given a triangle whose vertices are A(3, 4), B(-1, 2) and C(4, -2). 


21. Given isosceles triangle ABC with legs AB and BC. 

AB = 4x - 7, BC = 2x + 5 and AC = 3x - 2 

(a) find x (b) find AB, BC and AC 

99

22. Given isosceles triangle XYZ with base angles X and Y . 

XZ = 5x - 2, XY = 3x + 1 and YZ = 2x + 1 

(a) find x (b) which of the following is true? (1) XZ >XY (2) XZ < XY (3) XZ = XY 

23. Given isosceles triangle PQR with vertex angle Q. 

mP = 7x + 1, mR = 5x + 7 and m Q = 50x - 14. 

(a) find x (b) find mP, mR, and m Q 

24. In isosceles triangle ABC, AB and BC are the legs. 

AB = 5x, BC = 3x + 2 and AC = 4x + 2. Is ∆ABC an equilateral triangle? 

25. Given a triangle whose vertices are A(-1, 0), B(4, 1) and C(2, -2). 


26. Given a triangle whose vertices are A(-3, 1), B(0, -3) and C(3, -2). 


Section 2 - Classifying Triangles According to Angles 

1. A triangle is an ACUTE TRIANGLE if all three of its angles contain less than 90°. 

2. A triangle is an EQUIANGULAR TRIANGLE if all three of its angles are congruent. 

Note: An equiangular triangle is also equilateral. 

3. If one of the angles of a triangle contains more than 90°, then the triangle 

is an OBTUSE TRIANGLE. The other two angles are acute angles. 

F 

H 

80° 

60° 40° 

FHG is an acute 

triangle. 

Each angle contains 

less than 90 

The sides which form 

the right angle are 

called the LEGS. 

The side opposite the 

right angle is called the 

HYPOTENUSE. 

G 

J 

50° 

100° 30° 

K L 

JKL is an obtuse 

triangle. 

K is greater than 

90 and the other two 

100 

P 

60° 

60° 60° 

M N 

PMN is 

equiangular. 

All three angles have 

the same measure. 

triangle. angles triangle. are acute. 

equiangular. 

4. If one angle of a triangle is a right angle, then the triangle is RIGHT TRIANGLE. 

The other two angles are acute. 

ABC is a right triangle. 

A 

B 

C 

B contains 90 and the other 

two angles are acute. 

AB and BC are the legs. 

AC is the hypotenuse.

Example: 

1. The coordinates of the vertices of ABC are A(3, -2) B(4, 1) and C(1, 2) 

(a) Use the slope formula to find the slope of each of the three sides of the triangle 

(b) Are any of the sides perpendicular? 

(c) What can you conclude about ∆ABC? 

(a) 

(b) 

3 1 

Slope of AB and Slope of BC . 

1 3 

Since AB is increasing (slope is positive) and BC is decreasing (slope is 

negative) 

and since their slope fractions are reciprocals, AB BC . 

(c) Since AB BC , then ABC is a right angle and ABC is a right triangle. 

AC would be the hypotenuse. 


1. A triangle whose angles each contain less than 90° is called a(an)_____________triangle. 

2. A triangle which contains one angle greater than 90° is called a(an) __________ triangle. 

3. A triangle which contains a 90° angle is called a(an) _______________triangle. 

4. If all the angles of a triangle are congruent, then the triangle is called 

a(an) _________________ triangle. 

5. In a right triangle, the side opposite the right angle is called the ________________ . 

6. In right triangle ABC, 

 

 

 

 

y 

Slope of AB 

x 2 1 2 1 3 

3 

 

3 4 3 4 1 

1 

 

 

 

 

y 

Slope of AC 

x 2 2 2 2 4 2 

 

3 1 3 121 

a) AB and ACare called ____________ 

b) BC is called the ________________ 

B 

A 

101 

 

 

 

 

y 

Slope of BC 

x 1 2 121 

4 1 4 13 

C 

C 

A 

B

7. (TF) A triangle which is equiangular is also equilateral. 

8. In ∆ ABC , m A = 40°, m B = 6° and m C = 134° . ∆ ABC must be 

(a) an acute triangle (b) an obtuse triangle (c) a right triangle 

9. In ∆ XYZ, m X = 52°, m Y = 43° and m Z = 85°. ∆ XYZ must be 


10. In ∆PQR, m P = 42°, m Q = 43° and m R = 90° . ∆PQR must be 


11. ∆ ABC is a right triangle with the right angle at A. 

(a) Draw ∆ ABC 

(b) The hypotenuse of the triangle is ______. 

(c) The legs of the triangle are ______ and ______. 

12. In ∆ABC , m A = 60°, m B = 60° and m C = 60°. 

Which of the following is not true? 

(a) ∆ABC is an acute triangle (b) ∆ABC is equilateral 

(c) ∆ABC is scalene (d) ∆ABC is equiangular 

13. The coordinates of the vertices of ABC are A(6, -5) B(0, 4) and C(3, -7) 


(b) Are any of the sides perpendicular? Why? 

(c) Is ∆ABC a right triangle? If so, which side is the hypotenuse? 

14. The coordinates of the vertices of ABC are A(2, 0) B(4, -1) and C(2, -1) 




15. The coordinates of the vertices of ABC are A(2, -1) B(3, 5) and C(-3, 6) 




16. The coordinates of the vertices of ABC are A(2, -3) B(4, 0) and C(-2, 5) 




17. Which of the following is true? 

(a) the base angles of an isosceles triangle may be obtuse. 

(b) the angles of an equilateral triangle may be obtuse. 

(c) the vertex angle of are isosceles triangle may be obtuse. 

18. Which of the following is not true? 

(a) A right triangle may be isosceles. 

(b) A right triangle may be scalene. 

(c) A right triangle may be equilateral. 

102

19. An equiangular triangle may not be 

(a) acute (b) equilateral (c) isosceles (d) obtuse 

20. Which of the following is true? 

(a) the base angles of an isosceles triangle may be right angles. 

(b) the base angles of an isosceles triangle may be obtuse angles. 

(c) the base angles of an isosceles triangle must be acute angles. 

Section 3 - Sum of the Interior Angles of a Triangle 

1. The sum of the interior angles of any triangle is always 180°. 

Example: 

The angles of ∆ABC are represented by 50x - 14, 5x + 7 and 7x + 1. 

(a) find x (b) find each angle of the triangle 

A 

(c) Which of the following statements is not true? 

(i) ∆ABC is isosceles 

7x + 1 

(ii) ∆ABC is an obtuse triangle 

(iii) ∆ABC is an acute triangle 

Since the angles were given in no particular order, it doesn't 

matter where we put them in the diagram. 

Since the angles of any triangle add up to 180‚ 

we can write the equation: 50x - 14 + 5x + 7 +7x + 1 = 180 

Combine like terms: 62x - 6 = 180 

Add 6 to both sides: 62x = 186 

Divide both sides by 62: x = 3 

To find mAplug x = 3 into 7x + 1: 7(3) + 1 = 21 + 1 = 22 

To find m B, plug x = 3 into 50x - 14: 50(3) - 14 = 150 - 14 = 136 

To find m C, 

plug x = 3 into 5x + 7: 5(3) + 7 = 15 + 7 = 22 

Therefore, the angles of the triangle contain 22°, 136° and 22°. 


1. The angles of ∆ABC are represented by 2x, x + 10 and 2x - 30. 


(c) Which of the following is true? 

(i) ∆ ABC is a right triangle 

(ii) ∆ ABC is an isosceles triangle 

(iii) ∆ABC is an obtuse triangle 

103 

B 

50x - 14 

Since two of the angles are congruent, we know that the triangle is isosceles. 

Since one angle contains more than 90 , we know that the triangle is obtuse. 

Therefore, in part (c) above, choice (iii) is NOT TRUE. 

5x + 7 

C

2. The angles of ∆ABC are represented by x + 35, 2x + 10 and 3x - 15. 



(i) ∆ ABC is a right triangle 

(ii) ∆ ABC is an equilateral triangle 

(iii) ∆ABC is a scalene triangle 

3. The angles of ∆ABC are represented by x + 2, 3x + 16 and 7x - 36, 



(1) ∆ ABC is a right triangle 

(2) ∆ ABC is an isosceles triangle 

(3) ∆ABC is an equiangular triangle 

4. The angles of ∆ABC are represented by 3x + 18, 4x + 9 and 10x. 

(a) Find x (b) Find each angle of the triangle. 

(c) Which of the following is not true? 

(1) ∆ABC is a right triangle 

(2) ∆ABC is an isosceles triangle 

(3) ∆ABC is equilateral 

5. The angles of a triangle are represented by 4x + 2, 5x - 15 and 2x + 6 

(a)Find x (b) Find each angle of the triangle. 


(1) the triangle is a right triangle 

(2) the triangle is an isosceles triangle 

(3) the triangle is an obtuse triangle 

6. The angles of ∆ABC are represented by x, 11x - 4 and 3x +4. 



(1) ∆ABC is an isosceles right triangle 

(2) ∆ABC is an obtuse triangle 

(3) ∆ABC is equilateral 

7. The angles of ∆ABC are represented by 4x, 3x + 7 and 4x +8. 




(2) ∆ABC is an equilateral triangle 

(3) ∆ABC is an acute triangle 

8. The angles of ∆ABC are represented by x +3, 7x - 9 and 8x - 6. 

(a)Find x (b) Find each angle of the triangle. 

(c) Which of the following is true 

(1) ∆ABC is a right triangle 


(3) ∆ABC is an acute triangle 

104

9. The angles of a triangle are represented by 6x +3, 5x +10 , and 11x+13. 


(c) Which of the following is not true? 

(1) The triangle is a isosceles triangle. 

(2) The triangle is a right triangle. 

(3) The triangle is an obtuse triangle 

10. The angles of a triangle are represented by 2x - 4, x + 28, and 3x-36. 



(1) the triangle is a right triangle 

(2) the triangle is an obtuse triangle 

(3) the triangle is an equilateral triangle 

Section 4 - Corollaries of the Triangle Sum Theorem: 

1. A COROLLARY is a theorem that follows easily from a previous theorem. Once we know 

that the sum of the angles of any triangle is 180°, it will be easy to convince ourselves that 

following corollaries are also true. 

2. If two angles of one triangle are 

congruent to two angles of a second 

triangle, then the third angles 

must be congruent also. 

3. A triangle may contain 

no more than one right angle 

or one obtuse angle. 

4. The acute angles of a 

right triangle are 

complementary. 

5. Each angle of an 

equilateral triangle 

contains 60° 

A 

A 

105 

? 

C 

60 80 

B 

D 

E 

? 

60 80 

A D and B F, then C E 

since both C and E would contain 40° 

With two right angles or two obtuse angles, 

the sides of the triangle won't meet 

and the sum of the angles of the "triangle" 

would add up to more than 180°! 

C B 

F 

60° 

60° 60° 

D E 

 

Since the three angles of the triangle must 

add up to 180 , 

Aand B must add up to 90 . 

So, Aand B must be complementary. 

Since the triangle is equilateral, it is also 

equiangular. 

If we divide the 180 evenly among the three angles, 

each angle will contain 60 

F

6. Each angle of an 

isosceles right triangle 

contains 45° 

1. 

2. 

Examples: 

Is ∆ABC an acute triangle, an obtuse triangle or a right triangle? 

A 

14° 

C 

65° 

? 

3. Find the vertex angle of an isosceles triangle if the base angles each contain 50° 

50° 50° 

N P 

4. The vertex angle of an isosceles triangle contains 68°. 

Find the measure of each of the base angles. 

R 

68° 

R 

? 

? ? 

N P 

K 

B 

45° 

A 

H 

35° 

45° 

In ABC, m A = 35° and m B = 82° 

Find the number of degrees in C. 

B 

82° 

In ABC, m A = 14° and m C = 65° 

106 

G 

? 

C 

Since the vertex angle contains 90 , we 

subtract 90 from 180 and split the result 

evenly between the two base angles. 

First we must find m B by adding 14 and 65 

and then subtracting from 180. 

14 + 65 = 79 

180 - 79 = 101 

Each base angle would contain 45 

To find mC, add 35 and 82 and then 

subtract the result from 180. 

35 + 82 = 117 

180 - 117 = 63 

181 

So, mC 63 

So, B contains 101 and so ABC is an 

obtuse triangle. 

We find m R by adding the two base angles and 

then subtracting from 180: 

50 + 50 = 100 

180 - 100 = 80 

So, mR 80 

First, we subtract 68 from 180 and then we split the result evenly 

between the two base angles. 

180 - 68 = 112 

112 2 56 

So, each base angle contains 56

5. One acute angle of a right triangle contains 37°. Find the other acute angle. 

U 

V 

? 

37° 

W 

Since the acute angles of a right triangle are 

complementary, we subtract 37 from 90 to 

obtain the other acute angle. 


1. Which of the following may not represent the three angles of a triangle? 

(a) 100°, 45°, 35° (b) 26°, 82°, 72° (c) 35°, 125°, 30° 

2. In ∆ABC, m A = 65° and m B = 72°. Find m C. 

3. For each of the following, two angles of a triangle are given. Find the third angle. 

(a) 59° and 63° (b) 42° and 108° (c) 121° and 50° (d) 62° and 58° 

4. Find the number of degrees in each angle of an equilateral triangle. 

5. Find the number of degrees in each acute angle of an isosceles right triangle. 

6. In ∆PQR, m P = 30° and m Q = 60°. ∆PQR is a(an) 

(a) acute triangle (b) obtuse triangle (c) right triangle 

7. In ∆XYZ, m X = 56° and m Y = 32°. ∆XYZ is a(an) 


8. In ∆ABC, m A = 84° and m B = 15°. ∆ABC is a(an) 


9. The sum of the interior angles of any triangle is ___________ degrees. 

10. In an equilateral triangle, each angle contains _________ degrees. 

11. In an isosceles right triangle, the acute angles each contain _________ degrees. 

12. (TF) If two angles of one triangle are congruent to two angles of a second triangle, then the 

third angles must also be congruent. 

13. (TF) The acute angles of any right triangle are supplementary. 

14. (TF) A triangle may contain more than one right angle. 

15. (TF) Each angle of an equilateral triangle contains 45°. 

16. If two angles of a triangle are 40° and 60°, then the third angle of the triangle contains 

(a) 40° (b) 100° (c) 80° 

17. The number of degrees in each angle of an equilateral triangle is (a) 45° (b) 60° (c) 90° 

107 

90 - 37 = 53 

So the other acute angle contains 53

18. The acute angles of a right triangle are (a) congruent (b) complementary (c) supplementary 

19. In ∆ABC, m A = 50° and m B = 40°. Then ∆ABC is a(an) 


20. In ∆XYZ, m X = 30° and m Y = 40°. Then ∆XYZ is a(an) 


21. In ∆ABC, m A = 80° and m B = 20°. Which of the following is not true? 

(a) ∆ABC is an acute triangle 

(b) ∆ABC is an isosceles triangle 

(c) ∆ABC is an obtuse triangle 

22. Which of the following is not always true? 

(a) the acute angles of a right triangle are complementary 

(b) the acute angles of a right triangle are congruent 

(c) the acute angles of an isosceles right triangle are congruent 

23. Find the vertex angle of an isosceles triangle if the base angles each contain 80°. 

24. One acute angle of a right triangle contains 28°. Find the other acute angle. 

25. The vertex angle of an isosceles triangle contains 72°. Find the measure of each base angle. 

26. Given isosceles triangle ABC with legs AB and BC . 

27. In isosceles triangle ABC with legs AB and BC , m B = 65°. Find m A. 

28. Find the vertex angle of an isosceles triangle if the base angles each contain 46°. 

29. The vertex angle of an isosceles triangle contains 120°. Find the measure of each base angle. 

30. Given right ∆ABC with hypotenuse AB . If m A = 63°, find m B. 

31. Given isosceles ∆XYZ with legs XY and YZ. If m Y = 48°, find m X and m Z. 

32. In isosceles ∆ABC with legs AB and AC, m A = 46°. 

Find the number of degrees in B . 

33. 

m A = 70°. (a) Find m C (b) find m B 

m1 _______ m5 ________ 

m2 _______ m6 ________ 

m3 _______ m7 ________ 

m4 ________ 

3 

108 

2 1 

4 

42 

78 

5 

7 6

34. AD BC 

35. 

m1 ______ m2 ______ 

m3 ______ m4 ______ 

Which of the following is false? 

(1) ∆AEF is an isosceles triangle 

(2) ∆AEF is a right triangle 

(3) ∆AEF is a scalene triangle 

m1 ______ m2 ______ 

m3 ______ m4 ______ 

36. Dark arrows indicate parallel lines. 

m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

37. In the diagram, a b 

m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 


m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

1 

8 

B 

120° 

3 

110° 

3 

3 

5 

60° 

109 

1 2 

E 

110° 

8 

2 

5 

8 

1 

45° 

9 

2 

9 

6 

40° 

30° 

4 

1 2 

9 

6 

70° 

2 

3 

1 

7 

F 

7 

7 

4 

3 

25° 

6 

4 

4 

5 

G 

4 

a 

b 

C 

a 

b


m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

40. Each base angle of an isosceles triangle contains 50°. Find the number of degrees 

in the vertex angle. 

41. In isosceles ∆XYZ with legs XY and XZ, m Y = 70°. Which of the following is false? 

(a) m Z = 70° (b) m X = 70° (c) m X = 40° 

42. The vertex angle of an isosceles triangle contains 30°. Then each base angle contains 

(a) 30° (b) 75° (c) 150° 

43. In isosceles ∆ABC with legs AB and BC , m B = 42°. Then A contains 

(a) 42° (b) 138° (c) 69° 

44. One acute angle of a right triangle contains 42°. The other acute angle contains 

(a) 42° (b) 90° (c) 48° 

45. In right ∆DEF, with legs DF and EF , m D = 60°. Then m E is equal to 

(a) 30° (b) 60° (c) 90° 


m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 


m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

1 

4 

2 

5 

25° 

140° 

6 

3 

110 

9 

30° 

5 6 7 

7 

9 

8 

30° 70° 

4 

8 9 

110° 

5 6 7 

3 

1 

8 

3 

2 

1 

2 

4 

a 

b 

a 

b 

a 

b



Section 5 - Word Problems: 

Examples: 

1. Find the number of degrees in each angle of a triangle if the ratio of the angles is 4: 3: 2. 

A 

m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

m1 _____ m6 _____ 

m2 _____ m7 _____ 

m3 _____ m8 _____ 

m4 _____ m9 _____ 

m5 _____ 

4x 

C 

2x 

3x 

B 

Since the sum of the angles of any triangle is 180°, 

we write the equation: 4x + 2x + 3x = 180 

Combine like terms: 9x = 180 


To find m A, we plug x = 20 into 4x: 4(20) = 80 

To find m B, we plug x = 20 into 3x: 3(20) = 60 

To find m C we plug x = 20 into 2x: 2(20) = 40 

So, the angles of the triangle are 80°, 60° and 40° 

4 

5 

4 

111 

8 

9 

5 6 7 

27° 

8 

120° 

9 

6 7 

Let the angles of the triangle be represented by “4x”, “3x” and 

“2x”. 

75° 

3 

42° 

1 

3 

2 

1 

2 

a 

b 

a 

b

2. If one angle of a triangle is twice the smallest angle and the third angle of the triangle is 

three times the smallest angle, find the number of degrees in each angle of the triangle. 

A 

x 

C 

2x 

3x 

Then, “twice the Since smallest the sum angle” of the is angles represented of any by triangle “2x” and is 180°, 

“Three times the smallest angle” is represented by “3x”. 

we write the equation: x + 2x + 3x = 180 

B 

Combine like terms: 6x = 180 


To find m A we replace x with 30 

To find m B we plug x = 30 into 3x: 3(30) = 90 

To find m B we plug x = 30 into 2x: 2(30) = 60 


3. In a triangle, the second angle is 35 degrees more than the first angle and 

the third angle is 5 degrees less than the first angle. 

Find the number of degrees in each angle of the triangle. 

A 

C 

x - 5 

We let “x” represent the number of degrees in the first angle. 

Then “35 degrees more than the first angle” is “x + 35” 

and “5 degrees less than the first angle” is “x – 5” 

x x + 35 

Let “x” represent the number of degrees in the “smallest 

B 

angle”. 


we write the equation: x + x + 35 + x - 5 = 180 

Combine like terms: 3x + 30 = 180 



To find m A, we replace x with 50 

To find m B, we plug x = 50 into x + 35: 50 + 35 = 85 

To find mC, we plug x = 50 into x - 5: 50 - 5 = 45 


112

4. Two angles of a triangle are in the ratio 3 : 4. The third angle is 20 degrees more than the 

smaller of the first two angles. Find the number of degrees in each angle of the triangle. 

Since “Two angles are in the ratio 3 : 4”, we let “3x” and “4x” represent these angles. 

Then “20 more than the smaller of the first two angles” is “3x + 20” 

C 

3x + 20 

3x 4x 

A B 


we write the equation: 3x + 4x + 3 x + 20 = 180 




To find mAwe plug x = 16 into 3x: 3(16) = 48 

To find mB, we plug x = 16 into 4x: 4(16) = 64 

To find m C we plug x = 16 into 3x + 20: 3(16) + 20 = 48 + 20 = 68 


5. The vertex angle of an isosceles triangle exceeds three times a base angle by 5 degrees. 


x 

Let “x” represent a base angle of the triangle. 

Since the base angles of an isosceles triangle are congruent, then the other base 

angle will also be represented by “x”. 

Since the vertex angle “exceeds 3 times a base angle by 5”, we represent the 

vertex angle by “3x + 5” 

C 

3x + 5 

A B 

x 


we write the equation: 

113 

x + x + 3 x + 5 = 180 




To find mAandm B, we replace x with 35 

To find m C, we plug x = 35 into 3x + 5: 3(35) + 5 = 105 + 5 = 110 

So, the angles of the triangle are 35°, 35° and 110°

6. Each base angle of an isosceles triangle is 9 less than four times the vertex angle. 


7. Find the number of degrees in the acute angles of a right triangle if one is four times the other. 

A 

C 

4x 

A 

Let “x” represent the number of degrees in the vertex angle. 

Then, “9 less than 4 times the vertex angle” would be represented by “4x - 9” 

Since the base angles of an isosceles triangle are congruent, each base angle would be “4x - 9” 

x 

C 

x 

4x - 9 4x - 9 

B 

B 

We write the equation: x + 4x = 90 

Combine complementary, 

like terms: 5x = 90 


To find m B, we replace x with 18 


We write the equation: 4x - 9 + 4x - 9 + x = 180 

Combine like terms: 9x - 18 = 180 

Add 18 to both sides both sides: 9x = 198 


To find mAandm B, 

we plug x = 22 into 4x - 9: 4(22) - 9 = 88 - 9 = 79 

To find m C, 

we replace x with 22 

So the angles of the triangle are 22°, 79° and 79° 

We let “x” represent one of the acute angles of the right triangle. 

Then the other acute angle would be represented by “4x”. 

Since the acute angles of a right triangle are complementary, 

To find m C, , we plug x = 18 into 4x: 4(18) = 72 

So, the acute angles of the right triangle are 18° and 72° 


1. Find the number of degrees in each angle of a triangle if the ratio of the angles is 5 : 3 : 1. 


3. If one angle of a triangle is 5 times the smallest angle and the third angle is 9 times the 

smallest 

angle, find the number of degrees in each angle of the triangle. 

114

4. In a triangle, the second angles 47 degrees more than the first angle and the third angle is 23 

degrees less than the first angle. Find the number of degrees in each angle of the triangle. 

5. Two angles of a triangle are in the ratio 5:2. The third angle of the triangle is 60 degrees more 

than the larger of the two angles. Find the number of degrees in each angle of the triangle. 

6. The vertex angle of an isosceles triangle is three times as large as each base angle. 


7. The vertex angle of an isosceles triangle exceeds a base angle by 45 degrees. 


8. The vertex angle of an isosceles triangle is 20 degrees less than three times each base angle. 

Find the number of degrees in each angle of the triangles. 

9. Each base angle of the isosceles triangle is 7 times the vertex angle. 


10. The vertex angle of an isosceles triangle exceeds twice a base angle by 60 degrees. 


11. Find the number of degrees in the acute angles of a right triangle if one is twice the other. 


13. In a triangle, the second angle is 14 degrees more than the first and the third angle is 6 more 

than 3 times the first angle. Find the number of degrees in each angle of the triangle. 

14. Two angles of a triangle are in the ratio 2 : 3. The third angle is 5 degrees more than the 

smaller of the first two angles. Find the number of degrees in the angles of the triangle. 

15. The vertex angle of an isosceles triangle exceeds twice the base angle by 16 degrees. 

Find each angle of the triangle. 

16. Each base angle of an isosceles triangle is 8 degrees less than 3 times the vertex angle. 


17. Find the number of degrees in each acute angle of a right triangle if one is 5 degrees less 

than 4 times the other. 

Section 6 - Medians and Altitudes: 

1. A segment which joins the vertex 

of an angle of a triangle to the 

midpoint of the opposite side 

is called a MEDIAN. 

A median bisects the side 

to which it is drawn. 

B 

A 

M 

median 

115 

C 

If point M is the midpoint of BC ’ 

Then AM is the median to BC 

and BM = MC

2. Each triangle has three medians that can be drawn, one to the midpoint of each side 

of the triangle. The point where the three medians intersect is called the CENTROID of the 

triangle. The centroid is always in the interior of the triangle. 

3. The median to the base of an isosceles triangle is perpendicular to the base 

and bisects the vertex angle. 

A M B 

4. The medians to the legs of an isosceles triangle are congruent to each other. 

C 

5. A segment drawn from the vertex of an angle of a triangle perpendicular to the opposite side 

is called an ALTITUDE. 

E 

C 

E 

B 

P 

F 

6. Each triangle has three altitudes: 

C 

1 2 

F 

A D 

B 

D 

A D 

B 

The three altitudes 

of an acute triangle 

intersect in the 

interior of the triangle. 

C 

A 

P 

D 

M N 

A B 

C 

E 

C 

A 

F 

B 

Two of the three 116 altitudes 

of an obtuse triangle 

are outside the triangle. 

The three altitudes do not 

intersect. 

Medians AD, BE and CF 

intersect at point P. 

Point P is called the 

CENTROID of the triangle. 

Median CM is drawn to the 

base of isosceles ABC . 

CM AB and 1 2 

AN and BM are the medians 

to the legs of isosceles ABC . 

AN BM 

CD is Cthe 

altitude to side AB 

CD AB 

A 

D 

In a right triangle, 

two of the altitudes 

coincide with the 

legs of the right 

triangle 

B

7. The altitude to the base 

of an isosceles triangle 

bisects the base and 

bisects the vertex angle. 

The altitude to the base 

is the same segment 

as the median to the base. 

8. The altitudes to the legs 

of an isosceles triangle 

are congruent to each other. 

9. 

A 

A 

C 

1 2 

M 

C 

10. In an equilateral triangle, the three medians are congruent to each other, the three altitudes 

are congruent to each other, and the three angle bisectors are congruent to each other 

A 

C 

P N 

M 

A 

T 

B 

M, P and N are midpoints 

D 

M 

117 

B 

D E 

Altitude: BT AC 

B C 

Angle Bisector: ABD DBC 

A 

C 

B 

E F 

D 

B 

CM is the altitude to base AB 

A 

AM MB 

1 2 

AE and BD are the 

altitudes to the legs of 

isosceles ABC 

AE BD 

Median: BM bisects AC 

Point M is the midpoint of AC 

Medians Altitudes Angle Bisectors 

C 

X Y 

CM AN BP 

CD AF BE 

CZ AY BX 

Z 

B

11. In an equilateral 

triangle, 

the three medians, 

the three altitudes 

and the 

three angle bisectors 

all coincide. 


1. A segment drawn from the vertex of an angle of a triangle to the midpoint of the opposite side 

is 

called a ___________________. 

2. A segment drawn from the vertex of an angle of a triangle perpendicular to the opposite side is 

called a(an)__________________. . 

3. An altitude of a triangle is ____________ to the side to which it is drawn. 

4. A median of a triangle _______________ the side to which it is drawn. 

5. The point where the three medians of a triangle intersect is called the _______________. 

6. The medians of an obtuse triangle (a) intersect in the interior of the triangle 

(b) do not intersect (c) intersect at a point on the triangle. 

7. The medians of a right triangle (a) intersect in the interior of a triangle 

(b) do not intersect (c) intersect at a point on the triangle 

8. The altitudes of an acute triangle (a) intersect in the interior of the triangle 


9. The altitudes of an obtuse triangle (a) intersect in the interior of the triangle 


10. The altitudes of a right triangle (a) intersect in the interior of the triangle 


11. (TF) The median to a side of a triangle bisects that side. 

12. (TF) The altitude to the base of an isosceles triangle is the same segment as the median drawn 

from the vertex angle to the base. 

13. (TF) The medians of an equilateral triangle are congruent. 

14. (TF) The medians of a right triangle intersect at the vertex of the right angle. 

15. (TF) The medians of an isosceles triangle are congruent. 

A 

16. (TF) The altitudes drawn from the base angles of an isosceles triangle are congruent. 

17. (TF) The altitude to the base of an isosceles triangle bisects the base. 

C 

M 

118 

B 

CM is the median to AB 

CM is the altitude to AB 

CM bisects 

ACB

18. (TF) The medians to the legs of an isosceles triangle are congruent. 

19. (TF) The median to the base of an isosceles triangle is perpendicular to the base. 

20. AB bisects the vertex angle of an isosceles triangle. Which of the following is NOT true? 

(a) AB is perpendicular to the base 

(b) AB is perpendicular to a leg 

(c) AB bisects the base 

21. If all three medians of a triangle are congruent to each other, then the triangle must be 

(a) right (b) isosceles (c) equilateral 

22. If the altitudes of a triangle intersect at a point on the triangle, then the triangle must be 

(a) acute (b) obtuse (c) right 

23. If an altitude, a median and the bisector of an angle of the triangle all coincide, 

then the triangle must be (a) acute (b) right (c) isosceles 

24. Which of the following is not true? 

(a) the bisector of the vertex angle of an isosceles triangle is the 

perpendicular bisector of the base 

(b)The altitudes to the legs of an isosceles triangle are congruent 

(c) Medians always intersect in the interior of the triangle 

(d) Altitudes always intersect in the interior of the triangle 

119

Section 7 - Congruent Triangles and CPCTC: 

1. Recall: Congruent segments have the same length 

and congruent angles have the same measure (same number of degrees). 

2. Two triangles are congruent if they can be made to coincide. 

A 

B 

C 

A’ 

3. Corresponding parts of two congruent triangles are congruent (CPCTC) 

A 

If two triangles are congruent, their corresponding sides are congruent and 

their corresponding angles are congruent 

B 

C 

A’ 

Examples: 

1. ABC A'B'C' AB = 5x - 2 , A'B' = 3x + 8 and B'C' = 4x + 1. 

(a) find x (b) find AB (c) find A'B' (d) find B'C'. 

A 

C 

5x - 2 B A’ 3x + 8 B’ 

C’ 

B’ 

4x + 1 

So, we write the equation: 5x - 2 = 3x + 8 

Subtract 3x from both sides: 2x - 2 = 8 



To find AB, plug x = 5 into 5x - 2: 5(5) - 2 = 25 - 2 = 23 

To find A'B', plug x = 5 into 3x + 8: 3(5) + 8 = 15 + 8 = 23 

To find B'C', plug x = 5 into 4x + 1: 4(5) + 1 = 20 + 1 = 21 

So, AB = 23, A’B’ = 23 and B’C’ = 21 

B’ 

120 

C’ 

C’ 

If we slide ABC to the 

right, it can be made to 

coincide with A'B'C' 

If ABC A'B'C' , then 

AB A 'B' A A 

' 

BC B'C' B B' 

AC A 'C' C C' 

By CPCTC, corresponding sides must be 

congruent so 

side AB must be congruent to side A'B'

A 

2. ABC A'B'C'. m A = 5x - 12, m B = 6x + 3 and m A' = 3x + 16. 

(a) find x (b) find m A (c) find m B (d) find m C 

C 

? 

5x - 12 6x + 3 3x + 16 

B A’ B’ 

So, we write the equation: 5x - 12 = 3x + 16 

Subtract 3x from both sides: 2x - 12 = 16 



To find m A, 

plug x = 14 into 5x - 12: 5(14) - 12 = 70 - 12 = 58 

To find m B, 

plug x = 14 into 6x + 3: 6(14) + 3 = 84 + 3 = 87 

To find m C, 

recall that the angles of a triangle must add up to 180°. 

We add 58 and 87 and then subtract from 180: 

58 + 87 = 145 and 180 - 143 = 35 


1. ABC A'B'C' . AB = 2x - 1 and A'B' = 5x - 13. 

(a) find x (b) find AB (c) find A'B' 

2. DEF D'E'F'. m D = 5x + 7 and m D' = 6x - 3. 

(a) find x (b) find m D (c) find m D' 

3. ABC A'B'C' . AB = 7x + 2, A'B' = 3x + 14 and BC = 2x + 1 

(a) find x (b) find AB (c) find A'B' (d) find BC (e) find B'C' 

4. ABC A'B'C' . m A = 6x - 2, m A' = 2x + 18 and m B = 20x. 

(a) find x (b) find m A (c) find m B (d) find m C (e) find m C' 

5. ABC A'B'C' . AB = 3x + 5 and A'B' = 7x - 11 

(a) find x (b) find AB (c) find A'B' 

C’ 

x 14, mA 58 , mB 87 and mC 35 

6. ABC A'B'C' . m A = 3x, m B = 5x + 10 and m A' = x + 20 


(f) Which of the following is true? 

(1) ∆ ABC and ∆A'B'C' are both acute triangles 

(2) ∆ ABC and ∆A'B'C' are both isosceles triangles 

(3) ∆ ABC and ∆A'B'C' are both right triangles 

121 

By CPCTC, corresponding 

angles must be congruent so 

A must be congruent to 

A'

7. ABC A'B'C' . AB = 2x + 3, A'B' = 7x - 12 and BC = x + 6. 

(a) find x (b) find AB and A'B' (c) find BC and B'C' 

(d) Are ∆ABC and ∆A'B'C' isosceles triangles? 

8. ABC A'B'C' . m A = 4x, m B = x + 45 and m A' = 3x + 15 


(f) Which of the following is not true? 

(1) ∆ ABC and ∆A'B'C' are both isosceles triangles 

(2) ∆ ABC and ∆A'B'C' are both equilateral triangles 

(3) ∆ ABC and ∆A'B'C' are both right triangles 

Section 8 - Congruency Theorems: 

1. SSS Congruency Theorem: (Side-Side-Side): Two triangles are congruent if the three sides 

of the first triangle are congruent to the corresponding sides of the second triangle. 

C 

A B 

C’ 

A’ B’ 

2. SAS Congruency Theorem: (Side - Angle - Side) Two triangles are congruent if two sides 

“SSS” Congruency Theorem 

and the included angle of the first triangle are congruent to two sides and the included angle 

of the second triangle. 

The "included angle" is the angle formed by the two sides. 

AC A'C', BC B'C', and AB A'B' . 

Congruent sides are marked with the same number of slashes. 

Since all three pairs of sides are marked, these two 

triangles are congruent by the 

The vertex of the angle is the common endpoint of the two sides. 

A A’ 

B C B’ C’ 

B is the angle included by AB and BC 

AB A'B', BC B'C' and B B' 

Since two pairs of corresponding sides are 

marked, and the angles included by these 

two sides are also marked, these two 

triangles are congruent by the 

“SAS” Congruency Theorem. 

3. ASA Congruency Theorem: (Angle - Side - Angle): Two triangles are congruent if two 

angles and the included side of the first triangle are congruent two angles and the included 

side of the second triangle. 

The "included side" is the segment formed by joining the vertices of the two angles. 

A 

B C 

A 

’ 

B’ C’ 

B B', C C' and BC B'C' 

Since two pairs of corresponding 

angles are marked and the sides 

included by these two angles are also 

marked, then these two triangles are 

congruent by the “ASA” Congruency 

122 

Theorem.

BC is the side included by B and C 

 

4. SAA Congruency Theorem: (Side - Angle – Angle): Two triangles are congruent if two 

angles and either non-included side of the first triangle are congruent to two angles and 

either non-included side of the second triangle. 

A 

B C 

5. HLR Congruency Theorem: (Hypotenuse - Leg - Right Angle): Two right triangles are 

congruent if the hypotenuse and one leg of the first right triangle are congruent to the 

hypotenuse and corresponding leg of the second right triangle. 

Examples: 

State which congruency theorem (SSS, SAS, ASA, SAA or HLR) is illustrated in each of the 

following. If no congruency theorem is illustrated, write NONE. 

1. 2. 

A 

right 

angle 

A’ 

B’ C’ 

A 

B 

B B', C C' and BA B'A' 

Since two pairs of corresponding 

angles are marked and a pair of sides 

not included by these two angles is 

also marked, then these two triangles 

are congruent by the “SAA” 

Congruency Theorem. 

C 

123 

A’ 

B’ C’ 

Hypotenuse AC Hypotenuse A'C', leg AB legA'B', 

and BandB'arerightangles Since the hypotenuse of right ABC is congruent to the hypotenuse of right A'B'C' 

and a pair of corresponding legs is also congruent, 

then these two triangles are congruent by the “HLR” Congruency Theorem. 

B 

leg 

hypotenuse 

C 

C’ 

“HLR” Congruency Theorem 

A’ 

B’ 

angle 

B 

A 

side 

angle 

C 

B’ 

A’ 

“ASA” Congruency Theorem 

C’

3. 4. 

angle 

5. 

C 

A angle B 

angle 

angle 

Here, in ∆ABE, only an angle 

and a side are marked. 

However, since 1 and 2 are 

vertical angles and since we know that 

vertical angles are congruent, 

we may mark these ourselves. 

Then, we will have the 

ASA Congruency Theorem 

124 

6. 


Here, in ∆ABD, only two sides are 

marked. However, since the two 

triangles share a common side, BD , 

we may mark this side with an "X" 

and we will now have SSS. 

Here, in ∆ABD, we have hypotenuse 

AD marked. 

We also have ABD is a right angle. 

That means that DBC is also a 

right angle and we can mark that. 

We also see that the triangles share a 

common side so we can mark that. 

Then we will have the HLR Congruency 

Theorem. 



1. 2. 3. 

C’ 

A’ B’ 

Since there is no "AAA" Congruency 

Theorem, we write "NONE" 

A 

B 

side 

1 

E 

2 

C 

D 

A 

A 

side 

hypotenuse 

side 

D 

D 

B 

B 

C 

C

4. 5. 6. 

7. 8. 9. 

10. 11. 12 

13 14 15 

In each of the following, mark the given information. You may also mark "common side" or 

vertical angles. You may not mark right angles unless they are given. When the diagram is 

marked, state which congruency theorem has been marked. 

If no congruency theorem is marked, write NONE. 

16. Q and T are right angles 

R is the midpoint of QT . PQ = WT 

P 

Q 

R 

W 

T 

17. P W 

R is the midpoint 

of QT 

125 

Q 

P 

R 

W 

T

18. H is the midpoint of FG 

IH FG 

20. 

PQ QT, 

WT QT 

PR WR 

F H 

G 

P 

Q 

I 

R 

19. JK NM, KL ML and JL NL 

21. R is the midpoint of QT 

R is the midpoint of PW 



22. 23. 24. 

25. 26. 27. 

28. 29. 30. 

31. 32. 33. 

T 

W 

P 

126 

J 

Q 

K 

R 

L 

M 

T 

N 

W

In each of the following, mark the given information. You may also mark "common side" or 

vertical angles. You may not mark right angles unless they are given. When the diagram is 

marked, state which congruency theorem has been marked. 

If no congruency theorem is marked, write NONE. 

34. 

36. PQ DE, PR DF 

37. 

R 

38. 

A D 

C is the midpoint of AD 

A 

B 

B is the midpoint of AC, 

C 

and RQ FE 

P 

Cis a right angle, Ais 

a right angle, 

E 

A 

Q 

F 

AE CD 

B 

E 

D 

D E 

D 

C 

35. A C, D E and AB BC 

127 

A 

A 

D 

B 

39. D is the midpoint of AB 

AC BC 

B 

BA AD, ED AD 

C is the midpoint of BE 

C 

A D 

B 

C 

E 

E 

C 

D

40. A is a right angle, 

C is a right angle, 

BE BD and AE CD 

E 

A 

42. C is the midpoint of BD and 

C is the midpoint of AE 

A 

1 

B 

3 

2 

128 

41. DB AC 

B is the midpoint of AC 

43. AD BC and 1 2 

44. DB ACandAD DC 

45. BC DF, AC EF and C F 

A 

A 

B 

B 

C 

Section 9 - Exterior Angles of a Triangle: 

1. If one side of a triangle is extended, a new angle is formed, with the extension as one side 

of the angle and the side of the triangle as the other side of the angle. 

This angle is called an 

EXTERIOR ANGLE. 

D 

C 

D 

D B 

B 

C 

E 

A 

A 

A 

C D 

D 

1 

D 

C 

B 

D 

2 

B 

C 

C 

E F 

When side AC is extended to 

point D, BCD is formed. 

BCD is an exterior angle 

with sides CD and CB

2. The angle of the triangle that is next to the exterior angle is called the 

ADJACENT INTERIOR ANGLE. 

The other two angles of the triangle are called the REMOTE INTERIOR ANGLES. 

A 

1 

3. The Exterior Angle Theorem: 

B 

3 

2 

C 

4 

4 is an exterior angle. 

2 is its adjacent interior angle. 

1 and 3 are its remote interior angles. 

(a) The sum of the measure of an exterior angle and its adjacent interior angle is 180°. 

(b) The measure of an exterior angle of a triangle is equal to the sum of the measures 

of its remote interior angles. 

Examples: 

1. In ∆ABC, mA = 52° and mB = 68°. Find the number of degrees in an exterior angle at C. 

A 

2. In ∆ABC, mABC = 72°. Find the number of degrees in an exterior angle at B. 

A 

52° 

B 

68° 

C 

72° 

B 

C 

x 

x 

m3 m4 180 

m4 m1 m 2 

A 

129 

1 

3 

Since we wish to find the number of degrees 

in the exterior angle, we call this "x": 

Since the measure of an exterior angle is 

equal to the sum of the measures of the 

remote interior angles, 

B 

2 

4 

C 

we simply add 52° and 68° 

and so x = 120°. 

We draw a triangle with an exterior angle. Label the vertex of this angle "B" 

Since we wish to find the number of degrees in the exterior angle, 

we call this "x": 

Since the sum of an exterior angle and its adjacent interior angle is 180°, 

we simply subtract 72 from 180, and so x = 108°.

3. In isosceles ∆ABC, with base BC , mB = 70°. 

Find the number of degrees in an exterior angle at C. 

B 

4. In isosceles triangle ABC with vertex angle C, mC = 104°. 

Find the number of degrees in an exterior angle at A. 

B 

70° 

A 

5. In ∆ABC, an exterior angle at A contains 107°. If m ABC = 50°, find m C. 

B 

C 

104° 

C 

50° 

x 

C 

x 


1. (a) 1 is called a(an)______________________angle 

(b) 2 is called a(an)_______________________angle 

(c) 3 and 4 are called ___________________angles 

(d) m 1 = m _______ + m _________ 

(e) m 1 + m 2 = _________ 

A 

x 

A 

We draw an isosceles triangle with an exterior angle. 

Label the vertex of this angle "C" and the base of the isosceles triangle as " BC " 

We draw an isosceles triangle with 

an exterior angle. Label the vertex 

of this angle "A" and the vertex 

angle of the isosceles triangle as C. 

107° 

Since the base angles of an isosceles triangle 

are congruent, then m ACB is also 70° 

Since the sum of an exterior angle and its 

adjacent interior angle is 180°, 

we simply subtract 70 from 180. So x = 110°. 

First, we must find the base angles of the triangle. 

So, we subtract 104 from 180 and then divide by 2 to 

split it evenly between the two base angles: 

180 - 104 = 76 and 76 2 = 38 

2. An exterior angle is equal to the sum of the measures of its _______________________. 

3. The sum of an exterior angle and its adjacent interior angle is _______ 

130 

And so, each base angle is 38° 

Since the sum of an exterior angle and its adjacent 

interior angle is 180°, 

we simply subtract 38 from 180. So x = 142°. 

Since the measure of an exterior angle is 

equal to the sum of the measures of the 

remote interior angles, 

we simply subtract 50 from 107 

and so x = 57°‚ 

4 

3 2 1

4. In ∆XYZ, m X = 22° and m Y = 57°. Find the number of degrees in an exterior angle at Z. 

5. In ∆XYZ, mYXZ = 110°. Find the number of degrees in an exterior angle at X. 

6. In isosceles ∆ABC, with vertex angle B, m A = 30°. 


7. In isosceles ∆ABC, with vertex angle B, m B = 22°. 


8. In ∆ABC, an exterior angle at B contains 59°. If m ACB = 22°, find m A. 

9. Find the value of “x” in each of the following: 

(a) 

(b) 

D 

x + 38 

2x - 9 

5x-35 

A B C 

(c) (d) 

D 

4x 

6x - 20 

8x + 10 

A B C 

10. In ∆ABC, m A = 28° and m B = 107°. 


11. In ∆ABC, m ABC = 125°. Find the number of degrees in an exterior angle at B. 

12. In isosceles triangle ABC, with base AB , m A = 62°. 

Find the number of degrees in an exterior angle at B. 

13. In ∆ABC, an exterior angle at B contains 120°. If m BAC = 75°, find m C. 

14. In ∆ABC, m B = 46° and m C = 25°. 


15. In isosceles triangle ABC, with vertex angle A, m B = 22°. 


16. In isosceles triangle ABC, with base BC , m A = 80°. 

Find the number of degrees in an exterior angle at B. 

17. In ∆ABC, an exterior angle at A contains 87°. If m ABC = 62°, find m C. 

131 

D 

5x - 5 

8x - 23 

A B C 

3x - 51 

D 

x + 23 

A B 

C

18. Find the value of “x” in each of the following: 

(a) 

D 

6x - 2 

4x - 6 

Section 10 - Triangle Inequalities: 

In ∆ABC, A is the 

smallest angle. 

Therefore, BC is 

the shortest side 

(b) (c) 

1. The hypotenuse is the longest side of a right triangle. 

2. In a triangle, the largest angle of the triangle is opposite the longest side of the triangle 

and the smallest angle is opposite the shortest side. 

A 

5 

B 

10 

7 

3. In a triangle, the longest side of the triangle is opposite the largest angle 

and the shortest side of the triangle is opposite the smallest angle. 

A 

8x + 4 

A B C 

In ∆ABC, side AC is the 

longest side. 

Therefore, B is 

the largest angle. 

B 

80° 

40° 60° 

C 

C 

3x - 50 

Hypotenuse DF is the longest side of right triangle DEF 

D 

x - 7 

132 

A 

A 

5 

3x - 15 

A B 

C 

B 

10 

D 

E 

7 

C 

In ∆ABC, side AB is the 

shortest side. 

Therefore, C is 

the smallest angle. 

B 

80° 

40° 60° 

In ∆ABC, 

B is the 

largest angle. 

Therefore, AC is 

the longest side 

C 

D 

2x - 16 

F 

3x + 21 

A B C

Step 1: 

Step 2: 

Step 3: 

A 

A 

A 

A 

B 

60° 

40° 

40° 

B 

80° 

40° 

80° 

60° 

70° 

30° 

C 

Examples: 

In the diagram, which segment 

is the shortest segment? 

40° 

B 

80° 

B 

80° 

80° 

60° 

80° 

60° 

70° 

30° 

C 

D 

70° 

60° 

50° 

1. Which side of the triangle is longer: 

BC or AB ? 

C 

70° 

D 

70° 

60° 

C 

D 

70° 

30° 

60° 

50° 

50° 

E 

E 

E 

133 

A 

40° 

B 

80° 

80° 

60° 

A 

70° 

30° 

At this point, all sides have been eliminated except BD . 

Therefore, BD is the shortest segment in the diagram 


C 

D 

70° 

60° 

B 

70° 

50° 

50° 

First, we consider ABC . 

Since we are looking for the shortest 

segment, we can eliminate the segments 

opposite the 80 angle and the 60 angle. 

We eliminate these segments by putting 

slashes through those two sides. 

Next, we consider BCD . 






Finally, we consider DEC . 






2. Which side of the triangle is longer: 

AC or BC ? 

E 

C

3. Which angle of the 

triangle is larger? 

Aor C 

A 

10 

B 

8 

6. Find m C 

Which side of the 

triangle is the 

shortest side? 

A 

50° 

B 

90° 

C 

C 


triangle is larger? 

Aor B 

A 

B 

12 

16 

7. Which angle of the triangle 

is the largest angle? 

9. In ∆ABC, AB = 12, BC = 15 and AC = 5. 

(a) which is the largest angle of the triangle? 

(b) which is the smallest angle of the triangle? 

7 

134 

C 

5. Find m B. 

Which side of the triangle 

is the longest side? 


is the smallest angle? 

10. In ∆XYZ, XY = 10, XZ = 11 and YZ = 18. What is the largest angle of the triangle? 

11. In ∆ABC, m A = 65° and m B = 25°. 

(a) Find m C (b) What is the longest side of the triangle? 

C 

12. In ∆XYZ, m X = 15° and m Y = 63°. What is the longest side of the triangle? 

13. In isosceles ∆ABC, with vertex A, AB = 11 and BC = 6. 

Which of the following is not true? (a) B C (b) B > A (c) B < A 

14. In isosceles ∆ABC, with base AB , m C = 58°. (a) Find m A and m B 

(b) Which of the following is true? (1) AB > BC (2) AB < AC (3) AC < BC 

15. In isosceles ∆ABC, with base BC , m B = 65°. Which of the following is true? 

(a) AB > AC 

(b) the base is the longest side of the triangle 

(c) the base is the shortest side of the triangle 

10 

A 12 B 

16. Given right triangle ABC with right angle at A. What is the longest side of the triangle? 

A 

B 

50° 

12 

C 

30° 

A 9 B 

8 

C

17. In the diagram, 

which segment 

is the shortest 

segment? 

A 

A 

80° 

B 

80° 

70° 

60° 

B 

40° 

A 

80° 

60° 

50° 

40° 

F 

C 

B 

30° 

90° 

C 

D 

80° 

19. In the diagram, which segment 


70° 

70° 60° 

70° 

21. Which side of the triangle 

is longer: BC or AB ? 

A 

B 

70° 

80° 

24. Find m C 


is the shortest side? 

C 

50° 

50° 

70° 

A 

30° 

E 

D 

C 

B 

135 

A 

6 7 


is the longest segment? 

C 

70° 

40° 


triangle is larger? Aor C 


triangle is larger: 

Aor B? 

B 

60° 

80° 

F 

75° 

50° 

60° 

35° 

C 

70° 

50° 

D 

50° 

80° 

23. Find m A. 


is the longest side? 


is the smallest angle? 

27. In ∆ABC, AB = 7, BC = 11 and AC = 15. Which is the largest angle of the triangle? 

28. In ∆ABC, m A = 105° and m B = 37°. 

(a) find m C (b) Which is the shortest side of the triangle? 

A 

B 

16 

18. In the diagram, 

which segment 

is the longest 

segment? 

B 

70 

40° 

D 

50 

° 

80° 

30 

° 60° 70 ° 

80° 60° ° 

A 

C 

14 

C 

A 

B 

18 

30° 

C 

70° 

12 

A 19 B 

E 

C 

E

29. In ∆XYZ, m X = 71° and m Y = 8°. What is the longest side of the triangle? 

30. In ∆PQR, m P = 90°. What is the longest side of the triangle? 

31. In isosceles ∆ABC, with vertex B , AB = 8 and AC = 11. 

Which of the following is not true? (a) A > B (b) C 

32. In isosceles ∆ABC, with base BC , m A = 72°. (a) find m B and m C 

(b) Which of the following is true? 

(1) AB < BC (2) AC > BC (3) AB > AC 

33. In isosceles ∆XYZ, with vertex X, m X = 70°. 

Which of the following is true? (a) XY > XZ 

(b) YZ is the longest side of the triangle 

(c) YZ is the shortest side of the triangle. 

34. (TF) The hypotenuse is always the longest side of a right triangle. 



A 

40° 

B 

90° 

80° 



A 

40° 

B 

80° 

80° 

60° 

50° 

30° 

70° 

60° 70° 

C 

A 

50° 

F 

70° 

30° 

D 

70° 

60° 

50° 

E 

D 

C 

136 


is the longest segment? 

A 

80° 

75° 

80° 

B 

40° 

B 

40° 

F 

80° 

60° 

30° 

60° 

60° 

75° 

30° 

70° 

50° 

70° 

C 

C 

D 

70° 

60° 



D 

80° 

50° 

50° 

50° 

E 

E

Section 11: Sum of Any Two Sides of a Triangle: 

1. The sum of the lengths of any two sides of a triangle must be greater than the length of the third side. 

3 

8 

A B 

10 

A B 

10 

6 

C 

C 

P 

D 

D 

A B 

E 

5 

6 

4 

To make a triangle whose sides are 6, 8 and 10, 

we bring segment AC and segment BD towards each other. 

They will intersect at point P, forming the “peak” of the triangle. 

If we try to make a triangle whose sides are 3, 5 and 10, 

we see that the lengths of AC and BD only add up to 8. 

When point C and point D are brought together to form the 

“peak” of the triangle, 

they will collapse onto segment AB and leave a gap. 

2. In general, given the lengths of two sides of a triangle, the third side must be 

less than the sum of the two given sides and greater than the positive difference 

between the two given sides. 

A 

12 

C 

a 

C 

10 

c 

D If we try to make a triangle whose sides are 6, 4 and 10, 

16 

B 

b 

we see that the lengths of AC and BD add up to exactly 10. 

When point C and point D are brought together to form the 

“peak” of the triangle, 

they will collapse onto segment AB at point E. 

(1) c < a + b 

(2) If b > a, then c > b - a 

Example: 

In ABC , the length of AB 

must be less than 12 + 16 = 28 

and greater than 16 - 12 = 4 

So AB can be any number between 4 and 28. 

137


1. Which of the following may represent the sides of a triangle? 

(a) 3, 6, 8 (b) 4, 7, 2 (c) 6, 11, 17 (d) 5, 8, 14 

2. Two sides of a triangle are 6 and 18. The third side of the triangle must be greater 

than ______ but less than ______. 



4. Two sides of a triangle are 15 and 28. Which of the following could be the length of 

the third side? (a) 7 (b) 30 (c) 50 



6. The perimeter of an isosceles triangle is 21. 

Draw all possible triangles where the lengths of the sides are integers. 



8. Which of the following may represent the sides of a triangle? 

(a) 10, 12, 25 (b) 13, 17, 4 (c) 14, 18, 22 (d) 8, 10, 1 













Section 12 - Types of Polygons: 

1. A figure with many sides is called a POLYGON. 

(a) A polygon with 3 sides is called a TRIANGLE. 

(b) A polygon with 4 sides is called a QUADRILATERAL. 

(c) A polygon with 5 sides is called a PENTAGON. 

(d) A polygon with 6 sides is called a HEXAGON. 

138

(e) A polygon with 7 sides is called a SEPTAGON. 

(f) A polygon with 8 sides is called an OCTAGON. 

(g) A polygon with 9 sides is called a NONAGON. 

(h) A polygon with 10 sides is called a DECAGON. 

(i) A polygon with "n" sides is called an "N-GON" 

2. An EQUILATERAL POLYGON is a polygon with all sides congruent. 

An EQUIANGULAR POLYGON is a polygon with all angles congruent. 

A REGULAR POLYGON is both equilateral and equiangular. 

3. A figure is said to be NON-CONVEX when points P and Q can be found within the figure 

such that the segment PQ has points which are not in the figure. If no points P and Q can be 

found, the figure is said to be CONVEX. 

(a) (b) (c) (d) (e) 

P Q 

Non-Convex 

Convex 

Non-Convex Convex Non-Convex 

Non-convex figures tend to have “holes” or “indentations” in them. 


1. Count the number of sides and then write the name of each polygon illustrated: 

(a) (b) (c) (d) 

C 

D 

E 

B 

C 

F D 

(e) (f) (g) (h) 

I 

H G F 

A 

B 

C 

E 

D 

2. A figure with many sides is called a _________________. 

3. A hexagon has (a) 5 sides (b) 6 sides (c) 7 sides 

A 

B 

F 

F 

E 

H E 

A 

G 

C 

D 

Q 

P 

D 

N 

O 

P 

Q 

A 

139 

E A 

B 

C 

C 

M 

D 

B 

L 

K 

J 

H 

G 

E F 

I 

C 

B 

A 

P Q 

C D E 

J 

I 

H 

G 

F 

A B

4. A polygon with 5 sides is called a _______________ 

5. A polygon with 4 sides is called a ___________________. 

6. Which of the following has 10 sides? (a) septagon (b) nonagon (c) decagon 

7. A polygon with all angles congruent is said to be _________________________ 

8. A septagon has _________ sides. 

9. A polygon that is both equilateral and equiangular is said to be a _______________polygon. 

10. Which of the following is a regular quadrilateral? 

D C 

(a) (b) 

A B 

11. A polygon with 9 sides is called a _______________. 

12. A polygon that has all sides congruent is said to be _____________________. 

13. Which of the following is non-convex? 

(a) (b) (c) 

14. Which of the following is convex? 

(a) (b) (c) 

Section 13 – Interior Angles of a Polygon: 

1. The sum of the measures of the interior angles of any triangle is always 180 . 

2. Let “n” represent the number of sides of any polygon. 

A polygon with “n” sides will also have “n” interior angles. 

A 

1 

B 

E 

2 

5 

3 

4 

C 

D 

ABCDE is a pentagon. 

The pentagon has 5 vertices: 

Points A, B, C, D, and E are vertices. 

The pentagon has 5 sides: 

AB, BC, CD, DE and EA are sides of the pentagon. 

The pentagon has 5 interior angles 

1, 2, 3, 4, and 5 are interior angles of the 

pentagon. 

140 

(c)

3. To find the sum of all interior angles of a polygon that has “n” sides and “n” interior angles, 

use the following formula: 

Sum of all interior angles = 180n 2 

Example #1: 

Find the sum of all interior angles of a septagon. 

Since a septagon has 7 interior angles, we let “n” = 7: 

We write the formula: Sum of all interior angles = 180n 2 

Replace “n” with “7” Sum of all interior angles = 1807 2 

Simplify “7 - 2” Sum of all interior angles = 180 5 Multiply 180 times 5: Sum of all interior angles = 900 

So, all 7 interior angles of a septagon will add up to 900 

Example #2: 

The sum of all interior angles of a polygon is 1620 . How many sides does the polygon 

have? 

We write the formula: Sum of all interior angles = 180n 2 

We replace “sum of all interior angles” 

with 1620: 1620 180n 2 

Distribute 180: 1620 180n 360 

Add “360” to both sides of the equation: 1980 = 180 n 

Divide both sides by “180”: 11 = n 

So the polygon would have 11 sides. 

141

4. If a polygon is a regular polygon (both equilateral and equiangular), we can find EACH 

interior angle of the polygon by dividing the sum of all the interior angles by the number of 

angles in the polygon. 

To find EACH interior angle of a regular polygon that has “n” sides and “n” interior 

use the following formula: 

180 n 2 

Each interior angle of a regular polygon = 

n 

Example #3: 

142 

 

Find the number of degrees in each interior angle of a regular polygon with 12 sides: 

 

 

180 n 2 

We write the formula: Each interior angle of a regular polygon = 

n 

 

180 12 2 

Replace “n” with 12: Each interior angle of a regular polygon = 

12 

 

18010 Subtract “12 – 2”: Each interior angle of a regular polygon = 

12 

Multiply 180 x 10: Each interior angle of a regular polygon = 1800 

12 

Divide 1800 by 12: Each interior angle of a regular polygon = 150 

So, each of the 12 interior angles of the polygon would contain 150 degrees. 

Example #4: 

Each interior angle of a regular polygon contains 162 degrees. 

How many sides does the polygon have? 

 

We write the formula: Each interior angle 

180n2 of a regular polygon = 

n 

Replace “Each interior angle 

180 n 2 

of a regular polygon” with 162 : 162 = 

n 

Re-write “162” as 162 

162 

1 

1 

 

180 n 2 

n 

162 n 180 n 2 

 

Cross-multiply: 

Distribute “180”: 162 n 180n 360 

Add “- 180n” to both sides: 18n 360 

Divide both sides by –18: n 

20

So the polygon would have 20 sides. 


1. Find the sum of all interior angles of a polygon with 15 sides. 




3. The sum of all interior angles of a polygon is 2520. How many sides does the polygon 

have? 


4. The sum of all interior angles of a polygon is 1440 . How many sides does the polygon 

have? 


5. Find the number of degrees in each interior angle of a regular polygon with 10 sides: 

180n2 Each interior angle of a regular polygon = 

n 



n 

7. Each interior angle of a regular polygon contains 160 degrees. 



n 



180 n 2 


n 

143 

 






have? 



have? 

Sum of all interior angles = 180n 2



n 



n 



180 n 2 


n 

144 

 



180 n 2 


n 

17. Find the sum of all interior angles of a quadrilateral. 

 

18. Find the number of degrees in each interior angle of a regular pentagon. 


have? 

20. Each interior angle of a regular polygon contains 108 degrees. How many sides does 

the polygon have? 


have? 

22. Find the sum of all interior angles of a decagon. 

23. Each interior angle of a regular polygon contains 150 degrees. How many sides 

does the polygon have? 

24. Find the number of degrees in each interior angle of a regular octagon.

Section 14 – Exterior Angles of a Polygon: 

1. If a side of a polygon is extended, the exterior angle formed is always supplementary to its 

adjacent interior angle. 

In the figure, side AB is extended to form an exterior angle 

F 

E 

1 is the exterior angle and 2 is its adjacent interior angle. 

f 

g 

a 

e 

Since 1 and 2 form a linear pair, 

then m1 m2 180 2. The sum of the exterior angles of a polygon (one per vertex) is always 360 . 

d 

A 

e 

c 

D 

2 1 

a 

b 

C 

B P 

a b c d e 360 a b c d e f g 360 

3. If a polygon is a regular polygon (both equilateral and equiangular), we can find EACH 

exterior angle by dividing 360 by the number of sides in the polygon. 

c 

40 

d 

80 

Each exterior angle of a regular polygon with “n” sides = 360 

n 

b 

150 

135 

a 

70 

b 

d 

145 

c 

Example #1 

Find the value of a, b, c and d 

Since a = 45 and b = 30 , then 

“a” is an exterior angle and its adjacent interior angle 

contains 135 . Since 135 a 180, then a = 45 

“b” is an exterior angle and its adjacent interior angle 

contains 150 . Since 150 b 180 , then b = 30 . 

There are six exterior angles in the diagram. 

All six exterior angles must add up to 360 . 

Since “c” and “d” are linear pairs, then “c” and “d” are supplementary so d 85. g 

f 

h 

i 

e 

a b c d e f g h i j 360 45 70 30 40 80 c 360 

j 

d 

c 

a 

b 

265 c 360 

c 95

Example #2 

The polygon in the diagram is a regular polygon. 

(a) Find the number of degrees in each exterior angle of the polygon. 

(b) Find the number of degrees in each interior angle of the polygon. 

(a) First, we count the number of congruent sides in the 

regular polygon and find that there are 8 sides. 

This means that there will be 8 congruent exterior angles. 

To find the number of degrees in each of the congruent 

exterior angles, we divide 360 8 45 

. 

Therefore, each of the 8 congruent exterior angles contains 45 

(b) Since each exterior angle is a linear pair with its adjacent interior angle, 

we subtract 180 45 135 Therefore, each of the eight congruent interior angles contains 135 

Assignment – Section 14 

1. The sum of the exterior angles of any polygon is always ______________ degrees. 

2. Find each of the angles in the diagram: 


20 

60 

r 

a 

b 

15 

z 

w x 

120 

15 

z y 

b 

a 

p 

22 

x 

y 

w 

25 

75 

40 

40 

x = __________ y = __________ 

z = __________ w = __________ 

a = __________ b = __________ 

p = __________ w = __________ 

x = __________ y = __________ 

z = __________ r = __________ 

a = __________ b = __________ 

146


32 

75 

t 

p 

b 

a 

87 

z 

x 

y 

18 

85 

x = __________ y = __________ 

z = __________ p = __________ 

t = __________ a = __________ 

b = __________ 

5. The polygon below is a REGULAR POLYGON. 

(a) Find the number of sides in the polygon. 

(b) Find the number of degrees in each 

exterior angle of the polygon 

(c) Find the number of degrees in each 

interior angle of the polygon 

6. (a) Find the number of degrees in each exterior angle of a regular decagon. 

(b) Find the number of degrees in each interior angle of a regular decagon. 

7. (a) Find the number of degrees in each exterior angle of a regular quadrilateral. 

(b) Find the number of degrees in each interior angle of a regular quadrilateral. 

8. Find the sum of the exterior angles of a polygon with 22 sides. 

9. Find the number of degrees in each exterior angle of a regular nonagon. 

10. Find the sum of the exterior angles of a septagon. 

11. Each exterior angle of a regular polygon contains 72. 


12. Each exterior angle of a regular polygon contains 30 degrees. 


13. Each exterior angle of a regular polygon contains 18 degrees. 


14. Find the sum of the exterior angles of a polygon with 11 sides. 

147

CHAPTER 4 - TRIANGLES Section 1- Classifying ... - Willets Geometry

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?