Section 1: Types of Quadrilaterals The Trapezoid ... - Willets Geometry

Section 1: Types of Quadrilaterals
A quadrilateral is a figure with four sides. It is named
by naming its vertices in a clockwise or counter-clockwise
direction. Although there are many types of quadrilaterals,
we will concentrate on three families of quadrilaterals:
Trapezoid
Kite
Parallelogram
The Trapezoid Family: Trapezoid and Isosceles Trapezoid
(a) A TRAPEZOID is a quadrilateral with two and only two sides parallel.
The parallel sides are called the BASES
The non-parallel sides are called the LEGS.
IASSOTS on the left and right sides are
supplementary so
(b) A trapezoid whose legs are congruent is called an ISOSCELES TRAPEZOID.
PT RQ
AB DC but
AD is not parallel to BC
m1 m3 180 and
m2 m4 180
P
T
An isosceles trapezoid has all the properties of a trapezoid
An isosceles trapezoid has a vertical
symmetry line, which means that when
folded from left to right, it coincides
with itself.
The base angles of an isosceles T
trapezoid are congruent
3
1 2 and 3 4
1
Opposite angles of an isosceles
trapezoid are supplementary
P
m1 m4 180 and m2 m3 180 In an isosceles trapezoid, the
diagonals are congruent so PR TQ
R
Q
D C
A B
D C
1
3
A B
P
P
4
R
1
2
T
T
3
4
Q
2
4
R
R
2
Q
Q

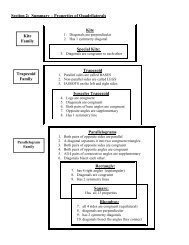
The Kite Family: Kite and Special Kite
(a) A KITE has two distinct pairs of congruent adjacent sides.
AB BC and AD CD
but AB is not congruent to AD
and BC is not congruent to CD
If diagonal AC is drawn, a kite can be thought of as two
isosceles triangles that have a common base. The segment
connecting the vertex angles of these isosceles triangles
is called the SYMMETRY DIAGONAL. If the kite is folded
left to right, it will coincide with itself.
BD is the symmetry diagonal.
The symmetry diagonal bisects the angles it connect.
If you fold a kite along the symmetry diagonal, any angles that
coincide will be congruent : 1 2 and 3 4
In a kite, the diagonals are perpendicular:
mAEB = mBEC = mDEC = mDEA = 90°
The symmetry diagonal of a kite bisects the other diagonal.
AE = EC but BE ≠ ED
(b) A kite whose diagonals are congruent is
called a SPECIAL KITE.
FH GE
A special kite has all the properties of a kite.
B
A C
D
B
A C
E
G
D
F H
E
1 2
A C
3
B
D
4

The Parallelogram Family: Parallelogram, Rectangle, Rhombus and Square
(a) A PARALLELOGRAM is a quadrilateral
with both pairs of opposite sides parallel.
AB CD and AD BC
A diagonal of a parallelogram, separates it
into two congruent triangles.
ADC CBA
Both pairs of opposite sides are congruent
AB DCandADBC Both pairs of opposite angles are congruent
1 3 and 2 4
All four pairs of consecutive angles are supplementary
m1 m2 180 and m2 m3 180
m3 m4 180 and m4 m1 180
The diagonals of a parallelogram bisect each other
M is the midpoint of AC and
M is the midpoint of BD
(b) A RECTANGLE is a parallelogram with 4 right angles.
A rectangle is an equiangular quadrilateral.
A rectangle has all the properties of a parallelogram.
The diagonals of a rectangle are congruent: AC BD
A rectangle has 2 symmetry lines. However, neither
diagonal is a symmetry diagonal.
D
A B
D
A B
1
D
4
A B
A D
B
D
A
2
M
3
C
A B
D
D
C
C
C
C
B
A B
C
C

(c) A RHOMBUS is a quadrilateral with all four sides congruent.
A rhombus is an equilateral quadrilateral.
AB BC DC
AD
A rhombus has all the properties of a parallelogram
The diagonals of a rhombus are perpendicular.
AC BD
A rhombus has two symmetry diagonals
A rhombus can be folded left to right and,
unlike a kite, it can also be folded top to bottom.
Any segments or angles that fold onto each other
are congruent.
The diagonals of a rhombus bisect the angles
they connect.
1 2, 3 4, 5 6 and 7 8
(d) A SQUARE is a quadrilateral with 4 right angles and 4 congruent sides.
A square is a regular quadrilateral since it is both equilateral
and equiangular.
A square has all the properties of a parallelogram,
all the properties of a rectangle and all the properties of a rhombus.
The diagonals of a square are perpendicular, congruent
and bisect each other.
AC BD, AC BD
M is the midpoint of AC
M is the midpoint of BD
A square has 4 symmetry lines,
two of which are symmetry diagonals.
A
A
1
D
5
7
A C
6
8
B
A
D
3
D
B
B
M
2
4
A
B
D
C
B
C
C
D
C