Numerical analysis of time discretization of optimal control problems

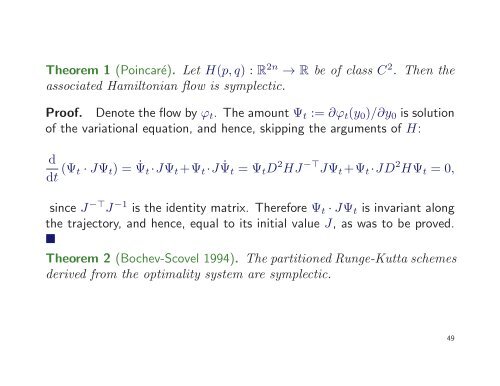
Theorem 1 (Poincaré). Let H(p,q) : R 2n → R be of class C 2 . Then the
associated Hamiltonian flow is symplectic.
Proof. Denote the flow by ϕt. The amount Ψt := ∂ϕt(y0)/∂y0 is solution
of the variational equation, and hence, skipping the arguments of H:
d
dt (Ψt·JΨt) = ˙ Ψt·JΨt+Ψt·J ˙ Ψt = ΨtD 2 HJ −⊤ JΨt+Ψt·JD 2 HΨt = 0,
since J −⊤ J −1 is the identity matrix. Therefore Ψt·JΨt is invariant along
the trajectory, and hence, equal to its initial value J, as was to be proved.
Theorem 2 (Bochev-Scovel 1994). The partitioned Runge-Kutta schemes
derived from the optimality system are symplectic.
49