Linear Matrix Inequalities in Control

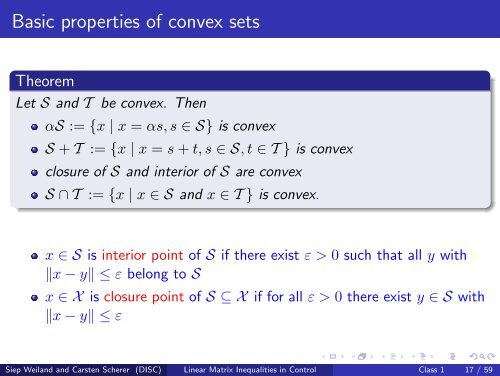
Basic properties of convex sets
Theorem
Let S and T be convex. Then
αS := {x | x = αs, s ∈ S} is convex
S + T := {x | x = s + t, s ∈ S, t ∈ T } is convex
closure of S and interior of S are convex
S ∩ T := {x | x ∈ S and x ∈ T } is convex.
x ∈ S is interior point of S if there exist ε > 0 such that all y with
x − y ≤ ε belong to S
x ∈ X is closure point of S ⊆ X if for all ε > 0 there exist y ∈ S with
x − y ≤ ε
Siep Weiland and Carsten Scherer (DISC) Linear Matrix Inequalities in Control Class 1 17 / 59