Chapter 5 - WebRing

CHAPTER 5. MAGNETIC SYSTEMS 249
(a) (b)
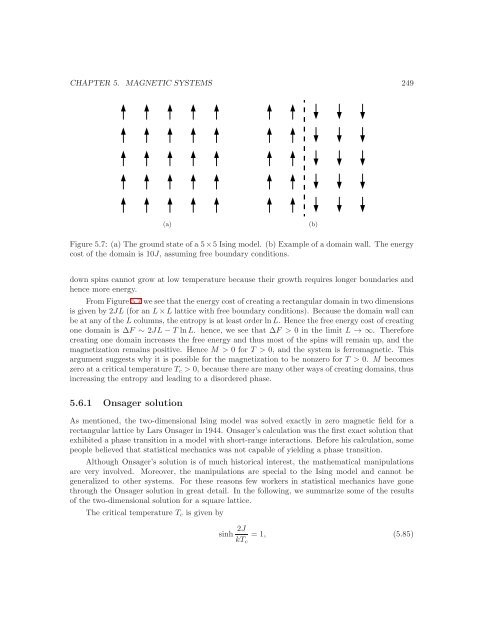
Figure 5.7: (a) The ground state of a 5×5 Ising model. (b) Example of a domain wall. The energy
cost of the domain is 10J, assuming free boundary conditions.
down spins cannot grow at low temperature because their growth requires longer boundaries and
hence more energy.
FromFigure5.7weseethatthe energycostofcreatingarectangulardomainin twodimensions
is given by 2JL (for an L×L lattice with free boundary conditions). Because the domain wall can
be at any of the L columns, the entropy is at least order lnL. Hence the free energy cost of creating
one domain is ∆F ∼ 2JL−T lnL. hence, we see that ∆F > 0 in the limit L → ∞. Therefore
creating one domain increases the free energy and thus most of the spins will remain up, and the
magnetization remains positive. Hence M > 0 for T > 0, and the system is ferromagnetic. This
argument suggests why it is possible for the magnetization to be nonzero for T > 0. M becomes
zero at a critical temperature Tc > 0, because there are many other ways of creating domains, thus
increasing the entropy and leading to a disordered phase.
5.6.1 Onsager solution
As mentioned, the two-dimensional Ising model was solved exactly in zero magnetic field for a
rectangular lattice by Lars Onsager in 1944. Onsager’s calculation was the first exact solution that
exhibited a phase transition in a model with short-range interactions. Before his calculation, some
people believed that statistical mechanics was not capable of yielding a phase transition.
Although Onsager’s solution is of much historical interest, the mathematical manipulations
are very involved. Moreover, the manipulations are special to the Ising model and cannot be
generalized to other systems. For these reasons few workers in statistical mechanics have gone
through the Onsager solution in great detail. In the following, we summarize some of the results
of the two-dimensional solution for a square lattice.
The critical temperature Tc is given by
sinh 2J
= 1, (5.85)
kTc