Simply Sequentially Additive Labeling of Some Special Trees

Simply sequentially additive labeling 6511
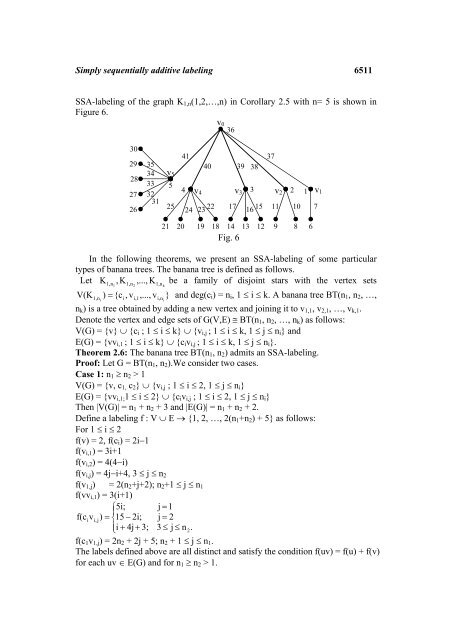
SSA-labeling of the graph K1,n(1,2,…,n) in Corollary 2.5 with n= 5 is shown in
Figure 6.
30
29
28
27
26
35
34
33
32
31
In the following theorems, we present an SSA-labeling of some particular
types of banana trees. The banana tree is defined as follows.
K , K ,..., K be a family of disjoint stars with the vertex sets
Let 1, n1
1, n2
1, nk
V(K n ) i {ci
, vi,1,...,
vi,
ni
21
v5
5
25
4
20
41
24
v4
19
40
22
23
18
v0
36
1, = } and deg(ci) = ni, 1 ≤ i ≤ k. A banana tree BT(n1, n2, …,
nk) is a tree obtained by adding a new vertex and joining it to v1,1, v2,1, …, vk,1.
Denote the vertex and edge sets of G(V,E) ≅ BT(n1, n2, …, nk) as follows:
V(G) = {v} ∪ {ci ; 1 ≤ i ≤ k} ∪ {vi,j ; 1 ≤ i ≤ k, 1 ≤ j ≤ ni} and
E(G) = {vvi,1 ; 1 ≤ i ≤ k} ∪ {civi,j ; 1 ≤ i ≤ k, 1 ≤ j ≤ ni}.
Theorem 2.6: The banana tree BT(n1, n2) admits an SSA-labeling.
Proof: Let G = BT(n1, n2).We consider two cases.
Case 1: n1 ≥ n2 > 1
V(G) = {v, c1, c2} ∪ {vi,j ; 1 ≤ i ≤ 2, 1 ≤ j ≤ ni}
E(G) = {vvi,1;1 ≤ i ≤ 2} ∪ {civi,j ; 1 ≤ i ≤ 2, 1 ≤ j ≤ ni}
Then |V(G)| = n1 + n2 + 3 and |E(G)| = n1 + n2 + 2.
Define a labeling f : V ∪ E → {1, 2, …, 2(n1+n2) + 5} as follows:
For 1 ≤ i ≤ 2
f(v) = 2, f(ci) = 2i−1
f(vi,1) = 3i+1
f(vi,2) = 4(4−i)
f(vi,j) = 4j−i+4, 3 ≤ j ≤ n2
f(v1,j) = 2(n2+j+2); n2+1 ≤ j ≤ n1
f(vvi,1) = 3(i+1)
⎪
⎧5i;
j = 1
f(ci
vi,
j)
= ⎨15
− 2i; j = 2
⎪⎩ i + 4j + 3; 3 ≤ j ≤ n 2.
f(c1v1,j) = 2n2 + 2j + 5; n2 + 1 ≤ j ≤ n1.
The labels defined above are all distinct and satisfy the condition f(uv) = f(u) + f(v)
for each uv ∈ E(G) and for n1 ≥ n2 > 1.
17
14
39
v3
Fig. 6
3
15
16
13
38
12
37
v2
11
9
2
10
8
1
6
v1
7