Alg 1 TE Lesson 10-8
Alg 1 TE Lesson 10-8
Alg 1 TE Lesson 10-8
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
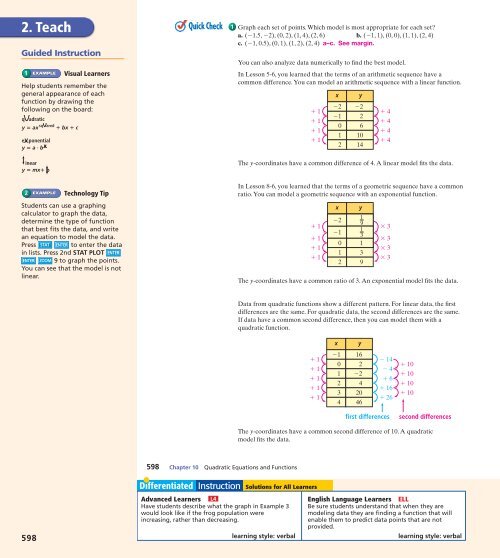
2. Teach<br />
Guided Instruction<br />
1<br />
Visual Learners<br />
Help students remember the<br />
general appearance of each<br />
function by drawing the<br />
following on the board:<br />
2<br />
EXAMPLE<br />
q adratic<br />
y ax sq ared bx c<br />
e ponential<br />
y a · b<br />
inear<br />
y mx b<br />
EXAMPLE<br />
Technology Tip<br />
Students can use a graphing<br />
calculator to graph the data,<br />
determine the type of function<br />
that best fits the data, and write<br />
an equation to model the data.<br />
Press<br />
to enter the data<br />
in lists. Press 2nd STAT PLOT<br />
9 to graph the points.<br />
You can see that the model is not<br />
linear.<br />
Quick Check<br />
1<br />
Graph each set of points. Which model is most appropriate for each set?<br />
a. (-1.5, -2), (0, 2), (1, 4), (2, 6) b. (-1, 1), (0, 0), (1, 1), (2, 4)<br />
c. (-1, 0.5), (0, 1), (1, 2), (2, 4) a–c. See margin.<br />
You can also analyze data numerically to find the best model.<br />
In <strong>Lesson</strong> 5-6, you learned that the terms of an arithmetic sequence have a<br />
common difference. You can model an arithmetic sequence with a linear function.<br />
1<br />
1<br />
1<br />
1<br />
The y-coordinates have a common difference of 4. A linear model fits the data.<br />
In <strong>Lesson</strong> 8-6, you learned that the terms of a geometric sequence have a common<br />
ratio. You can model a geometric sequence with an exponential function.<br />
1<br />
1<br />
1<br />
1<br />
x<br />
2 2<br />
1 2<br />
0 6<br />
1 <strong>10</strong><br />
x<br />
2<br />
1<br />
0<br />
The y-coordinates have a common ratio of 3. An exponential model fits the data.<br />
y<br />
2 14<br />
y<br />
1<br />
9<br />
1<br />
3<br />
1<br />
1 3<br />
2 9<br />
4<br />
4<br />
4<br />
4<br />
3<br />
3<br />
3<br />
3<br />
Data from quadratic functions show a different pattern. For linear data, the first<br />
differences are the same. For quadratic data, the second differences are the same.<br />
If data have a common second difference, then you can model them with a<br />
quadratic function.<br />
x<br />
y<br />
1<br />
1<br />
1<br />
1<br />
1<br />
1 16<br />
0 2<br />
1 2<br />
2 4<br />
3 20<br />
4 46<br />
14<br />
4<br />
6<br />
16<br />
26<br />
<strong>10</strong><br />
<strong>10</strong><br />
<strong>10</strong><br />
<strong>10</strong><br />
first differences<br />
second differences<br />
The y-coordinates have a common second difference of <strong>10</strong>. A quadratic<br />
model fits the data.<br />
598 Chapter <strong>10</strong> Quadratic Equations and Functions<br />
598<br />
Advanced Learners L4<br />
Have students describe what the graph in Example 3<br />
would look like if the frog population were<br />
increasing, rather than decreasing.<br />
learning style: verbal<br />
English Language Learners ELL<br />
Be sure students understand that when they are<br />
modeling data they are finding a function that will<br />
enable them to predict data points that are not<br />
provided.<br />
learning style: verbal