You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
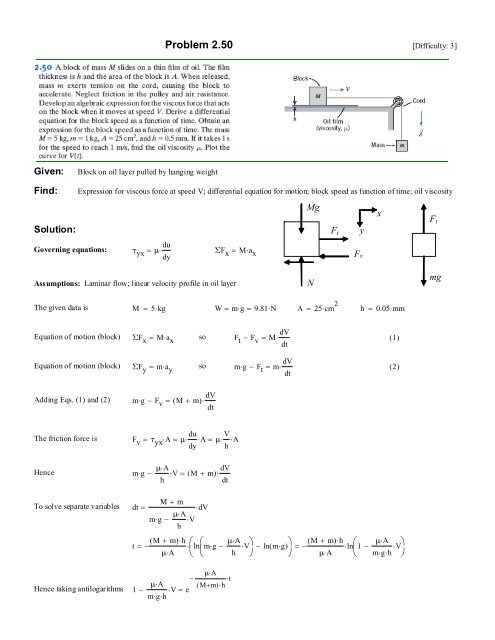
<strong>Problem</strong> 2.50 [Difficulty: 3]<br />
Given:<br />
Block on oil layer pulled by hanging weight<br />
Find:<br />
Solution:<br />
Expression for viscous force at speed V; differential equation for motion; block speed as function of time; oil viscosity<br />
Mg<br />
x<br />
Governing equations: τ yx = μ⋅<br />
du<br />
ΣF<br />
dy<br />
x = Ma ⋅ x<br />
F v<br />
F t<br />
y<br />
F t<br />
Assumptions: Laminar flow; linear velocity profile in oil layer<br />
N<br />
mg<br />
The given data is M = 5⋅kg<br />
W = m⋅g<br />
= 9.81⋅N<br />
A = 25⋅cm 2 h = 0.05⋅mm<br />
Equation of motion (block) ΣF x = Ma ⋅ x<br />
so F t − F v<br />
Equation of motion (block) ΣF y = ma ⋅ y<br />
so mg ⋅<br />
= M⋅<br />
dV<br />
( 1)<br />
dt<br />
− F t = m⋅<br />
dV<br />
( 2)<br />
dt<br />
Adding Eqs. (1) and (2)<br />
mg ⋅<br />
− F v = ( M + m) ⋅<br />
dV<br />
dt<br />
The friction force is F v = τ yx ⋅A<br />
= μ⋅<br />
du ⋅A<br />
= μ⋅<br />
V ⋅A<br />
dy h<br />
Hence<br />
mg ⋅<br />
μ⋅A<br />
− ⋅V = ( M + m) ⋅<br />
dV<br />
h<br />
dt<br />
To solve separate variables<br />
dt =<br />
M + m<br />
⋅dV<br />
μ⋅A<br />
mg ⋅ − ⋅V<br />
h<br />
⎛<br />
⎝<br />
⎛<br />
⎝<br />
( M + m) ⋅h<br />
t = − ⋅⎜ln⎜m⋅g<br />
−<br />
μ⋅A<br />
μ⋅A<br />
h<br />
⋅V<br />
⎞<br />
⎠<br />
−<br />
ln( m⋅g)<br />
⎞<br />
⎠<br />
⎛<br />
⎝<br />
( M + m) ⋅h<br />
= − ⋅ln⎜1<br />
−<br />
μ⋅A<br />
μ⋅A<br />
mg ⋅ ⋅h<br />
⋅V<br />
⎞<br />
⎠<br />
Hence taking antilogarithms 1 −<br />
μ⋅A<br />
mg ⋅ ⋅h<br />
⋅V = e<br />
−<br />
μ⋅A<br />
⋅t<br />
( M+<br />
m) ⋅h
⎡<br />
μ⋅A<br />
Finally V mg ⋅ ⋅h<br />
−<br />
( M<br />
1 e<br />
+ m) ⋅<br />
⋅t<br />
⎢<br />
h<br />
= ⋅⎣<br />
−<br />
The maximum velocity is V =<br />
mg ⋅ ⋅h<br />
μ⋅A<br />
μ⋅A<br />
In Excel:<br />
⎤<br />
⎥⎦<br />
The data is M = 5.00 kg To find the viscosity for which the speed is 1 m/s after 1 s<br />
m = 1.00 kg use Goal Seek with the velocity targeted to be 1 m/s by varying<br />
g = 9.81 m/s 2 the viscosity in the set of cell below:<br />
0 = 1.30 N.s/m 2<br />
A = 25 cm 2 t (s) V (m/s)<br />
h = 0.5 mm 1.00 1.000<br />
Speed V of Block vs Time t<br />
t (s) V (m/s)<br />
0.00 0.000<br />
0.10 0.155<br />
0.20 0.294<br />
0.30 0.419<br />
0.40 0.531<br />
0.50 0.632<br />
0.60 0.722<br />
0.70 0.803<br />
0.80 0.876<br />
0.90 0.941<br />
1.00 1.00<br />
1.10 1.05<br />
1.20 1.10<br />
1.30 1.14<br />
1.40 1.18<br />
1.50 1.21<br />
1.60 1.25<br />
1.70 1.27<br />
1.80 1.30<br />
1.90 1.32<br />
2.00 1.34<br />
2.10 1.36<br />
2.20 1.37<br />
2.30 1.39<br />
2.40 1.40<br />
2.50 1.41<br />
2.60 1.42<br />
2.70 1.43<br />
2.80 1.44<br />
2.90 1.45<br />
3.00 1.46<br />
1.6<br />
1.4<br />
1.2<br />
1.0<br />
V (m/s) 0.8<br />
0.6<br />
0.4<br />
0.2<br />
0.0<br />
0.0 0.5 1.0 1.5 2.0 2.5 3.0<br />
t (s)
<strong>Problem</strong> 2.63 [Difficulty: 4]
<strong>Problem</strong> 2.73 [Difficulty: 4]<br />
Given:<br />
Find:<br />
Conical bearing geometry<br />
Expression for shear stress; Viscous torque on shaft<br />
Solution:<br />
ds<br />
dz<br />
Basic equation τ = μ⋅<br />
du dT = r⋅τ⋅dA<br />
Infinitesimal shear torque<br />
dy<br />
Assumptions: Newtonian fluid, linear velocity profile (in narrow clearance gap), no slip condition<br />
tan( θ)<br />
r<br />
= so r = z⋅tan( θ)<br />
z<br />
Then τ = μ⋅<br />
du = μ⋅<br />
∆u = μ⋅<br />
( ω⋅r<br />
− 0)<br />
=<br />
dy ∆y ( a − 0)<br />
μω ⋅ ⋅z⋅tan( θ)<br />
a<br />
a<br />
z<br />
r<br />
Section AA<br />
AA<br />
U= ωr<br />
As we move up the device, shear stress increases linearly (because rate of shear strain does)<br />
But from the sketch dz = ds⋅cos( θ)<br />
dA = 2⋅π⋅r⋅ds<br />
= 2⋅π⋅r⋅<br />
dz<br />
cos( θ)<br />
The viscous torque on the element of area is dT = r⋅τ⋅dA<br />
r μω ⋅ ⋅z⋅tan(<br />
θ)<br />
dz<br />
= ⋅ ⋅2⋅π⋅r⋅<br />
dT =<br />
a<br />
cos( θ)<br />
Integrating and using limits z = H and z = 0<br />
πμ ⋅ ⋅ω⋅tan( θ) 3 ⋅H 4<br />
T =<br />
2a ⋅ ⋅cos( θ)<br />
2⋅π⋅μ⋅ω⋅z 3 ⋅tan( θ) 3<br />
⋅dz<br />
a⋅cos( θ)<br />
Solving for µ<br />
μ =<br />
2a ⋅ ⋅cos( θ)<br />
⋅T<br />
πω ⋅ ⋅tan( θ) 3 ⋅H 4<br />
Using given data H = 25⋅mm<br />
θ = 30⋅deg<br />
a = 0.2⋅mm<br />
ω = 75⋅<br />
rev T = 0.325⋅N⋅m<br />
s<br />
μ<br />
=<br />
2a ⋅ ⋅cos( θ)<br />
T<br />
μ = 1.012⋅<br />
Ns πω ⋅ ⋅tan( θ) ⋅H 4<br />
m 2<br />
From Fig. A.2, at 20 o C, CASTOR OIL has this viscosity!