Skopje, Makedonija INTERPOLATION POLYNOMIALS VIA AIFS 1 ...

More documents

Recommendations

Info

$Fractional and operational calculus with generalized fractional ...$

$Numerical Methods Course Notes Version 0.1 (UCSD Math 174, Fall ...$

70 LJ. KOCIĆ, S. G-ZAJKOVA, V. ANDOVA where [ m L (x) = 1 x − a c − a ( ) 2 x − a ... c − a ( ) n ] T x − a c − a is the ”left” monomial basis, coincides with P n (x) on the left subinterval [a, c], and, at the same time, the ”right” polynomial Pn R (x) =(a R ) T · m R (x), x ∈ [c, b] where [ m R (x) = 1 x − c b − c ( ) 2 x − c ... b − c ( ) n ] T x − c b − c coincides with P n (x) on the right subinterval [c, b]. In fact, it is a binary subdivision problem for the monomial basis. Now, the monomial and Bernstein basis of degree n are connected by m(x) =T BM · b(x) (3.3) where T BM is an n × n upper-triangular matrix of the form (for n =1, 2, 3and4) ⎡ ⎤ ⎡ [ ] 1 1 [1] , , ⎣ 1 1 1 ⎤ 1 1 1 1 1 0 1/4 1/2 3/4 1 0 1/2 1 ⎦ , 0 1 ⎢ 0 0 1/6 1/2 1 ⎥ 0 0 1 ⎣ 0 0 0 1/4 1 ⎦ , ... 0 0 0 0 1 Multiplying both sides of (3.3) by the vector a T from the left yields the last expression is Bernstein polynomial, defined on [0, 1]. Now, subdivision for given λ (formulae (2.4)) results in p L = S L (λ) · p and p R = S R (λ) · p. On the other hand, by inverting (3.3), which is always possible since all matrices T BM are regular, so that (T BM ) −1 always exists, one gets b(x) = T MB · m(x), where it is set (T BM ) −1 = T MB . Consequently, p T L · b(x) = ( p T ) ( ) L · T MB · m(x) = T T T MB · p L · m(x), which results in a L = T T MB · p L. Similarly, a R = T T MB · p R. Now, taking into account that p L = S L (λ) · p and p R = S R (λ) · p, andthat p = T T BM · a, we obtain { aL = T T MB · p L = T T MB · S L(λ) · p = ( T T MB · S ) L(λ) · T T BM · a =AL · a a R = T T MB · p R = T T MB · S R(λ) · p = ( T T MB · S R(λ) · TBM) T · a =AR · a Example 2. For ā T = [ −17/90 59/36 7/9 −97/36 −4/45 5/9 ] the polynomial P n (x), given by (3.1) is defined on [a, b] =[−2, 2]. The coefficients of the polynomial given by (3.2) are a T = [ 2 809/15 −7112/15 58288/45 −65024/45 5120/9 ]
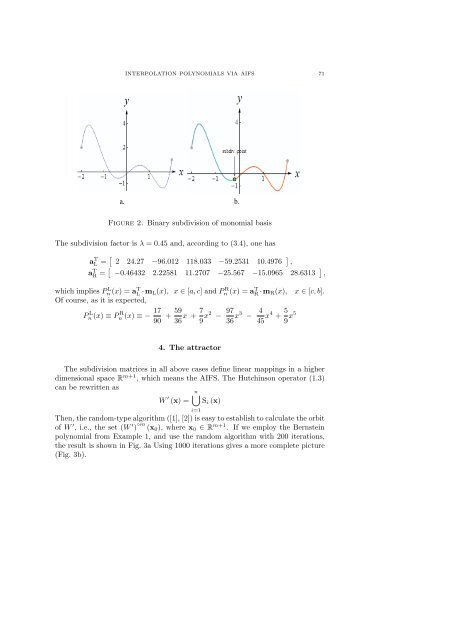
INTERPOLATION POLYNOMIALS VIA AIFS 71 a. b. Figure 2. Binary subdivision of monomial basis The subdivision factor is λ =0.45 and, according to (3.4), one has a T L = [ 2 24.27 −96.012 118.033 −59.2531 10.4976 ] , a T R = [ −0.46432 2.22581 11.2707 −25.567 −15.0965 28.6313 ] , which implies Pn L (x) =a T L ·m L(x), x ∈ [a, c] andPn R (x) =a T R ·m R(x), x ∈ [c, b]. Of course, as it is expected, Pn L (x) ≡ Pn R (x) ≡− 17 90 + 59 36 x + 7 9 x2 − 97 36 x3 − 4 45 x4 + 5 9 x5 4. The attractor The subdivision matrices in all above cases define linear mappings in a higher dimensional space R m+1 , which means the AIFS. The Hutchinson operator (1.3) can be rewritten as n⋃ W ′ (x) = S i (x) Then, the random-type algorithm ([1], [2]) is easy to establish to calculate the orbit of W ′ , i.e., the set (W ′ ) ◦m (x 0 ), where x 0 ∈ R m+1 . If we employ the Bernstein polynomial from Example 1, and use the random algorithm with 200 iterations, the result is shown in Fig. 3a Using 1000 iterations gives a more complete picture (Fig. 3b). i=1
Page 1 and 2: Matematiqki Bilten ISSN 0351-336X 3
Page 3 and 4: INTERPOLATION POLYNOMIALS VIA AIFS
Page 5 and 6: INTERPOLATION POLYNOMIALS VIA AIFS
Page 7: INTERPOLATION POLYNOMIALS VIA AIFS

Skopje, Makedonija INTERPOLATION POLYNOMIALS VIA AIFS 1 ...

You also want an ePaper? Increase the reach of your titles

Delete template?

Save as template?