The Riemann Integral

14 1. The Riemann Integral
1.2
1
0.8
y
0.6
0.4
0.2
0
0 0.2 0.4 0.6 0.8 1
x
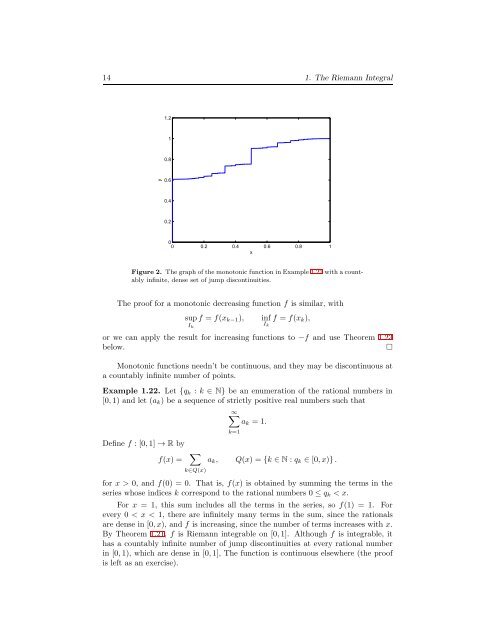
Figure 2. The graph of the monotonic function in Example 1.22 with a countably
infinite, dense set of jump discontinuities.
The proof for a monotonic decreasing function f is similar, with
sup
I k
f = f(x k−1 ),
inf
I k
f = f(x k ),
or we can apply the result for increasing functions to −f and use Theorem 1.23
below.
□
Monotonic functions needn’t be continuous, and they may be discontinuous at
a countably infinite number of points.
Example 1.22. Let {q k : k ∈ N} be an enumeration of the rational numbers in
[0,1) and let (a k ) be a sequence of strictly positive real numbers such that
∞∑
a k = 1.
Define f : [0,1] → R by
f(x) = ∑
k∈Q(x)
a k ,
k=1
Q(x) = {k ∈ N : q k ∈ [0,x)}.
for x > 0, and f(0) = 0. That is, f(x) is obtained by summing the terms in the
series whose indices k correspond to the rational numbers 0 ≤ q k < x.
For x = 1, this sum includes all the terms in the series, so f(1) = 1. For
every 0 < x < 1, there are infinitely many terms in the sum, since the rationals
are dense in [0,x), and f is increasing, since the number of terms increases with x.
By Theorem 1.21, f is Riemann integrable on [0,1]. Although f is integrable, it
has a countably infinite number of jump discontinuities at every rational number
in [0,1), which are dense in [0,1], The function is continuous elsewhere (the proof
is left as an exercise).