RADIAL ACCELERATION

5.2 Radial Acceleration 149
Example 5.3 Continued
2pr (Fig. 5.5). Then the time to make one revolution is T,
then the speed v is
v = di stan
tim
ce
e
= 2 pr
T
Therefore, T = 2pr/v. For each revolution there is an
angular displacement of ∆q = 2p radians, so
w = ∆ q
∆
t
= 2 p
T
Substituting T = 2pr/v and remembering that the radius is
half the diameter,
2p
w = = v 2p r/v
r = 13.0
m/
s
= 40.0 ra d
(0.650
m)
/2 s
2p
rad
Discussion Check: Time for one revolution is
40 . 0 rad/s
2.
04
m
= 0.157 s. Time to travel a distance 2pr = 2.04 m is
= 0.157 s. Looks good.
1 3.
0 m/s
You could have obtained this answer immediately by
looking back through the text for the equation w = v/r and
plugging in numbers, but the solution here shows that you
can re-create that equation. Here, and in many cases, there
is no need to memorize a formula if you understand the
concepts behind the formula. You are then less apt to make
a mistake by forgetting a factor or constant in the equation,
or by using an inappropriate formula. For another example,
if an object moves along a straight line at a constant velocity,
you know instantly that the displacement is the velocity
times the time interval—not because you have memorized
an equation (∆r = v ∆t), but because you understand the
concepts of displacement and velocity. This is the sort of
internalization of scientific thinking that you will develop
with more and more practice in problem solving.
Practice Problem 5.3
Rolling Drum
A cylindrical steel drum is tipped over and rolled along
the floor of a warehouse. If the drum has a radius of 0.40 m
and makes one complete turn every 8.0 s, how long does
it take to roll the drum 36 m?
5.2 RADIAL ACCELERATION
For a particle undergoing uniform circular motion, the magnitude of the velocity vector
is constant, but its direction is continuously changing. At any instant of time, the direction
of the instantaneous velocity is tangent to the path, as discussed in Section 3.2. Since
the direction of the velocity continually changes, the particle has a nonzero acceleration.
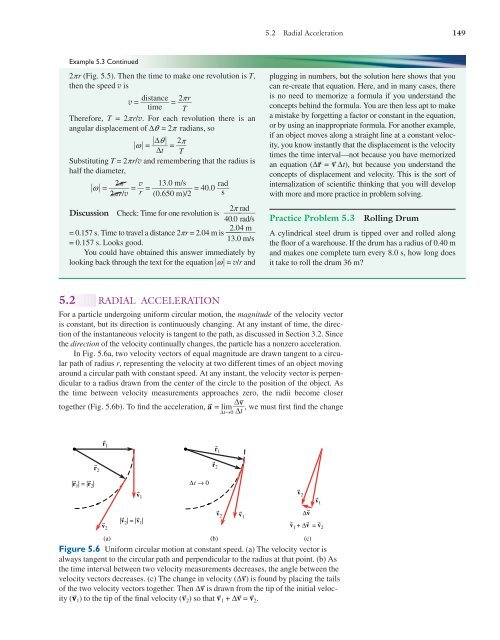
In Fig. 5.6a, two velocity vectors of equal magnitude are drawn tangent to a circular
path of radius r, representing the velocity at two different times of an object moving
around a circular path with constant speed. At any instant, the velocity vector is perpendicular
to a radius drawn from the center of the circle to the position of the object. As
the time between velocity measurements approaches zero, the radii become closer
together (Fig. 5.6b). To find the acceleration, a = lim ∆ v
,
∆t→0 ∆ t
we must first find the change
r 1
r 1
r
r 2
2
|r 1 | = |r 2 |
∆t → 0
v v 1 2
v 1
v 2 v 1
∆v
|v 2 | = |v 1 |
v 2 v 1 + ∆v = v 2
(a) (b) (c)
Figure 5.6 Uniform circular motion at constant speed. (a) The velocity vector is
always tangent to the circular path and perpendicular to the radius at that point. (b) As
the time interval between two velocity measurements decreases, the angle between the
velocity vectors decreases. (c) The change in velocity (∆v) is found by placing the tails
of the two velocity vectors together. Then ∆v is drawn from the tip of the initial velocity
(v 1 ) to the tip of the final velocity (v 2 ) so that v 1 + ∆v = v 2 .

150 Chapter 5 Circular Motion
In uniform circular motion, the
direction of the acceleration is
radially inward (toward the
center of the circular path).
v 5
v 6
a 5
a 1
a 2
a 6
v 1
v 2
v 4
a 4
a 3
v 3
Figure 5.7 In uniform circular
motion, the acceleration is
always directed toward the center
of the circle, perpendicular to
the velocity.
v 2
∆q
v1
∆v
Figure 5.8 The velocity vector
sweeps out an arc of a circle
whose “length” is nearly equal to
that of the chord ∆v.
in velocity ∆v for a very short time interval. Figure 5.6c shows that as the time interval
∆t approaches zero, the angle between the two velocities also approaches zero and ∆v
becomes perpendicular to the velocity.
Since ∆v is perpendicular to the velocity, it is directed along a radius of the circle.
Inspection of Figs. 5.6b and 5.6c shows that ∆v is radially inward (toward the center of
the circle). Since the acceleration a has the same direction as ∆v (in the limit ∆t → 0),
the acceleration is also directed radially inward (Fig. 5.7)—that is, along a radius of the
circular path toward the center of the circle. The acceleration of an object undergoing
uniform circular motion is often called the radial acceleration a r . The word radial here
just reminds us of the direction of the acceleration. (A synonym for radial acceleration
is centripetal acceleration. Centripetal means “toward the center.”)
Magnitude of the Radial Acceleration
To find the magnitude of the radial acceleration for uniform circular motion, we must
find the change in velocity ∆v for a time interval ∆t in the limit ∆t → 0. The velocity
keeps the same magnitude but changes direction at a steady rate, equal to the angular
velocity w. In a time interval ∆t, the velocity v rotates through an angle equal to the
angular displacement ∆q = w ∆t. During this time interval, the velocity vector sweeps
out an arc of a circle of “radius” v (Fig. 5.8). In the limit ∆t → 0, the magnitude of ∆v
becomes equal to the arc length, since a very short arc approaches a straight line. Then
∆v = arc length = radius of circle × angle subtended
= v ∆q = v w ∆t
Acceleration is the rate of change of velocity, so the magnitude of the radial acceleration
is
a r = a = ∆ v
= vw (w in radians per unit time) (5-11)
∆t
where absolute value symbols are used with the vector quantities to indicate their magnitudes.
Velocity and angular velocity are not independent; v = w r. It is usually most
convenient to write the magnitude of the radial acceleration in terms of one or the other
of these two quantities. So we write the radial acceleration in two other equivalent ways
using v = wr:
a r = v 2
r
or a r = w 2 r (w in radians per unit time) (5-12)
Note that Eqs. (5-11) and (5-12) are valid only when w is measured in radians per
unit time (normally rad/s, but rad/min or rad/h would be correct).
Example 5.4
A Spinning CD
If a CD spins at 210 rpm, what is the radial acceleration
of a point on the outer rim of the CD? The
CD is 12 cm in diameter.
Strategy From the number of revolutions per minute,
we can find the frequency and the angular velocity. The
angular velocity and the radius of the CD enable us to
calculate the radial acceleration.
Solution We convert 210 rpm into a frequency in revolutions
per second (Hz).
rev
1
f = 210 × m in
m in
6 0 s
= 3.5 re v
s
= 3.5 Hz
For each revolution, the CD rotates through an angle of
2p radians. The angular velocity is
w = 2p f = 2p rad ians
× 3.5 re v
rev
s
= 7.0p rad/s
Then using Eq. (5-11), the radial acceleration is
a r = w 2 r = (7.0p rad/s) 2 × 0.060 m = 29 m/s 2
Continued on next page

5.2 Radial Acceleration 151
Example 5.4 Continued
Discussion When finding the radial acceleration, use
whichever form of Eq. (5-12) is more convenient. For rotating
objects such as the spinning CD, it’s usually easiest to
think in terms of the angular velocity. For an object moving
around a circle, such as a satellite in orbit whose speed is
known, it might be easier to use v 2 /r. Since the two equations
are equivalent, either can be used in any situation.
Practice Problem 5.4 Radial Acceleration
of a Point on an Old Record
What is the radial acceleration of a point 25.4 cm from
the center of a record that is rotating at 78 rpm on a
turntable?
Now that we know the magnitude and direction of the acceleration of any object in
uniform circular motion, we can use Newton’s second law to relate the net force acting
on the object to the speed and radius of its motion. The net force is found in the usual
way: each of the individual forces acting on the object is identified and then the forces
are added as vectors. Every force acting must be exerted by some other object. Resist
the temptation to add in a new, separate force just because something moves in a circle.
For an object to move in a circle at constant speed, real, physical forces such as gravity,
tension, normal forces, and friction must act on it; these forces combine to produce a
net force that has the correct magnitude and is always perpendicular to the velocity of
the object.
Problem-Solving Strategy for an Object in Uniform Circular Motion
1. Begin as for any Newton’s second law problem: identify all the forces acting on
the object and draw a free-body diagram.
2. Choose perpendicular axes at the point of interest so that one is radial and the
other is tangent to the circular path.
3. Find the radial component of each force.
4. Apply Newton’s second law as follows:
ΣF r = ma r
where ΣF r is the radial component of the net force and the radial component of
the acceleration is
a r = v 2
r = w 2 r
(For uniform circular motion, neither the net force nor the acceleration has a
tangential component.)
Example 5.5
The Hammer Throw
An athlete whirls a 4.00-kg hammer six or
seven times around and then releases it.
Although the purpose of whirling it around
several times is to increase the hammer’s speed, assume
that just before the hammer is released, it moves at constant
speed along a circular arc of radius 1.7 m. At the
instant she releases the hammer, it is 1.0 m above the
ground and its velocity is directed 40° above the horizontal.
The hammer lands a horizontal distance of 74.0 m
away. What force does the athlete apply to the grip just
before she releases it? Neglect air resistance.
Strategy After release, the only force acting on the
hammer is gravity. The hammer moves in a parabolic trajectory
like any other projectile. By analyzing the projectile
motion of the hammer, we can find the speed of the
hammer just after its release. Just before release, the
Continued on next page

152 Chapter 5 Circular Motion
Example 5.5 Continued
forces acting on the hammer are the tension in the cable
and gravity. We can relate the net force on the hammer to
its radial acceleration, calculated from the speed and
radius of its path. The problem becomes two subproblems,
one dealing with circular motion and the other with
projectile motion. The final velocity for the circular
motion is the initial velocity for the projectile motion.
Solution During its projectile motion, the initial
velocity has magnitude v i (to be determined) and direction
q = 40° above the horizontal. Choosing the +y-axis
pointing up, the displacement of the hammer (in component
form) is ∆x = 74 m and ∆y = –1.0 m (Fig. 5.9), the
acceleration of the hammer is a x = 0 and a y = –g, and the
initial velocity is v ix = v i cos q and v iy = v i sin q . Then,
from Eqs. (3-11) and (3-12),
∆x = (v i cos q ) ∆t and ∆y = (v i sin q ) ∆t – 1 2 g(∆t)2
Solving the left equation for ∆t and substituting into the
right equation gives
∆x
∆y = v i sinq – 1
vi cosq
2 g ∆x
v i cos q 2
After a bit of algebra, we can solve for v i . First we multiply
through by 2v 2 i cos 2 q :
2v 2 i cos 2 q ∆y = 2v 2 i cos 2 q ∆ x sin
q
– 2 v i 2 cos 2
cos
q
q
2
g ∆x
v c
os i q 2
Moving the first term on the right side to the left side and
factoring out v 2 i ,
v 2 i (2∆y cos 2 q – 2∆x cos q sinq ) = –g(∆x) 2
Now we solve for v i :
g(∆x)
v i =
2
2∆x cos q sin q – 2∆y cos 2 q
9.80 m/s =
2 × (74.0 m) 2
2(74.0 m) cos 40° sin 40° – 2(–1.0 m) cos 2 40°
= 26.9 m/s
The net force on the hammer
can be found from Newton’s second
law. The two forces acting on the
hammer are due to the tension in the
cable and to gravity (Fig. 5.10). We
neglect the gravitational force,
assuming that the hammer’s weight
is small compared to the tension in
the cable. Then the tension in the
cable is the only significant force
acting on the hammer. Assuming
uniform circular motion, the cable
pulls radially inward and causes a radial acceleration of
magnitude v 2 /r. Newton’s second law in the radial direction
is
ΣF r = T = ma r = m v 2
r
Substituting numerical values,
T =
4.00 kg × (26.9 m/s) 2
= 1700 N
1.7 m
The tension is much larger than the weight of the hammer
(≈40 N), so the assumption that we could ignore the
weight is justified. The athlete must apply a force of magnitude
1700 N—almost 400 lb—to the grip.
Discussion This example demonstrates the cumulative
nature of physics concepts. The basic concepts keep
reappearing, to be used over and over and to be extended
for use in new contexts. Part of the problem involves new
concepts (radial acceleration); the rest of the problem
involves old material (Newton’s second law, projectile
motion, and tension in a cord).
Practice Problem 5.5
Rotating Carousel
A horse located 8.0 m from the central axis of a rotating
carousel moves at a speed of 6.0 m/s. The horse is at a
fixed height (it does not move up and down). What is the
net force acting on a child seated upon this horse? The
child’s weight is 130 N.
T
mg
Figure 5.10
FBD for the hammer
just before its release.
(Not to scale.)
40°
Projectile motion
(parabolic trajectory)
y
x
Uniform
circular
motion
Release
point
∆ x = 74 m
∆ y = –1.0 m
Figure 5.9
Path of the hammer from just before its release until it hits the ground. (Distances are not to scale.)

5.3 Unbanked and Banked Curves 153
Example 5.6
Conical Pendulum
Suppose you whirl a stone in a horizontal circle at a slow
speed so that the weight of the stone is not negligible
compared to the tension in the cord. Then the cord cannot
be horizontal—the tension must have a vertical component
to cancel the weight and leave a horizontal net force
(Fig. 5.11). If the cord has length L, the stone has mass m,
and the cord makes an angle f with the vertical direction,
what is the constant angular speed of the stone?
Strategy The net force must point toward the center of
the circle, since the stone is in uniform circular motion.
With the stone in the position depicted in Fig. 5.11a, the
direction of the net force is along the +x-axis. This time
the tension in the cord does not pull toward the center, but
the net force does.
Solution Start by drawing a free-body diagram (Fig.
5.11b). Now apply Newton’s second law in component
form. The acceleration has components a x = w 2 r and a y =0.
For the x-components,
L
r = L sin f
ΣF x = T sin f = ma x = mw 2 r
f
y
(a)
Figure 5.11
(a) A stone is whirled in a horizontal circle of radius r = L sin f.
(b) A free-body diagram for the stone.
x
y
f
mg
T
(b)
x
Since the problem does not specify r, we must express r
in terms of L and f. In Fig. 5.11a, the radius forms a right
triangle with the cord and the y-axis. Then
r = L sin f
and
ΣF x =T sin f = mw 2 Lsin f
Therefore, T = mw 2 L. For the y-components,
ΣF y = T cos f – mg = ma y = 0 ⇒ T cos f = mg
Now we eliminate the tension:
(mw 2 L) cos f = mg
Solving for w ,
w =
g
L cos
f
Discussion We should check the dimensions of the
final expression. Since cos f is dimensionless,
[L] /[T
[ L
]2
]
= 1
[T]
which is correct for w (SI unit rad/s).
Another check is to ask how w and f are related for a
given length cord. As f increases toward 90°, the cord
gets closer to horizontal and the radius increases. In our
expression, as f increases, cosf decreases and, therefore,
w increases, in accordance with experience: the stone
would have to be whirled faster and faster to make the
cord more nearly horizontal.
Conceptual Practice Problem 5.6
Pendulum on the Moon
Conical
Examine the result of Example 5.6 to see how w depends
on g, all other things being equal. Where the gravitational
field is weaker, do you have to whirl the stone faster or
more slowly to keep the cord at the same angle f? Is that
in accord with your intuition?
5.3 UNBANKED AND BANKED CURVES
When you drive an automobile in a circular path along an unbanked roadway, friction acting
on the tires due to the pavement acts to keep the automobile moving in a curved path.
This frictional force acts sideways, toward the center of the car’s circular path (Fig. 5.12).
The frictional force might also have a tangential component; for example, if the car is
braking, a component of the frictional force makes the car slow down by acting backward
(opposite to the car’s velocity). For now we assume that the car’s speed is constant and
that the forward or backward component of the frictional force is negligibly small.