Trigonometry Handout

Trigonometry
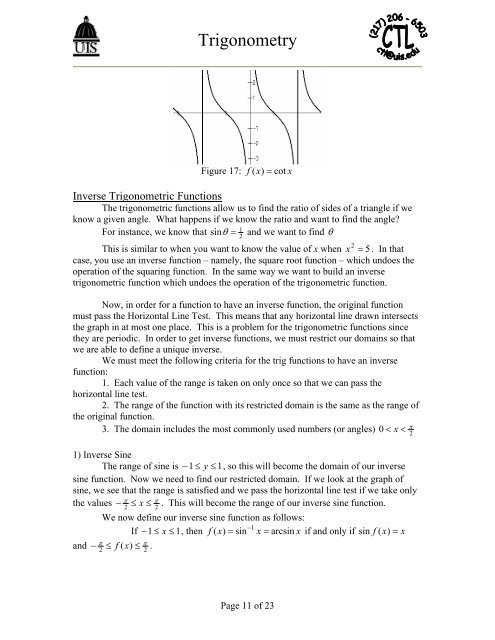
Figure 17:
f ( x)
= cot x
Inverse Trigonometric Functions
The trigonometric functions allow us to find the ratio of sides of a triangle if we
know a given angle. What happens if we know the ratio and want to find the angle?
1
For instance, we know that sin θ = and we want to find θ
2
This is similar to when you want to know the value of x when x
2 = 5 . In that
case, you use an inverse function – namely, the square root function – which undoes the
operation of the squaring function. In the same way we want to build an inverse
trigonometric function which undoes the operation of the trigonometric function.
Now, in order for a function to have an inverse function, the original function
must pass the Horizontal Line Test. This means that any horizontal line drawn intersects
the graph in at most one place. This is a problem for the trigonometric functions since
they are periodic. In order to get inverse functions, we must restrict our domains so that
we are able to define a unique inverse.
We must meet the following criteria for the trig functions to have an inverse
function:
1. Each value of the range is taken on only once so that we can pass the
horizontal line test.
2. The range of the function with its restricted domain is the same as the range of
the original function.
π
3. The domain includes the most commonly used numbers (or angles) 0 < x <
2
1) Inverse Sine
The range of sine is −1 ≤ y ≤ 1, so this will become the domain of our inverse
sine function. Now we need to find our restricted domain. If we look at the graph of
sine, we see that the range is satisfied and we pass the horizontal line test if we take only
π π
the values − ≤ x ≤ . This will become the range of our inverse sine function.
2 2
We now define our inverse sine function as follows:
−1
If −1 ≤ x ≤ 1, then f ( x)
= sin x = arcsin x if and only if sin f ( x)
= x
π
π
and − ≤ f ( x)
≤ .
2 2
Page 11 of 23