alternative lecture notes - Rational points and algebraic cycles
alternative lecture notes - Rational points and algebraic cycles
alternative lecture notes - Rational points and algebraic cycles
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
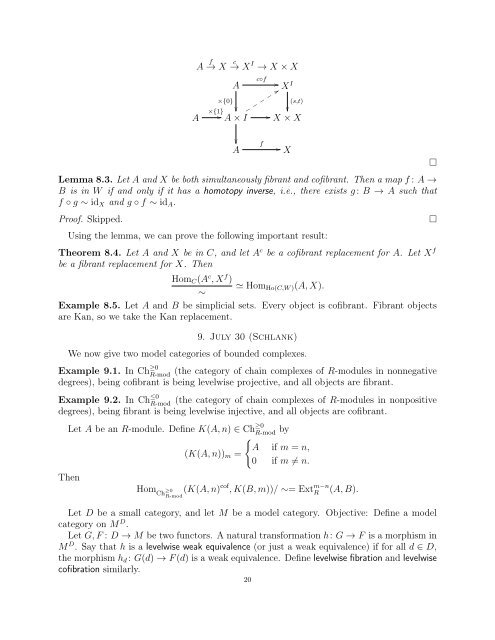
A f → X c → X I → X × X<br />
×{0}<br />
A<br />
c◦f<br />
<br />
X I<br />
<br />
<br />
(s,t)<br />
<br />
<br />
A ×{1} A × I X × X<br />
A<br />
Lemma 8.3. Let A <strong>and</strong> X be both simultaneously fibrant <strong>and</strong> cofibrant. Then a map f : A →<br />
B is in W if <strong>and</strong> only if it has a homotopy inverse, i.e., there exists g : B → A such that<br />
f ◦ g ∼ id X <strong>and</strong> g ◦ f ∼ id A .<br />
Proof. Skipped.<br />
Using the lemma, we can prove the following important result:<br />
Theorem 8.4. Let A <strong>and</strong> X be in C, <strong>and</strong> let A c be a cofibrant replacement for A. Let X f<br />
be a fibrant replacement for X. Then<br />
Hom C (A c , X f )<br />
≃ Hom Ho(C,W ) (A, X).<br />
∼<br />
Example 8.5. Let A <strong>and</strong> B be simplicial sets. Every object is cofibrant. Fibrant objects<br />
are Kan, so we take the Kan replacement.<br />
f<br />
X<br />
9. July 30 (Schlank)<br />
We now give two model categories of bounded complexes.<br />
Example 9.1. In Ch ≥0<br />
R-mod<br />
(the category of chain complexes of R-modules in nonnegative<br />
degrees), being cofibrant is being levelwise projective, <strong>and</strong> all objects are fibrant.<br />
Example 9.2. In Ch ≤0<br />
R-mod<br />
(the category of chain complexes of R-modules in nonpositive<br />
degrees), being fibrant is being levelwise injective, <strong>and</strong> all objects are cofibrant.<br />
Let A be an R-module. Define K(A, n) ∈ Ch ≥0<br />
R-mod<br />
{<br />
by<br />
A if m = n,<br />
(K(A, n)) m =<br />
0 if m ≠ n.<br />
Then<br />
Hom Ch<br />
≥0 (K(A, n) cof , K(B, m))/ ∼= Ext m−n<br />
R (A, B).<br />
R-mod<br />
Let D be a small category, <strong>and</strong> let M be a model category. Objective: Define a model<br />
category on M D .<br />
Let G, F : D → M be two functors. A natural transformation h: G → F is a morphism in<br />
M D . Say that h is a levelwise weak equivalence (or just a weak equivalence) if for all d ∈ D,<br />
the morphism h d : G(d) → F (d) is a weak equivalence. Define levelwise fibration <strong>and</strong> levelwise<br />
cofibration similarly.<br />
20<br />
□<br />
□