alternative lecture notes - Rational points and algebraic cycles

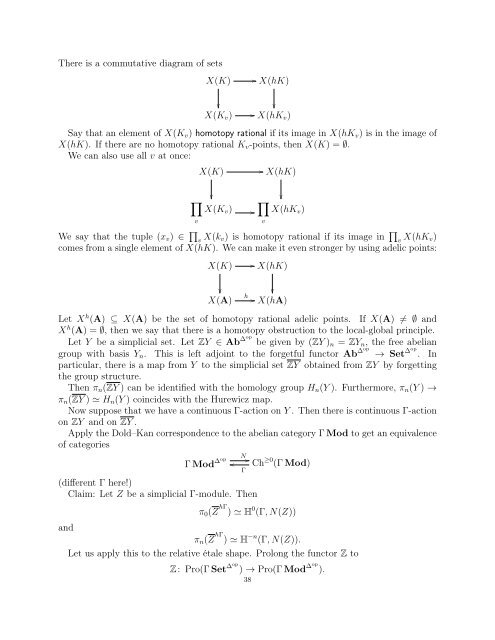
There is a commutative diagram of sets
X(K)
X(hK)
X(K v ) X(hK v )
Say that an element of X(K v ) homotopy rational if its image in X(hK v ) is in the image of
X(hK). If there are no homotopy rational K v -points, then X(K) = ∅.
We can also use all v at once:
X(K)
∏
X(K v )
v
X(hK)
∏
X(hK v )
We say that the tuple (x v ) ∈ ∏ v X(k v) is homotopy rational if its image in ∏ v X(hK v)
comes from a single element of X(hK). We can make it even stronger by using adelic points:
X(K)
v
X(hK)
X(A)
h
X(hA)
Let X h (A) ⊆ X(A) be the set of homotopy rational adelic points. If X(A) ≠ ∅ and
X h (A) = ∅, then we say that there is a homotopy obstruction to the local-global principle.
Let Y be a simplicial set. Let ZY ∈ Ab ∆op
be given by (ZY ) n = ZY n , the free abelian
group with basis Y n . This is left adjoint to the forgetful functor Ab ∆op → Set ∆op . In
particular, there is a map from Y to the simplicial set ZY obtained from ZY by forgetting
the group structure.
Then π n (ZY ) can be identified with the homology group H n (Y ). Furthermore, π n (Y ) →
π n (ZY ) ≃ H n (Y ) coincides with the Hurewicz map.
Now suppose that we have a continuous Γ-action on Y . Then there is continuous Γ-action
on ZY and on ZY .
Apply the Dold–Kan correspondence to the abelian category Γ Mod to get an equivalence
of categories
Γ Mod N ∆op Ch ≥0 (Γ Mod)
Γ
(different Γ here!)
Claim: Let Z be a simplicial Γ-module. Then
π 0 (Z hΓ ) ≃ H 0 (Γ, N(Z))
and
π n (Z hΓ ) ≃ H −n (Γ, N(Z)).
Let us apply this to the relative étale shape. Prolong the functor Z to
Z: Pro(Γ Set ∆op ) → Pro(Γ Mod ∆op ).
38