LECTURE 3: Polynomial interpolation and numerical differentiation
LECTURE 3: Polynomial interpolation and numerical differentiation
LECTURE 3: Polynomial interpolation and numerical differentiation
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
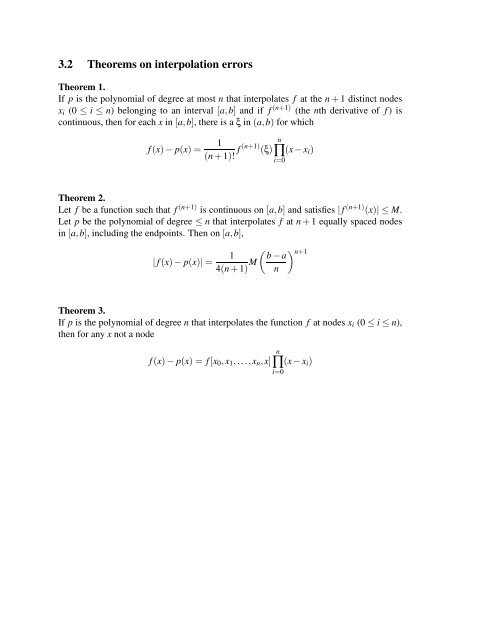
3.2 Theorems on <strong>interpolation</strong> errors<br />
Theorem 1.<br />
If p is the polynomial of degree at most n that interpolates f at the n+1 distinct nodes<br />
x i (0 ≤ i ≤ n) belonging to an interval [a,b] <strong>and</strong> if f (n+1) (the nth derivative of f ) is<br />
continuous, then for each x in [a,b], there is a ξ in(a,b) for which<br />
1<br />
f(x)− p(x)=<br />
(n+1)! f(n+1) (ξ)<br />
n<br />
∏<br />
i=0<br />
(x−x i )<br />
Theorem 2.<br />
Let f be a function such that f (n+1) is continuous on [a,b] <strong>and</strong> satisfies | f (n+1) (x)|≤M.<br />
Let p be the polynomial of degree ≤ n that interpolates f at n+1 equally spaced nodes<br />
in[a,b], including the endpoints. Then on[a,b],<br />
| f(x)− p(x)|=<br />
( )<br />
1 b−a n+1<br />
4(n+1) M n<br />
Theorem 3.<br />
If p is the polynomial of degree n that interpolates the function f at nodes x i (0≤i≤n),<br />
then for any x not a node<br />
f(x)− p(x)= f[x 0 ,x 1 ,...,x n ,x]<br />
n<br />
∏<br />
i=0<br />
(x−x i )