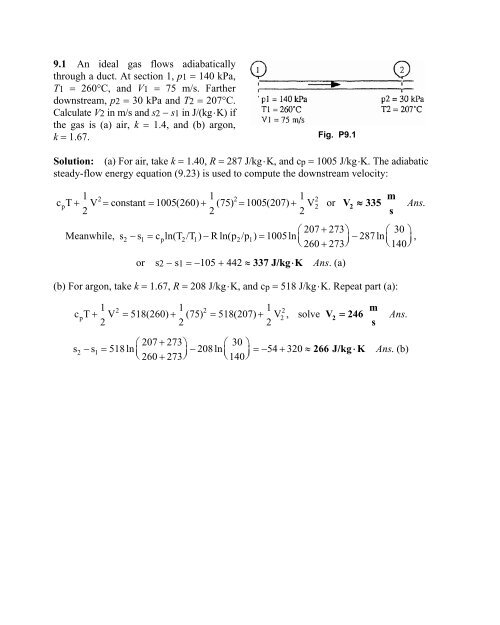
9.1 An ideal gas flows adiabatically through a duct. At section 1, p1 ...

9.1 An ideal gas flows adiabatically
through a duct. At section 1, p1 = 140 kPa,
T1 = 260°C, and V1 = 75 m/s. Farther
downstream, p2 = 30 kPa and T2 = 207°C.
Calculate V2 in m/s and s2 − s1 in J/(kg ⋅K)
if
the gas is (a) air, k = 1.4, and (b) argon,
k = 1.67.
Fig. P9.1
Solution: (a) For air, take k = 1.40, R = 287 J/kg ⋅ K, and cp = 1005 J/kg ⋅K. The adiabatic
steady-flow energy equation (9.23) is used to compute the downstream velocity:
1 2 1 2 1 2
m
cpT + V = constant = 1005(260) + (75) = 1005(207) + V2 or V2 ≈ 335 Ans.
2 2 2
s
⎛207 + 273⎞ ⎛ 30 ⎞
Meanwhile, s2 − s1 = cpln(T 2/T 1) − R ln(p 2/p 1)
= 1005ln ⎜ −287
ln ,
⎝
⎟ ⎜
260 + 273⎠ ⎝
⎟
140⎠
or s2 − s1 = −105 + 442 ≈ 337 J/kg ⋅ K Ans. (a)
(b) For argon, take k = 1.67, R = 208 J/kg ⋅ K, and cp = 518 J/kg ⋅ K. Repeat part (a):
1 2 1 2 1 2
m
cpT+ V = 518(260) + (75) = 518(207) + V 2,
solve V2 = 246 Ans.
2 2 2
s
⎛207 + 273⎞ ⎛ 30 ⎞
s2 − s1 = 518 ln ⎜ ⎟ − 208 ln ⎜ ⎟ = − 54 + 320 ≈ 266 J/kg ⋅ K
Ans.
(b)
⎝260 + 273⎠ ⎝140⎠

P9.7 Air flows through a variable-area duct. At section 1, A1 = 20 cm 2 , p1 = 300 kPa, ρ1 =
1.75 kg/m 3 , and V1 = 122.5 m/s. At section 2, the area is exactly the same, but the density is
much lower: ρ2 = 0.266 kg/m 3 , and T2 = 281 K. There is no transfer of work or heat. Assume
one-dimensional steady flow. (a) How can you reconcile these differences? (b) Find the mass
flow at section 2. Calculate (c) V2, (d) p2, and (e) s2 – s1. Hint: This problem requires the
continuity equation.
Solution: Part (a) is too confusing, let’s try (b, c, d, e) first. (b) The mass flow must be
constant:
m�
1
Then
= m�
V
2
2
= ρ A V
=
1
2
1
m�
ρ A
1
2
kg
2 m
= ( 1.
75 )( 0.
0020m
)( 122.
5 )
3
m
s
=
kg
0.
0429
s
=
0.
0429kg
/ s
3
2
( 0.
266kg
/ m )( 0.
002m
)
=
m
806
s
Ans.(
c)
1/2 1/2
Ans.(
b)
That’s pretty fast! Check a 2 = (kRT 2) = [1.4(287)(281)] = 336 m/s. Hence the Mach
number at section 2 is Ma 2 = V2/a2 = 806/336 = 2.40. The flow at section 2 is supersonic!
(d) The pressure at section 2 is easy, since the density and temperature are given:
p
2
= ρ RT
2
Similarly,
T
2
1
= ( 0.
266kg
/ m )( 287 m / s − K)(
281K
) = 21,
450 Pa
=
p1
Rρ
=
( 300000 Pa)
2
3
( 287 m / s − K)(
1.
75kg
/ m )
= 597 K
1
3
2
2
2
Ans(
d)
(e) Finally, with pressures and temperatures known, the entropy change follows from Eq. (9.8):
T2
p2
281 21450
J
s2 − s1
= c p ln( ) − R ln( ) = 1005ln(
) − 287 ln( ) = − 757 + 757 ≈ 0
T p
597 300000
kg − K
1
1
Ahah! Now I get it. (a) The flow is isentropic. Ans.(a) The stagnation properties, T = 605 K,
o
3
p o = 319 kPa, and ρ o = 1.805 kg/m are constant in the flow from section 1 to section 2.
Ans.(
e)

9.8 Atmospheric air at 20°C enters and
fills an insulated tank which is initially
evacuated. Using a control-volume analysis
from Eq. (3.63), compute the tank air
temperature when it is full.
Solution: The energy equation during filling of the adiabatic tank is
dQ dWshaft dECV
+ = 0 + 0 = −hatmm � entering,
or, after filling,
dt dt dt
ECV,final − ECV,initial = hatmm entered, or: mcvTtank = mcpT
atm
Thus T = (c /c )T = (1.4)(20 + 273) ≈ 410 K = 137° C Ans.
tank
p v atm