Chaos and quasi-periodicity in diffeomorphisms of the solid torus
Chaos and quasi-periodicity in diffeomorphisms of the solid torus
Chaos and quasi-periodicity in diffeomorphisms of the solid torus
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
PSfrag replacements<br />
p<br />
U ′<br />
∂ s<br />
q<br />
c<br />
∂ u<br />
d<br />
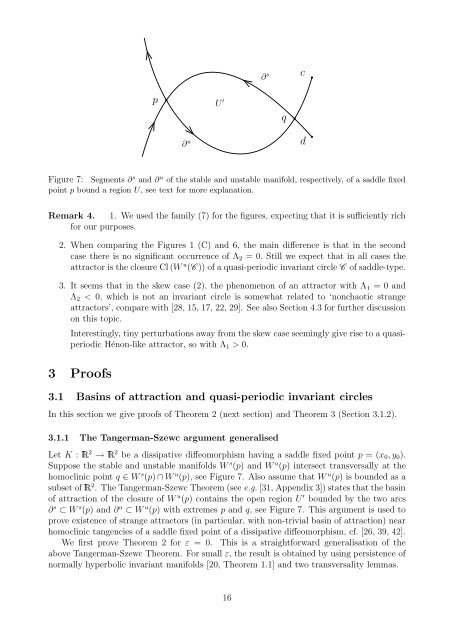
Figure 7: Segments ∂ s <strong>and</strong> ∂ u <strong>of</strong> <strong>the</strong> stable <strong>and</strong> unstable manifold, respectively, <strong>of</strong> a saddle fixed<br />
po<strong>in</strong>t p bound a region U, see text for more explanation.<br />
Remark 4. 1. We used <strong>the</strong> family (7) for <strong>the</strong> figures, expect<strong>in</strong>g that it is sufficiently rich<br />
for our purposes.<br />
2. When compar<strong>in</strong>g <strong>the</strong> Figures 1 (C) <strong>and</strong> 6, <strong>the</strong> ma<strong>in</strong> difference is that <strong>in</strong> <strong>the</strong> second<br />
case <strong>the</strong>re is no significant occurrence <strong>of</strong> Λ 2 = 0. Still we expect that <strong>in</strong> all cases <strong>the</strong><br />
attractor is <strong>the</strong> closure Cl (W u (C )) <strong>of</strong> a <strong>quasi</strong>-periodic <strong>in</strong>variant circle C <strong>of</strong> saddle-type.<br />
3. It seems that <strong>in</strong> <strong>the</strong> skew case (2), <strong>the</strong> phenomenon <strong>of</strong> an attractor with Λ 1 = 0 <strong>and</strong><br />
Λ 2 < 0, which is not an <strong>in</strong>variant circle is somewhat related to ‘nonchaotic strange<br />
attractors’, compare with [28, 15, 17, 22, 29]. See also Section 4.3 for fur<strong>the</strong>r discussion<br />
on this topic.<br />
Interest<strong>in</strong>gly, t<strong>in</strong>y perturbations away from <strong>the</strong> skew case seem<strong>in</strong>gly give rise to a <strong>quasi</strong>periodic<br />
Hénon-like attractor, so with Λ 1 > 0.<br />
3 Pro<strong>of</strong>s<br />
3.1 Bas<strong>in</strong>s <strong>of</strong> attraction <strong>and</strong> <strong>quasi</strong>-periodic <strong>in</strong>variant circles<br />
In this section we give pro<strong>of</strong>s <strong>of</strong> Theorem 2 (next section) <strong>and</strong> Theorem 3 (Section 3.1.2).<br />
3.1.1 The Tangerman-Szewc argument generalised<br />
Let K : 2 → 2 be a dissipative diffeomorphism hav<strong>in</strong>g a saddle fixed po<strong>in</strong>t p = (x 0 , y 0 ).<br />
Suppose <strong>the</strong> stable <strong>and</strong> unstable manifolds W s (p) <strong>and</strong> W u (p) <strong>in</strong>tersect transversally at <strong>the</strong><br />
homocl<strong>in</strong>ic po<strong>in</strong>t q ∈ W s (p) ∩ W u (p), see Figure 7. Also assume that W u (p) is bounded as a<br />
subset <strong>of</strong><br />
2 . The Tangerman-Szewc Theorem (see e.g. [31, Appendix 3]) states that <strong>the</strong> bas<strong>in</strong><br />
<strong>of</strong> attraction <strong>of</strong> <strong>the</strong> closure <strong>of</strong> W u (p) conta<strong>in</strong>s <strong>the</strong> open region U ′ bounded by <strong>the</strong> two arcs<br />
∂ s ⊂ W s (p) <strong>and</strong> ∂ u ⊂ W u (p) with extremes p <strong>and</strong> q, see Figure 7. This argument is used to<br />
prove existence <strong>of</strong> strange attractors (<strong>in</strong> particular, with non-trivial bas<strong>in</strong> <strong>of</strong> attraction) near<br />
homocl<strong>in</strong>ic tangencies <strong>of</strong> a saddle fixed po<strong>in</strong>t <strong>of</strong> a dissipative diffeomorphism, cf. [26, 39, 42].<br />
We first prove Theorem 2 for ε = 0. This is a straightforward generalisation <strong>of</strong> <strong>the</strong><br />
above Tangerman-Szewc Theorem. For small ε, <strong>the</strong> result is obta<strong>in</strong>ed by us<strong>in</strong>g persistence <strong>of</strong><br />
normally hyperbolic <strong>in</strong>variant manifolds [20, Theorem 1.1] <strong>and</strong> two transversality lemmas.<br />
16