(1985). Levine's Atlas of Corporate Interlocks. Connections ... - INSNA

I
I
I
I
I
1 0 3
Moon-shifting the ten points for the corporations
results in the somewhat changed map in Figure 7 . The corporations
ore now centered with respect to their directors
but the directors are not yet centered with respect
to the corporations . Mean-shifting the fifteen points for
the directors corrects this problem . But now, unfortunately,
the problem Is reversed . American Airlines is
properly centered with respect to the previous locutions
of John Leitch and William Beers, but they themselves
hove been moved to their own centered positions .
Still this is not the solution, although the configuration
is relatively clear . It can be proven that by
simply repeating this procedure, one map after another,
again and again, the maps converge to a "stabile" map .I3)
It is stabile in the sense that if it is subjected to
any more averaging (any more movement of the corporations
and directors), nothing happens : The map gets "smaller"
(the outer points, and therefore all other points, keep
moving toward the center) but the relative locations
stay constant . This is the centroid solution .
Computing moons once again produces a third set of
locations, now twice-removed from the original drawing .
Repeating this process . again and again, gets closer to o
solution . Continuing for ten steps, ten steps removed
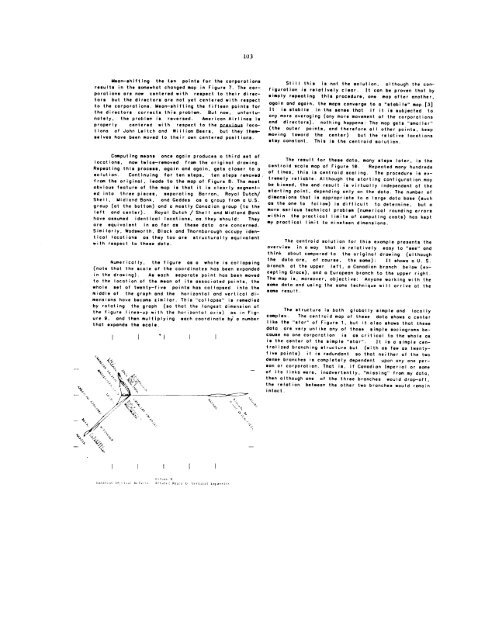
from the original, leads to the map of Figure 8 . The most
obvious feature of the map is that it is clearly segmented
into three pieces, separating Barron, Royal Dutch/
Shell . Midland Bank, and Geddes as a group from a U .S .
group (at the bottom) and a mostly Canadian group (to the
left and center) . Royal Dutch / Shell and Midland Son k
have assumed identical locations, as they should : They
are equivalent in so for as these data ore concerned .
Similarly, Wadsworth, Block and Thornborough occupy identical
locations am they too ore structurally equivalent
with respect to these data .
Numerically, the figure as a whole is collapsing
(note that the scale of the coordinates has been expanded
in the drawing) . As each separate point has been moved
to the location of the mean of its associated points, the
whole set of twenty-five points has collapsed into the
middle of the graph and the horizontal and vertical dimensions
hove become similar . This "collapse" is remedied
by rotating the graph (so that the longest dimension of
the figure lines-up with the horizontal axis) as in Figure
9, and then multiplying each coordinate by% a number
that expands the scale .
The result for these data, many steps later, is the
centroid scale map of Figure 10 . Repeated many hundreds
of times, this is centroid scaling . The procedure is extremely
reliable . Although the starting configuration may
be biased . the end result is virtually independent of the
starting point, depending only on the data . The number of
dimensions that is appropriate to a large data base (such
as the one to follow) is difficult to determine, but a
more serious technical problem (numerical rounding errors
within the practical limits of computing costs) has kept
my practical limit to nineteen dimensions .
The centroid solution for this example presents the
overview in a way that is relatively easy to "see" and
think about compared to the original drawing (although
the data are, of course, the some) : It shows a U . S .
branch at the upper left, a Canadian branch below (excepting
Grace), and a European branch to the upper right .
The mop is, moreover, objective : Anyone working with the
same data and using the some technique will arrive at the
same result .
The structure is both globally simple and locally
complex . The centroid mop of these data shows a center
like the "star" of Figure 1, but it also shows that these
data are very unlike any of those simple sociogroms because
no one corporation is as critical to the whole as
is the center of the simple "star" . It is a simple centralized
branching structure but (with as few as twentyfive
points) it is redundant so that neither of the two
dense branches is completely dependent upon any one person
or corporation . That is, if Canadian Imperial or some
of its links were, inadvertently, "missing" from my data,
then although one of the three branches would drop-off,
the relation between the other two branches would remain
intact .