Chapter 4 Trigonometric Functions
Chapter 4 Trigonometric Functions
Chapter 4 Trigonometric Functions
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Chapter</strong> 4<br />
<strong>Trigonometric</strong> <strong>Functions</strong><br />
Section 4.1<br />
Check Point Exercises<br />
1. The radian measure of a central angle is the length of<br />
the intercepted arc, s, divided by the circle’s radius, r.<br />
The length of the intercepted arc is 42 feet: s = 42<br />
feet. The circle’s radius is 12 feet: r = 12 feet. Now<br />
use the formula for radian measure to find the radian<br />
measure of θ .<br />
s 42 feet<br />
θ = = = 3.5<br />
r 12 feet<br />
Thus, the radian measure of θ is 3.5<br />
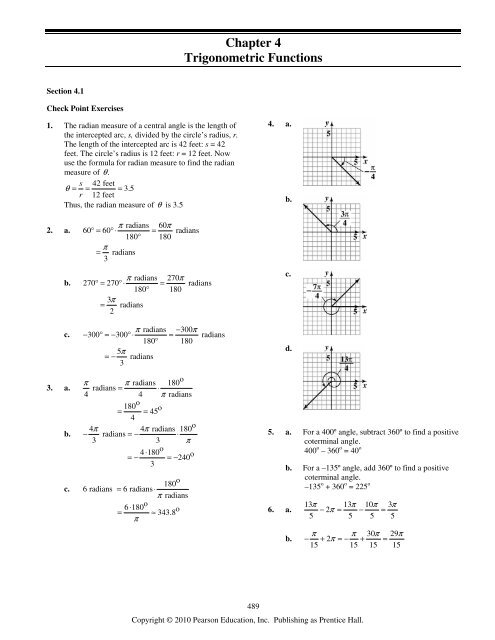
4. a.<br />
b.<br />
2. a.<br />
b.<br />
c.<br />
3. a.<br />
b.<br />
c.<br />
π radians 60π<br />
60°= 60 °⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
3<br />
π radians 270π<br />
270°= 270 °⋅ = radians<br />
180°<br />
180<br />
3π<br />
= radians<br />
2<br />
π radians −300π<br />
− 300°= − 300 °⋅ = radians<br />
180°<br />
180<br />
5π<br />
=− radians<br />
3<br />
π π radians 180<br />
o<br />
radians = ⋅<br />
4<br />
4 π radians<br />
180<br />
o<br />
= = 45<br />
o<br />
4<br />
4π<br />
4 π radians 180<br />
o<br />
− radians = − ⋅<br />
3 3 π<br />
4180 ⋅<br />
o<br />
=− =− 240<br />
o<br />
3<br />
180<br />
o<br />
6 radians = 6 radians ⋅<br />
π radians<br />
6⋅180 o<br />
= ≈ 343.8<br />
o<br />
π<br />
c.<br />
d.<br />
5. a. For a 400º angle, subtract 360º to find a positive<br />
coterminal angle.<br />
400 o – 360 o = 40 o<br />
6. a.<br />
b. For a –135º angle, add 360º to find a positive<br />
coterminal angle.<br />
–135 o + 360 o = 225 o<br />
b.<br />
13 π 13 10 3<br />
− 2π<br />
= π − π =<br />
π<br />
5 5 5 5<br />
30 29<br />
− π + 2π<br />
= − π + π =<br />
π<br />
15 15 15 15<br />
489<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
7. a. 855°− 360°⋅ 2 = 855°− 720°= 135°<br />
b.<br />
c.<br />
17π<br />
17π<br />
−2π<br />
⋅ 2= − 4π<br />
3 3<br />
17π 12π 5π<br />
= − =<br />
3 3 3<br />
25π<br />
25π<br />
− + 2π<br />
⋅ 3=− + 6π<br />
6 6<br />
25π 36π 11π<br />
=− + =<br />
6 6 6<br />
8. The formula s = rθ can only be used when θ is<br />
expressed in radians. Thus, we begin by converting<br />
π radians<br />
45° to radians. Multiply by .<br />
180°<br />
π radians 45<br />
45°= 45 °⋅ = π radians<br />
180°<br />
180<br />
π<br />
= radians<br />
4<br />
Now we can use the formula s = rθ to find the<br />
length of the arc. The circle’s radius is 6 inches : r =<br />
6 inches. The measure of the central angle in radians<br />
π π<br />
is : θ = . The length of the arc intercepted by this<br />
4 4<br />
central angle is<br />
⎛π<br />
⎞ 6π<br />
s = rθ<br />
= (6 inches) ⎜ ⎟= inches ≈ 4.71 inches.<br />
⎝ 4⎠<br />
4<br />
9. We are given ω , the angular speed.<br />
ω = 45 revolutions per minute<br />
We use the formula ν = rω<br />
to find v , the linear<br />
speed. Before applying the formula, we must express<br />
ω in radians per minute.<br />
45 revolutions 2 π radians<br />
ω = ⋅<br />
1 minute 1 revolution<br />
90 π radians<br />
=<br />
1 minute<br />
The angular speed of the propeller is 90π radians per<br />
minute. The linear speed is<br />
90π<br />
135 π inches<br />
ν = rω<br />
= 1.5 inches ⋅ = 1 minute minute<br />
The linear speed is 135π inches per minute, which is<br />
approximately 424 inches per minute.<br />
Exercise Set 4.1<br />
1. obtuse<br />
2. obtuse<br />
3. acute<br />
4. acute<br />
5. straight<br />
6. right<br />
7.<br />
8.<br />
9.<br />
10.<br />
11.<br />
12.<br />
13.<br />
14.<br />
θ = s 40 inches<br />
4 radians<br />
r<br />
= 10 inches<br />
=<br />
θ = s 30 feet<br />
6 radians<br />
r<br />
= 5 feet<br />
=<br />
s 8 yards 4<br />
θ = = = radians<br />
r 6 yards 3<br />
θ = s 18 yards<br />
2.25 radians<br />
r<br />
= 8 yards<br />
=<br />
θ = s 400 centimeters<br />
4 radians<br />
r<br />
= 100 centimeters<br />
=<br />
θ = s 600 centimeters<br />
6 radians<br />
r<br />
= 100 centimeters<br />
=<br />
π radians<br />
45°= 45°⋅<br />
180°<br />
45π<br />
= radians<br />
180<br />
π<br />
= radians<br />
4<br />
π radians<br />
18°= 18°⋅<br />
180°<br />
18π<br />
= radians<br />
180<br />
π<br />
= radians<br />
10<br />
15.<br />
π radians<br />
135°= 135°⋅<br />
180°<br />
135π<br />
= radians<br />
180<br />
3π<br />
= radians<br />
4<br />
490<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.1<br />
16.<br />
17.<br />
18.<br />
19.<br />
20.<br />
21.<br />
22.<br />
π radians<br />
150°= 150°⋅<br />
180°<br />
150π<br />
= radians<br />
180<br />
5π<br />
= radians<br />
6<br />
π radians<br />
300°= 300°⋅<br />
180°<br />
300π<br />
= radians<br />
180<br />
5π<br />
= radians<br />
3<br />
π radians<br />
330°= 330°⋅<br />
180°<br />
330π<br />
= radians<br />
180<br />
11π<br />
= radians<br />
6<br />
π radians<br />
− 225°= − 225°⋅<br />
180°<br />
225π<br />
=− radians<br />
180<br />
5π<br />
=− radians<br />
4<br />
π radians<br />
− 270°= − 270°⋅<br />
180°<br />
270π<br />
=− radians<br />
180<br />
3π<br />
=− radians<br />
2<br />
π π radians 180<br />
o<br />
radians = ⋅<br />
2 2 π radians<br />
180<br />
o<br />
=<br />
2<br />
= 90 o<br />
π π radians 180<br />
o<br />
radians = ⋅<br />
9 9 π radians<br />
180<br />
o<br />
= = 20<br />
o<br />
9<br />
23.<br />
24.<br />
25.<br />
26.<br />
27.<br />
28.<br />
29.<br />
30.<br />
31.<br />
2π<br />
2 π radians 180<br />
o<br />
radians = ⋅<br />
3 3 π radians<br />
2180 ⋅<br />
o<br />
=<br />
3<br />
= 120 o<br />
3 π radians 180<br />
0<br />
3⋅180 o<br />
⋅ = = 135<br />
o<br />
4 π radians 4<br />
7π<br />
7 π radians 180<br />
o<br />
radians = ⋅<br />
6 6 π radians<br />
7180 ⋅<br />
o<br />
=<br />
6<br />
= 210 o<br />
11 π radians 180<br />
o<br />
11⋅180 o<br />
⋅ = = 330<br />
o<br />
6 π radians 6<br />
180<br />
o<br />
− 3 π radians = −3 π radians ⋅<br />
π radians<br />
=−3180<br />
⋅<br />
o<br />
=−540<br />
o<br />
180<br />
o<br />
−4 π radians ⋅ = −4 ⋅ 180<br />
o<br />
=− 720<br />
o<br />
π radians<br />
π radians<br />
18°= 18°⋅<br />
180°<br />
18π<br />
= radians<br />
180<br />
≈ 0.31 radians<br />
π radians<br />
76°= 76°⋅<br />
180°<br />
76π<br />
= radians<br />
180<br />
≈ 1.33 radians<br />
π radians<br />
− 40°=− 40°⋅<br />
180°<br />
40π<br />
=− radians<br />
180<br />
≈−0.70 radians<br />
491<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
32.<br />
33.<br />
34.<br />
35.<br />
36.<br />
π radians<br />
− 50°=− 50°⋅<br />
180°<br />
50π<br />
=− radians<br />
180<br />
≈−0.87 radians<br />
π radians<br />
200°= 200°⋅<br />
180°<br />
200π<br />
= radians<br />
180<br />
≈ 3.49 radians<br />
π radians<br />
250°= 250°⋅<br />
180°<br />
250π<br />
= radians<br />
180<br />
≈ 4.36 radians<br />
180<br />
o<br />
2 radians = 2 radians ⋅<br />
π radians<br />
2180 ⋅<br />
o<br />
=<br />
π<br />
≈ 114.59<br />
o<br />
180<br />
o<br />
3⋅180 o<br />
3 radians ⋅ = ≈ 171.89<br />
o<br />
π radians π<br />
41.<br />
42.<br />
43.<br />
44.<br />
37.<br />
38.<br />
39.<br />
40.<br />
π π radians 180<br />
o<br />
radians = ⋅<br />
13 13 π radians<br />
180<br />
o<br />
=<br />
13<br />
≈ 13.85 o<br />
π 180<br />
o<br />
180<br />
o<br />
radians ⋅ = ≈ 10.59<br />
o<br />
17 π radians 17<br />
180<br />
o<br />
− 4.8 radians =−4.8 radians ⋅<br />
π radians<br />
−4.8⋅180<br />
o<br />
=<br />
π<br />
≈−275.02<br />
o<br />
180<br />
o<br />
−5.2⋅180<br />
o<br />
−5.2 radians⋅ =<br />
πradians<br />
π<br />
≈− 297.94<br />
o<br />
45.<br />
46.<br />
47.<br />
492<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.1<br />
48.<br />
55.<br />
49.<br />
56.<br />
50.<br />
57. 395°− 360°= 35°<br />
58. 415°− 360°= 55°<br />
59. − 150°+ 360°= 210°<br />
51.<br />
60. − 160°+ 360°= 200°<br />
61. − 765°+ 360°⋅ 3 =− 765°+ 1080°= 315°<br />
62. − 760°+ 360°⋅ 3 =− 760°+ 1080°= 320°<br />
52.<br />
53.<br />
54.<br />
63.<br />
64.<br />
65.<br />
66.<br />
67.<br />
68.<br />
69.<br />
19 π 19 12 7<br />
− 2π<br />
= π − π =<br />
π<br />
6 6 6 6<br />
17 π 17 10 7<br />
− 2π<br />
= π − π =<br />
π<br />
5 5 5 5<br />
23 π 23 23 20 3<br />
−2π<br />
⋅ 2= π − 4π<br />
= π − π =<br />
π<br />
5 5 5 5 5<br />
25 π 25 25 24<br />
−2π<br />
⋅ 2= π − 4π<br />
= π − π =<br />
π<br />
6 6 6 6 6<br />
100 99<br />
− π + 2π<br />
=− π + π =<br />
π<br />
50 50 50 50<br />
80 79<br />
− π + 2π<br />
= − π + π =<br />
π<br />
40 40 40 40<br />
31π<br />
31π<br />
− + 2π<br />
⋅ 3=− + 6π<br />
7 7<br />
31π 42π 11π<br />
=− + =<br />
7 7 7<br />
493<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
38π<br />
38π<br />
70. − + 2π<br />
⋅ 3= − + 6π<br />
9 9<br />
38π 54π 16π<br />
=− + =<br />
9 9 9<br />
71. r = 12 inches, θ = 45°<br />
Begin by converting 45° to radians, in order to use<br />
the formula s = rθ .<br />
π radians π<br />
45°= 45°⋅ = radians<br />
180°<br />
4<br />
Now use the formula s = rθ .<br />
π<br />
s = rθ<br />
= 12⋅ = 3π<br />
inches ≈ 9.42 inches<br />
4<br />
72. r = 16 inches, θ = 60°<br />
Begin by converting 60° to radians, in order to use<br />
the formula s = rθ .<br />
π radians π<br />
60°= 60°⋅ = radians<br />
180°<br />
3<br />
Now use the formula s = rθ .<br />
π 16π<br />
s = rθ<br />
= 16 ⋅ = inches ≈ 16.76 inches<br />
3 3<br />
73. r = 8feet, θ = 225°<br />
Begin by converting 225° to radians, in order to use<br />
the formula s = rθ .<br />
π radians 5π<br />
225°= 225°⋅ = radians<br />
180°<br />
4<br />
Now use the formula s = rθ .<br />
5π<br />
s = rθ<br />
= 8⋅ = 10π<br />
feet ≈ 31.42 feet<br />
4<br />
74. r = 9 yards, θ = 315°<br />
Begin by converting 315° to radians, in order to use<br />
the formula s = rθ .<br />
π radians 7π<br />
315°= 315°⋅ = radians<br />
180°<br />
4<br />
Now use the formula s = rθ .<br />
7π<br />
63π<br />
s = rθ<br />
= 9 ⋅ = yards ≈ 49.48 yards<br />
4 4<br />
75. 6 revolutions per second<br />
6 revolutions 2 π radians 12 π radians<br />
= ⋅ =<br />
1 second 1 revolutions 1 seconds<br />
= 12 π radians per second<br />
77.<br />
78.<br />
79.<br />
80.<br />
81.<br />
4π<br />
2π<br />
− and<br />
3 3<br />
7π<br />
5π<br />
− and<br />
6 6<br />
3π<br />
5π<br />
− and<br />
4 4<br />
π 7π<br />
− and<br />
4 4<br />
π 3π<br />
− and<br />
2 2<br />
82. −π and π<br />
83.<br />
84.<br />
55 11π<br />
⋅ 2π<br />
=<br />
60 6<br />
35 7π<br />
⋅ 2π<br />
=<br />
60 6<br />
85. 3 minutes and 40 seconds equals 220 seconds.<br />
220 22π<br />
⋅ 2π<br />
=<br />
60 3<br />
86. 4 minutes and 25 seconds equals 265 seconds.<br />
265 53π<br />
⋅ 2π<br />
=<br />
60 6<br />
87. First, convert to degrees.<br />
1 1 360°<br />
revolution = revolution ⋅<br />
6 6 1 revolution<br />
1<br />
= ⋅ 360 °= 60 °<br />
6<br />
Now, convert 60° to radians.<br />
π radians 60π<br />
60°= 60°⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
3<br />
Therefore, 1 6 revolution is equivalent to 60° or π<br />
3<br />
radians.<br />
76. 20 revolutions per second<br />
20 revolutions 2 π radians 40 π radians<br />
= ⋅ =<br />
1 second 1 revolution 1 second<br />
= 40 π radians per second<br />
494<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.1<br />
88. First, convert to degrees.<br />
1 1 360<br />
o<br />
revolutions = revolutions ⋅<br />
3 3 1 revolution<br />
1<br />
= ⋅ 360<br />
o<br />
= 120<br />
o<br />
3<br />
Now, convert 120° to radians.<br />
π radians 120π<br />
120°= 120°⋅ = radians<br />
180°<br />
180<br />
2π<br />
= radians<br />
3<br />
Therefore, 1 3 revolution is equivalent to 120° or 2 π<br />
3<br />
radians.<br />
89. The distance that the tip of the minute hand moves is<br />
given by its arc length, s. Since s = rθ , we begin by<br />
finding r and θ . We are given that r = 8 inches. The<br />
minute hand moves from 12 to 2 o'clock, or 1 6 of a<br />
complete revolution. The formula s = rθ can only be<br />
used when θ is expressed in radians. We must<br />
convert 1 revolution to radians.<br />
6<br />
1 1 2 π radians<br />
revolution = revolution ⋅<br />
6 6 1 revolution<br />
π<br />
= radians<br />
3<br />
The distance the tip of the minute hand moves is<br />
⎛π<br />
⎞ 8π<br />
s = rθ<br />
= (8 inches) ⎜ ⎟=<br />
inches<br />
⎝ 3 ⎠ 3<br />
≈ 8.38 inches.<br />
90. The distance that the tip of the minute hand moves is<br />
given by its arc length, s. Since s = rθ , we begin by<br />
finding r and θ . We are given that<br />
r = 6 inches. The minute hand moves from 12 to 4<br />
o’clock, or 1 of a complete revolution. The formula<br />
3<br />
s = rθ can only be used when θ is expressed in<br />
radians. We must convert 1 revolution to radians.<br />
3<br />
1 1 2 π radians<br />
revolution = revolution ⋅<br />
3 3 1 revolution<br />
2π<br />
= radians<br />
3<br />
The distance the tip of the minute hand moves is<br />
⎛2π<br />
⎞ 12π<br />
s = rθ<br />
= (6 inches) ⎜ ⎟=<br />
inches<br />
⎝ 3 ⎠ 3<br />
= 4π<br />
inches ≈12.57 inches.<br />
91. The length of each arc is given by s = rθ . We are<br />
given that r = 24 inches and<br />
θ = 90 ° . The formula s = rθ can only be used when<br />
θ is expressed in radians.<br />
π radians 90π<br />
90°= 90°⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
2<br />
The length of each arc is<br />
⎛π<br />
⎞<br />
s = rθ<br />
= (24 inches) ⎜ ⎟=<br />
12π<br />
inches<br />
⎝ 2 ⎠<br />
≈ 37.70 inches.<br />
92. The distance that the wheel moves is given by<br />
s = rθ . We are given that r = 80 centimeters and θ<br />
= 60°. The formula s = rθ can only be used when θ<br />
is expressed in radians.<br />
π radians 60π<br />
60°= 60°⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
3<br />
The length that the wheel moves is<br />
⎛π<br />
⎞ 80π<br />
s = rθ<br />
= (80 centimeters) ⎜ ⎟ = centimeters<br />
⎝ 3⎠<br />
3<br />
≈ 83.78 centimeters.<br />
s<br />
93. Recall that θ = . We are given that<br />
r<br />
s = 8000 miles and r = 4000 miles.<br />
s 8000 miles<br />
θ = = = 2 radians<br />
r 4000 miles<br />
Now, convert 2 radians to degrees.<br />
180<br />
o<br />
2 radians = 2 radians ⋅ ≈ 114.59<br />
o<br />
π radians<br />
s<br />
94. Recall that θ = . We are given that<br />
r<br />
s = 10,000 miles and r = 4000 miles.<br />
s 10,000 miles<br />
θ = = = 2.5 radians<br />
r 4000 miles<br />
Now, convert 2.5 radians to degrees.<br />
180<br />
o<br />
2.5 radians⋅ ≈ 143.24<br />
o<br />
2 π radians<br />
495<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
95. Recall that s = rθ . We are given that<br />
r = 4000 miles and θ = 30°. The formula s = rθ can<br />
only be used when θ is expressed in radians.<br />
π radians 30π<br />
30°= 30°⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
6<br />
⎛π<br />
⎞<br />
s= rθ<br />
=(4000 miles) ⎜ ⎟ ≈ 2094 miles<br />
⎝ 6 ⎠<br />
To the nearest mile, the distance from A to B is<br />
2094 miles.<br />
96. Recall that s = rθ . We are given that<br />
r = 4000 miles and θ = 10°. We can only use the<br />
formula s = rθ when θ is expressed in radians.<br />
π radians 10π<br />
10°= 10°⋅ = radians<br />
180°<br />
180<br />
π<br />
= radians<br />
18<br />
⎛ π ⎞<br />
s= rθ<br />
=(4000 miles) ⎜ ⎟ ≈ 698 miles<br />
⎝18<br />
⎠<br />
To the nearest mile, the distance from A to B is<br />
698 miles.<br />
97. Linear speed is given by ν = rω<br />
. We are given that<br />
π<br />
ω = radians per hour and<br />
12<br />
r = 4000 miles. Therefore,<br />
⎛ π ⎞<br />
ν = rω<br />
= (4000 miles) ⎜ ⎟<br />
⎝ 12 ⎠<br />
4000π<br />
= miles per hour<br />
12<br />
≈ 1047 miles per hour<br />
The linear speed is about 1047 miles per hour.<br />
98. Linear speed is given by ν = rω<br />
. We are given that<br />
r = 25 feet and the wheel rotates at 3 revolutions per<br />
minute. We need to convert 3 revolutions per minute<br />
to radians per minute.<br />
3 revolutions per minute<br />
2π<br />
radians<br />
= 3 revolutions per minute ⋅ 1 revolution<br />
= 6π<br />
radians per minute<br />
ν = rω = (25 feet)(6 π) ≈ 471 feet per minute<br />
The linear speed of the Ferris wheel is about<br />
471 feet per minute.<br />
99. Linear speed is given by ν = rω<br />
. We are given that<br />
r = 12 feet and the wheel rotates at 20 revolutions per<br />
minute.<br />
20 revolutions per minute<br />
2π<br />
radians<br />
= 20 revolutions per minute⋅<br />
1 revolution<br />
= 40π<br />
radians per minute<br />
ν = rω = (12 feet)(40 π)<br />
≈ 1508 feet per minute<br />
The linear speed of the wheel is about<br />
1508 feet per minute.<br />
100. Begin by converting 2.5 revolutions per minute to<br />
radians per minute.<br />
2.5 revolutions per minute<br />
2π<br />
radians<br />
= 2.5 revolutions per minute ⋅ 1 revolution<br />
= 5π<br />
radians per minute<br />
The linear speed of the animals in the outer rows is<br />
ν = rω = (20 feet)(5 π) ≈ 100 feet per minute<br />
The linear speed of the animals in the inner rows is<br />
ν = rω = (10 feet)(5 π) ≈ 50 feet per minute<br />
The difference is 100π − 50π = 50π<br />
feet per minute<br />
or about 157.08 feet per minute.<br />
101. – 112. Answers may vary.<br />
113.<br />
114.<br />
115.<br />
116.<br />
496<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.2<br />
117. does not make sense; Explanations will vary. Sample<br />
explanation: Angles greater than π will exceed a<br />
straight angle.<br />
118. does not make sense; Explanations will vary. Sample<br />
explanation: It is possible for π to be used in an<br />
angle measured using degrees.<br />
119. makes sense<br />
120. does not make sense; Explanations will vary. Sample<br />
explanation: That will not be possible if the angle is a<br />
multiple of 2 π .<br />
121. A right angle measures 90° and<br />
π<br />
90<br />
≈ °= radians 1.57 radians.<br />
2<br />
3<br />
If θ = radians = 1.5 radians, θ is smaller than a<br />
2<br />
right angle.<br />
122. s = rθ<br />
Begin by changing θ = 20° to radians.<br />
π π<br />
20°= 20°⋅ = radians<br />
180°<br />
9<br />
π<br />
100= r 9<br />
900<br />
r = ≈ 286 miles<br />
π<br />
To the nearest mile, a radius of 286 miles should be<br />
used.<br />
123. s = rθ<br />
Begin by changing θ = 26° to radians.<br />
124.<br />
π 13π<br />
26°= 26°⋅ = radians<br />
180°<br />
90<br />
13π<br />
s=4000⋅<br />
90<br />
≈ 1815 miles<br />
To the nearest mile, Miami, Florida is 1815 miles<br />
north of the equator.<br />
125. domain: { x −1≤ x ≤ 1}<br />
or [ − 1,1]<br />
range: { y −1≤ y ≤ 1}<br />
or [ − 1,1]<br />
126.<br />
1 3<br />
x =− ; y =<br />
2 2<br />
1<br />
−<br />
x 2 1 1 3 3<br />
= = − =− =−<br />
y 3 3 3 3 3<br />
2<br />
Section 4.2<br />
Check Point Exercises<br />
1.<br />
P ⎛<br />
⎜<br />
⎝<br />
sin t<br />
3 1<br />
,<br />
2 2<br />
= y =<br />
⎞<br />
⎟<br />
⎠<br />
1<br />
2<br />
cost<br />
= x =<br />
3<br />
2<br />
1<br />
2<br />
3<br />
2<br />
y<br />
tan t = = =<br />
x<br />
1<br />
csct<br />
= = 2<br />
y<br />
1 2 3<br />
sect<br />
= =<br />
x 3<br />
cot t = x = 3<br />
y<br />
3<br />
3<br />
2. The point P on the unit circle that corresponds to<br />
t = π has coordinates (–1, 0). Use x = –1 and y = 0 to<br />
find the values of the trigonometric functions.<br />
sinπ<br />
= y = 0<br />
cosπ<br />
= x = −1<br />
y 0<br />
tanπ<br />
= = = 0<br />
x −1<br />
1 1<br />
secπ<br />
= = = −1<br />
x −1<br />
x −1<br />
cotπ<br />
= = = undefined<br />
y 0<br />
1 1<br />
cscπ<br />
= = = undefined<br />
y 0<br />
497<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
3.<br />
π ⎛ 1 1 ⎞<br />
t = , P⎜ , ⎟<br />
4 ⎝ 2 2 ⎠<br />
π 1<br />
csc = =<br />
4 y<br />
2<br />
π 1<br />
sec = =<br />
4 x<br />
2<br />
π x<br />
cot = =<br />
4 y<br />
= 1<br />
1<br />
y<br />
1<br />
2<br />
⎛ π ⎞ ⎛π<br />
⎞<br />
4. a. sec⎜− = sec = 2<br />
4<br />
⎟ ⎜<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
5.<br />
b.<br />
⎛ π ⎞ ⎛π<br />
⎞ 2<br />
sin ⎜− sin<br />
4<br />
⎟ =− ⎜ =−<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ 2<br />
2<br />
sinθ<br />
tanθ<br />
= =<br />
3<br />
cosθ<br />
5<br />
3<br />
2 3 2<br />
= ⋅ =<br />
3 5 5<br />
2 5 2 5<br />
= ⋅ =<br />
5 5 5<br />
1 1 3<br />
cscθ<br />
= = =<br />
sinθ<br />
2 2<br />
3<br />
1 1 3<br />
secθ<br />
= = =<br />
cosθ<br />
5 5<br />
3<br />
3 5 3 5<br />
= ⋅ =<br />
5 5 5<br />
1 1 5<br />
cotθ<br />
= = =<br />
tanθ<br />
2 2<br />
5<br />
6.<br />
7. a.<br />
1 π<br />
sin t = , 0 ≤ t <<br />
2 2<br />
2 2<br />
sin t+ cos t = 1<br />
2<br />
⎛1<br />
⎞ +<br />
2 =<br />
⎜ cos 1<br />
2<br />
⎟ t<br />
⎝ ⎠<br />
2 1<br />
cos t = 1−<br />
4<br />
3 3<br />
cost<br />
= =<br />
4 2<br />
π<br />
Because 0 ≤ t < , cos t is positive.<br />
2<br />
b.<br />
5π ⎛π ⎞ π<br />
cot = cot π cot 1<br />
4<br />
⎜ + = =<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
⎛ 9π<br />
⎞ ⎛ 9π<br />
⎞<br />
cos ⎜− cos 4π<br />
4<br />
⎟= ⎜− +<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
7π<br />
= cos 4<br />
=<br />
π<br />
8. a. sin ≈ 0.7071<br />
4<br />
2<br />
2<br />
b. csc 1.5 ≈ 1.0025<br />
Exercise Set 4.2<br />
1. The point P on the unit circle has coordinates<br />
⎛ 15 8 ⎞<br />
⎜−<br />
,<br />
17 17<br />
⎟<br />
⎝ ⎠ . Use 15 8<br />
x =− and y = to find the<br />
17 17<br />
values of the trigonometric functions.<br />
8<br />
sin t = y =<br />
17<br />
15<br />
cost<br />
= x = −<br />
17<br />
8<br />
y 17 8<br />
tan t = = = −<br />
15<br />
x − 15<br />
17<br />
1 17<br />
csct<br />
= =<br />
y 8<br />
1 17<br />
sect<br />
= = −<br />
x 15<br />
x 15<br />
cot t = = −<br />
y 8<br />
498<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.2<br />
2. The point P on the unit circle has coordinates<br />
⎛ 5 12⎞<br />
⎜−<br />
, −<br />
13 13<br />
⎟<br />
⎝ ⎠ Use 5 12<br />
x =− and y =− to find the<br />
13 13<br />
values of the trigonometric functions.<br />
12<br />
sin t = y = −<br />
13<br />
5<br />
cost<br />
= x =−<br />
13<br />
12<br />
y −<br />
13 12<br />
tan t = = =<br />
5<br />
x − 5<br />
13<br />
1 13<br />
csct<br />
= = −<br />
y 12<br />
1 13<br />
sect<br />
= =−<br />
x 5<br />
x 5<br />
cot t = =<br />
y 12<br />
3. The point P on the unit circle that corresponds to<br />
t = − π<br />
4 has coordinates ⎛ 2 2 ⎞<br />
, −<br />
. Use x =<br />
⎜ 2 2 ⎟<br />
⎝ ⎠<br />
2<br />
and y =− to find the values of the trigonometric<br />
2<br />
functions.<br />
sin t = y = −<br />
2<br />
2<br />
cost<br />
= x =<br />
2<br />
2<br />
2<br />
y −<br />
2<br />
tan t = =<br />
x 2<br />
2<br />
=−1<br />
1<br />
csct<br />
= = −<br />
y<br />
2<br />
1<br />
sect<br />
= =<br />
x<br />
2<br />
x<br />
cot t = = −1<br />
y<br />
2<br />
2<br />
4. The point P on the unit circle that corresponds to<br />
3π<br />
⎛ 2 2 ⎞<br />
t = has coordinates<br />
− , . Use<br />
4<br />
⎜ 2 2 ⎟<br />
⎝ ⎠<br />
5.<br />
6.<br />
7.<br />
8.<br />
9.<br />
10.<br />
11.<br />
x =−<br />
2 2<br />
and y = to find the values of the<br />
2 2<br />
trigonometric functions.<br />
sin t = y =<br />
2<br />
2<br />
cost<br />
= x =−<br />
2<br />
2<br />
2<br />
y 2<br />
tan t = =<br />
x 2<br />
−<br />
2<br />
=−1<br />
1<br />
csct<br />
= =<br />
y<br />
2<br />
1<br />
sect<br />
= = −<br />
x<br />
2<br />
x<br />
cot t = = −1<br />
y<br />
π 1<br />
sin = 6 2<br />
π 3<br />
sin = 3 2<br />
5π 3<br />
cos =− 6 2<br />
2π 1<br />
cos =− 3 2<br />
0<br />
tan π= = 0<br />
−1<br />
0<br />
tan 0 = = 0<br />
1<br />
7π 1<br />
csc = =− 2<br />
6 −<br />
1<br />
2<br />
12.<br />
13.<br />
4π<br />
1 −2 3<br />
csc = = 3 − 3<br />
3<br />
2<br />
3<br />
2<br />
11π 1 2 3<br />
sec = = 6 3<br />
499<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
14.<br />
5π 1<br />
sec = = 2<br />
3<br />
1<br />
2<br />
24. a.<br />
11π<br />
− 3<br />
tan = = −<br />
6 3<br />
1<br />
2<br />
3<br />
2<br />
15.<br />
16.<br />
17.<br />
18.<br />
19. a.<br />
3π sin =− 1<br />
2<br />
3π cos = 0<br />
2<br />
3π sec = undefined<br />
2<br />
3π tan = undefined<br />
2<br />
b.<br />
20. a.<br />
b.<br />
21. a.<br />
π 3<br />
cos = 6 2<br />
⎛ π ⎞ π 3<br />
cos⎜− cos<br />
6<br />
⎟ = =<br />
⎝ ⎠ 6 2<br />
π 1<br />
cos = 3 2<br />
⎛ π ⎞ π 1<br />
cos⎜− cos<br />
3<br />
⎟= =<br />
⎝ ⎠ 3 2<br />
5π 1<br />
sin = 6 2<br />
25.<br />
26.<br />
b.<br />
⎛ 11π<br />
⎞ 11π<br />
3<br />
tan ⎜− tan<br />
6<br />
⎟= − =<br />
⎝ ⎠ 6 3<br />
8 15<br />
sin t = , cost<br />
=<br />
17 17<br />
8<br />
17 8<br />
tan t = =<br />
15<br />
15<br />
17<br />
17<br />
csct<br />
=<br />
8<br />
17<br />
sect<br />
=<br />
15<br />
15<br />
cot t =<br />
8<br />
3 4<br />
sin t = , cos t =<br />
5 5<br />
3<br />
5 3<br />
tan t = =<br />
4<br />
4<br />
5<br />
5<br />
csct<br />
=<br />
3<br />
5<br />
sect<br />
=<br />
4<br />
4<br />
cot t =<br />
3<br />
b.<br />
22. a.<br />
b.<br />
23. a.<br />
⎛ 5π<br />
⎞ 5π<br />
1<br />
sin ⎜− sin<br />
6<br />
⎟ =− = −<br />
⎝ ⎠ 6 2<br />
2π 3<br />
sin = 3 2<br />
⎛ 2π<br />
⎞ 2π<br />
3<br />
sin ⎜− sin<br />
3<br />
⎟ =− =−<br />
⎝ ⎠ 3 2<br />
3<br />
2<br />
1<br />
2<br />
5π<br />
−<br />
tan = = − 3<br />
3<br />
27.<br />
1 2 2<br />
sin t = , cos t =<br />
3 3<br />
1<br />
3 2<br />
tan t = =<br />
2 2 4<br />
3<br />
csct<br />
= 3<br />
3 2<br />
sect<br />
=<br />
4<br />
cot t = 2 2<br />
b.<br />
⎛ 5π<br />
⎞ 5π<br />
tan ⎜− = − tan = 3<br />
3<br />
⎟<br />
⎝ ⎠ 3<br />
500<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.2<br />
28.<br />
29.<br />
30.<br />
2 5<br />
sin t = , cost<br />
=<br />
3 3<br />
2<br />
3 2 5<br />
tan t = =<br />
5<br />
5<br />
3<br />
3<br />
csct<br />
=<br />
2<br />
3 5<br />
sect<br />
=<br />
5<br />
5<br />
cot t =<br />
2<br />
6 π<br />
sin t = , 0 ≤ t <<br />
7 2<br />
2 2<br />
sin t+ cos t = 1<br />
2<br />
⎛6<br />
⎞ +<br />
2<br />
t =<br />
⎜ cos 1<br />
7<br />
⎟<br />
⎝ ⎠<br />
2 36<br />
cos t = 1−<br />
49<br />
13 13<br />
cost<br />
= =<br />
49 7<br />
π<br />
Because 0 ≤ t < , cost<br />
is positive.<br />
2<br />
7 π<br />
sin t = , 0 ≤ t <<br />
8 2<br />
2 2<br />
sin t+ cos t = 1<br />
2<br />
⎛7<br />
⎞ +<br />
2<br />
t =<br />
⎜<br />
8<br />
⎟<br />
⎝ ⎠<br />
cos 1<br />
2 49<br />
cos t = 1−<br />
64<br />
31.<br />
32.<br />
33.<br />
34.<br />
39 π<br />
sin t = , 0 ≤ t <<br />
8 2<br />
2 2<br />
sin t+ cos t = 1<br />
2<br />
⎛ 39 ⎞<br />
+<br />
2 =<br />
cos t 1<br />
⎜<br />
8 ⎟<br />
⎝ ⎠<br />
2 39<br />
cos t = 1−<br />
64<br />
25 5<br />
cost<br />
= =<br />
64 8<br />
π<br />
Because 0 ≤ t < , cost<br />
is positive.<br />
2<br />
21 π<br />
sin t = , 0 ≤ t <<br />
5 2<br />
2 2<br />
sin t+ cos t = 1<br />
2<br />
⎛ 21 ⎞<br />
+<br />
2 =<br />
cos t 1<br />
⎜<br />
5 ⎟<br />
⎝ ⎠<br />
2 21<br />
cos t = 1−<br />
25<br />
4 2<br />
cost<br />
= =<br />
25 5<br />
π<br />
Because 0 ≤ t < , cos t is positive.<br />
2<br />
⎛ 1 ⎞<br />
sin1.7csc1.7 = sin1.7⎜<br />
= 1<br />
sin1.7<br />
⎟<br />
⎝ ⎠<br />
⎛ 1 ⎞<br />
cos 2.3 sec 2.3 = cos 2.3⎜<br />
= 1<br />
cos 2.3<br />
⎟<br />
⎝ ⎠<br />
15 15<br />
cost<br />
= =<br />
64 8<br />
π<br />
Because 0 ≤ t < , cos t is positive.<br />
2<br />
35.<br />
36.<br />
2 π 2 π<br />
sin + cos = 1 by the Pythagorean identity.<br />
6 2<br />
2 π 2 π<br />
sin + cos = 1 because<br />
3 3<br />
2 2<br />
sin t+ cos t = 1.<br />
37.<br />
2 π 2 π<br />
2 2<br />
sec − tan = 1 because 1+ tan t = sec t.<br />
3 3<br />
38.<br />
2 π 2 π<br />
csc − cot = 1 because<br />
6 6<br />
2 2<br />
1+ cot t = csc t.<br />
501<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
39.<br />
40.<br />
41.<br />
42.<br />
43.<br />
44.<br />
45.<br />
9π ⎛π ⎞ π 2<br />
cos = cos 2π<br />
cos<br />
4<br />
⎜ +<br />
4<br />
⎟= =<br />
⎝ ⎠ 4 2<br />
9π ⎛π ⎞ π<br />
csc = csc 2π<br />
csc 2<br />
4<br />
⎜ + = =<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
⎛ 9π ⎞ ⎛ 9π ⎞ 7π<br />
2<br />
sin ⎜− sin 4π<br />
sin<br />
4<br />
⎟= ⎜− + = = −<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ 4 2<br />
⎛ 9π ⎞ ⎛ 9π ⎞ 7π<br />
sec⎜− sec 4π<br />
sec 2<br />
4<br />
⎟= ⎜− + = =<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ 4<br />
5π ⎛π ⎞ π<br />
tan = tan π tan 1<br />
4<br />
⎜ + = =<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
5π ⎛π ⎞ π<br />
cot = cot π cot 1<br />
4<br />
⎜ + = =<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
⎛ 5π ⎞ ⎛3π ⎞ 3π<br />
cot ⎜− cot 2π<br />
cot 1<br />
4<br />
⎟= ⎜ − = = −<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ 4<br />
⎛ π ⎞ ⎛ π ⎞<br />
51. cos ⎜− − 1000π<br />
cos 2π<br />
4<br />
⎟ = ⎜− +<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
7π<br />
= cos 4<br />
2<br />
=<br />
2<br />
⎛ π ⎞ ⎛ π ⎞<br />
52. cos ⎜− − 2000π<br />
cos 2π<br />
4<br />
⎟ = ⎜− +<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
7π<br />
= cos 4<br />
2<br />
=<br />
2<br />
53. a.<br />
b.<br />
3π 2<br />
sin = 4 2<br />
11π ⎛3π ⎞ 3π<br />
2<br />
sin = sin 2π<br />
sin<br />
4<br />
⎜ +<br />
4<br />
⎟ = =<br />
⎝ ⎠ 4 2<br />
46.<br />
⎛ 9π ⎞ ⎛ 9π ⎞ 3π<br />
tan ⎜− tan 3π<br />
tan 1<br />
4<br />
⎟ = ⎜− + = = −<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ 4<br />
54. a.<br />
3π 2<br />
cos =− 4 2<br />
⎛π<br />
⎞ π<br />
47. − tan ⎜ + 15π<br />
=− tan = −1<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
b.<br />
11π ⎛3π ⎞ 3π<br />
2<br />
cos = cos 2π<br />
cos<br />
4<br />
⎜ +<br />
4<br />
⎟= =−<br />
⎝ ⎠ 4 2<br />
⎛π<br />
⎞ π<br />
48. − cot ⎜ + 17π<br />
=− cot = −1<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
⎛ π ⎞ ⎛ π ⎞<br />
49. sin ⎜− − 1000π<br />
sin 2π<br />
4<br />
⎟= ⎜− +<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
7π<br />
= sin 4<br />
2<br />
=−<br />
2<br />
⎛ π ⎞ ⎛ π ⎞<br />
50. sin ⎜− − 2000π<br />
sin 2π<br />
4<br />
⎟= ⎜− +<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
7π<br />
= sin 4<br />
2<br />
=−<br />
2<br />
π<br />
55. a. cos = 0<br />
2<br />
b.<br />
π<br />
56. a. sin = 1<br />
2<br />
b.<br />
9π<br />
⎛π<br />
⎞<br />
cos = cos 4<br />
2<br />
⎜ + π<br />
2<br />
⎟<br />
⎝ ⎠<br />
⎡π<br />
⎤<br />
= cos ⎢ + 2(2 π )<br />
2<br />
⎥<br />
⎣ ⎦<br />
π<br />
= cos 2<br />
= 0<br />
9π ⎛π ⎞ π<br />
sin = sin 4π<br />
sin 1<br />
2<br />
⎜ + = =<br />
2<br />
⎟<br />
⎝ ⎠ 2<br />
502<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.2<br />
0<br />
π<br />
57. a. tanπ = = 0<br />
69. cot ≈ 3.7321<br />
−1<br />
12<br />
b. tan17π = tan( π + 16 π)<br />
π<br />
70. cot ≈ 5.6713<br />
= tan[ π + 8(2 π)]<br />
18<br />
= tanπ<br />
71. sin( −t) − sin t = −sin t− sin t = − 2sin t =− 2a<br />
= 0<br />
72. tan( −t) − tan t = −tan t− tan t = − 2 tan t = − 2c<br />
π 0<br />
58. a. cot = = 0<br />
2 1<br />
73. 4cos( −t) − cos t = 4cost− cost = 3cost = 3b<br />
15π ⎛π ⎞ π<br />
b. cot = cot + 7π<br />
= cot = 0<br />
2<br />
⎜<br />
2<br />
⎟<br />
74. 3cos( −t) − cost = 3cost− cost = 2cost = 2b<br />
⎝ ⎠ 2<br />
75. sin( t+ 2 π) − cos( t+ 4 π) + tan( t+<br />
π)<br />
7π 2<br />
59. a. sin =−<br />
= sin( t) − cos( t) + tan( t)<br />
4 2<br />
= a− b+<br />
c<br />
47π<br />
⎛7π<br />
⎞<br />
b. sin = sin + 10π<br />
76. sin( t+ 2 π) + cos( t+ 4 π) − tan( t+<br />
π)<br />
4<br />
⎜<br />
4<br />
⎟<br />
⎝ ⎠<br />
= sin( t) + cos( t) −tan( t)<br />
⎡7π<br />
⎤<br />
= sin ⎢ + 5( 2π<br />
)<br />
= a+ b−c<br />
4<br />
⎥<br />
⎣ ⎦<br />
7π<br />
77. sin( −t−2 π) −cos( −t−4 π) −tan( −t−π)<br />
= sin 4<br />
= − sin( t+ 2 π) − cos( t+ 4 π) + tan( t+<br />
π)<br />
2<br />
=−sin( t) − cos( t) + tan( t)<br />
=−<br />
2<br />
=−a− b+<br />
c<br />
7π 2<br />
78. sin( −t− 2 π) + cos( −t−4 π) −tan( −t−π)<br />
60. a. cos = 4 2<br />
= − sin( t+ 2 π) + cos( t+ 4 π) + tan( t+<br />
π)<br />
=− sin( t) + cos( t) + tan( t)<br />
47π ⎛7π ⎞ 7π<br />
2<br />
b. cos = cos + 10π<br />
= cos =<br />
=− a+ b+<br />
c<br />
4<br />
⎜<br />
4<br />
⎟<br />
⎝ ⎠ 4 2<br />
79. cost+ cos( t+ 1000 π) −tan t− tan( t+<br />
999 π)<br />
61. sin 0.8 ≈ 0.7174<br />
− sin t+ 4sin( t−1000 π )<br />
= cost+ cost−tan t−tan t− sin t+<br />
4sin t<br />
≈ 62. cos 0.6 0.8253<br />
= 2cost− 2tant+<br />
3sint<br />
63. tan 3.4 0.2643<br />
= 3a+ 2b−<br />
≈ 2c<br />
64. tan ≈ 3.7 0.6247<br />
80. − cost+ 7cos( t+ 1000 π) + tan t+ tan( t+<br />
999 π)<br />
65. csc 1 1.1884<br />
+ sin ≈ t+ sin( t−1000 π )<br />
=− cost+ 7 cost+ tan t+ tan t+ sin t+<br />
sin t<br />
66. sec ≈ 1 1.8508<br />
= 6cost+ 2tant+<br />
2sint<br />
π = 2a+ 6b+<br />
2c<br />
67. cos ≈ 0.9511<br />
10<br />
3π 68. sin ≈ 0.8090<br />
10<br />
503<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
81. a.<br />
b.<br />
c.<br />
82. a.<br />
b.<br />
c.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 8.3sin ⎢ (80 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
= 12 + 8.3sin 0 = 12 + 8.3(0)<br />
= 12<br />
There are 12 hours of daylight in Fairbanks on<br />
March 21.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 8.3sin ⎢ (172 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
≈ 12 + 8.3sin1.5837<br />
≈ 20.3<br />
There are about 20.3 hours of daylight in<br />
Fairbanks on June 21.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 8.3sin ⎢ (355 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
≈ 12 + 8.3sin 4.7339<br />
≈ 3.7<br />
There are about 3.7 hours of daylight in<br />
Fairbanks on December 21.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 24sin ⎢ (80 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
12 + 24sin 0 = 12 + 24(0) = 12<br />
There are 12 hours of daylight in San Diego on<br />
March 21.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 24sin ⎢ (172 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
≈ 12 + 24sin1.5837<br />
≈ 14.3998<br />
There are about 14.4 hours of daylight in San<br />
Diego on June 21.<br />
⎡ 2π<br />
⎤<br />
H = 12 + 24sin ⎢ (355 −80)<br />
365<br />
⎥<br />
⎣<br />
⎦<br />
≈ 12 + 24sin 4.7339<br />
≈ 9.6<br />
There are about 9.6 hours of daylight in San<br />
Diego on December 21.<br />
83. a. For t = 7,<br />
π π<br />
E = sin ⋅ 7 = sin = 1<br />
14 2<br />
For t = 14,<br />
π<br />
E = sin ⋅ 14 = sinπ<br />
= 0<br />
14<br />
For t = 21,<br />
π 3π<br />
E = sin ⋅ 21 = sin = −1<br />
14 2<br />
For t = 28,<br />
π<br />
E = sin ⋅ 28 = sin 2π<br />
= sin 0 = 0<br />
14<br />
For t = 35,<br />
π 5π π<br />
E = sin ⋅ 35 = sin = sin = 1<br />
14 2 2<br />
Observations may vary.<br />
b. Because E(35) = E(7) = 1, the period is<br />
35 – 7 = 28 or 28 days.<br />
84. a. At 6 A.M., t = 0.<br />
π<br />
H = 10 + 4sin ⋅0<br />
6<br />
= 10+ 4sin0= 10+ 4⋅ 0=<br />
10<br />
The height is 10 feet.<br />
At 9 A.M., t = 3.<br />
π<br />
H = 10 + 4sin ⋅3<br />
6<br />
π<br />
= 10 + 4sin = 10 + 4(1) = 14<br />
2<br />
The height is 14 feet.<br />
At noon, t = 6.<br />
π<br />
H = 10 + 4sin ⋅6<br />
6<br />
= 10 + 4sinπ<br />
= 10 + 4⋅ 0 = 10<br />
The height is 10 feet.<br />
At 6 P.M., t = 12.<br />
π<br />
H = 10 + 4sin ⋅12<br />
6<br />
= 10 + 4sin 2π<br />
= 10 + 4⋅ 0 = 10<br />
The height is 10 feet.<br />
At midnight, t = 18.<br />
504<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.2<br />
π<br />
H = 10 + 4sin ⋅18<br />
6<br />
= 10 + 4sin 3π<br />
= 10 + 4sinπ<br />
= 10 + 4⋅ 0 = 10<br />
The height is 10 feet.<br />
At 3 A.M., t = 21.<br />
π<br />
H = 10 + 4sin ⋅21<br />
6<br />
7π<br />
3π<br />
= 10 + 4sin = 10 + 4sin<br />
2 2<br />
= 10 + 4( − 1) = 6<br />
The height is 6 feet.<br />
b. The sine function has a minimum at 3 π . Thus,<br />
2<br />
π 3π<br />
we find a low tide at t = or<br />
6 2<br />
t = 9. This value of t corresponds to 3 P.M. For t<br />
= 9,<br />
π<br />
h = 10 + 4sin ⋅9<br />
6<br />
3π<br />
= 10 + 4sin = 10 + 4( − 1) = 6<br />
2<br />
The height is 6 feet. From part a, the height at 3<br />
A.M. is also 6 feet. Thus, low tide is at<br />
3 A.M. and 3 P.M.<br />
The sine function has a maximum at 2<br />
π . Thus,<br />
π π<br />
we find a high tide at t = or t = 3. This<br />
6 2<br />
value of t corresponds to 9 a.m. From part a, the<br />
height at 9 A.M. is 14 feet. Because the sine has<br />
a period of we also find a maximum at 5 π<br />
2π .<br />
2<br />
π 5π<br />
We find another high tide at t = or t = 15.<br />
6 2<br />
This value of t corresponds to 9 P.M. Thus, high<br />
tide is at 9 A.M. and 9 P.M.<br />
c. The period of 2π the sine function is or on the<br />
interval [0, 2 π ] . The cycle of the sine function<br />
π 5π<br />
π<br />
starts at t = or t = 0, and ends at 2<br />
6 2<br />
6 t = π<br />
or t = 12. Thus, the period is 12 hours, which<br />
means high and low tides occur every 12 hours.<br />
85. – 96. Answers may vary.<br />
97. makes sense<br />
98. does not make sense; Explanations will vary.<br />
Sample explanation: sin t cannot be less than − 1.<br />
10<br />
Note that − ≈ − 1.58 < − 1.<br />
2<br />
99. does not make sense; Explanations will vary.<br />
Sample explanation: Cosine is not an odd function.<br />
100. makes sense<br />
101. t is in the third quadrant therefore sin t < 0,<br />
tan t > 0, and cot t > 0.<br />
Thus, only choice (c) is true.<br />
102.<br />
1<br />
f( x) = sin x and f( a)<br />
=<br />
4<br />
f( a) + f( a+ 2 π) + f( a+ 4 π) + f( a+<br />
6 π)<br />
⎛1<br />
⎞<br />
= 4 f( a) = 4⎜<br />
1 because sin x has a<br />
4<br />
⎟=<br />
⎝ ⎠<br />
period of 2 π.<br />
1<br />
103. f( x) = sin x and f( a)<br />
=<br />
4<br />
f( a) + 2 f( − a) = f( a) −2 f( a)<br />
1 ⎛1⎞<br />
= −2<br />
4 ⎜<br />
4 ⎟<br />
⎝ ⎠<br />
1<br />
=−<br />
4<br />
f(–a) = –f(a) because sin (–x) = –sin x.<br />
Sine is an odd function.<br />
104. The height is given by<br />
h = 45 + 40 sin(t – 90°)<br />
h (765 ° ) = 45 + 40sin(765°− 90 ° )<br />
≈ 16.7<br />
You are about 16.7 feet above the ground.<br />
105. First find the hypotenuse.<br />
2 2 2<br />
c = a + b<br />
2 2 2<br />
c = 5 + 12<br />
2<br />
c = 25 + 144<br />
2<br />
c = 169<br />
c = 13<br />
Next write the ratio.<br />
a 5<br />
c = 13<br />
505<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
106. First find the hypotenuse.<br />
2 2 2<br />
c = a + b<br />
2 2 2<br />
c = 1 + 1<br />
2<br />
c = 1+<br />
1<br />
2<br />
c = 2<br />
c = 2<br />
Next write the ratio and simplify.<br />
a 1<br />
c = 2<br />
1 2<br />
= ⋅<br />
2 2<br />
2<br />
=<br />
2<br />
2 2 2 2<br />
+ = +<br />
2 2<br />
⎛a⎞ ⎛b⎞<br />
a b<br />
107. ⎜<br />
c<br />
⎟ ⎜<br />
c<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ c c<br />
2 2<br />
a + b<br />
=<br />
2<br />
c<br />
2 2 2<br />
Since c = a + b , continue simplifying by<br />
2 2<br />
substituting c for a + b<br />
2 .<br />
2 2 2 2<br />
⎛a⎞ ⎛b⎞<br />
a b<br />
⎜<br />
c<br />
⎟ + ⎜<br />
c<br />
⎟ = +<br />
2 2<br />
⎝ ⎠ ⎝ ⎠ c c<br />
2 2<br />
a + b<br />
=<br />
2<br />
c<br />
2<br />
c<br />
2 2<br />
a + b<br />
=<br />
2<br />
c<br />
2<br />
c<br />
=<br />
2<br />
c<br />
= 1<br />
Section 4.3<br />
Check Point Exercises<br />
2 2 2<br />
1. Use the Pythagorean Theorem, c = a + b , to find<br />
c.<br />
a = 3, b = 4<br />
2 2 2 2 2<br />
c = a + b = 3 + 4 = 9+ 16=<br />
25<br />
c = 25 = 5<br />
Referring to these lengths as opposite, adjacent, and<br />
hypotenuse, we have<br />
opposite 3<br />
sinθ<br />
= =<br />
hypotenuse 5<br />
adjacent 4<br />
cosθ<br />
= =<br />
hypotenuse 5<br />
opposite 3<br />
tanθ<br />
= =<br />
adjacent 4<br />
hypotenuse 5<br />
cscθ<br />
= =<br />
opposite 3<br />
hypotenuse 5<br />
secθ<br />
= =<br />
adjacent 4<br />
adjacent 4<br />
cotθ<br />
= =<br />
opposite 3<br />
2. Use the Pythagorean Theorem,<br />
2 2 2<br />
c = a + b , to find<br />
b.<br />
2 2 2<br />
a + b = c<br />
2 2 2<br />
1 + b = 5<br />
2<br />
1+ b = 25<br />
2<br />
b = 24<br />
b = 24 = 2 6<br />
Note that side a is opposite θ and side b is adjacent<br />
to θ .<br />
opposite 1<br />
sinθ<br />
= =<br />
hypotenuse 5<br />
adjacent 2 6<br />
cosθ<br />
= =<br />
hypotenuse 5<br />
opposite 1 6<br />
tanθ<br />
= = =<br />
adjacent 2 6 12<br />
hypotenuse 5<br />
cscθ<br />
= = = 5<br />
opposite 1<br />
hypotenuse 5 5 6<br />
secθ<br />
= = =<br />
adjacent 2 6 12<br />
adjacent 2 6<br />
cotθ<br />
= = = 2 6<br />
opposite 1<br />
506<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.3<br />
3. Apply the definitions of these three trigonometric functions.<br />
length of hypotenuse<br />
csc 45°=<br />
length of side opposite 45°<br />
2<br />
= = 2<br />
1<br />
length of hypotenuse<br />
sec45°=<br />
length of side adjacent to 45°<br />
2<br />
= = 2<br />
1<br />
length of side adjacent to 45°<br />
cot 45°=<br />
length of side opposite 45°<br />
1<br />
= = 1<br />
1<br />
4.<br />
length of side opposite 60°<br />
tan 60°=<br />
length of side adjacent to 60°<br />
3<br />
= = 3<br />
1<br />
length of side opposite 30°<br />
tan 30°=<br />
length of side adjacent to 30°<br />
1 1 3 3<br />
= = ⋅ =<br />
3 3 3 3<br />
5. a.<br />
sin 46<br />
o<br />
= cos(90<br />
o<br />
− 46<br />
o<br />
) = cos 44<br />
o<br />
π ⎛π π ⎞<br />
b. cot = tan ⎜ − ⎟<br />
12 ⎝ 2 12 ⎠<br />
⎛6π<br />
π ⎞<br />
= tan ⎜ − ⎟<br />
⎝ 12 12 ⎠<br />
5π<br />
= tan 12<br />
6. Because we have a known angle, an unknown opposite side, and a known adjacent side, we select the<br />
tangent function.<br />
tan 24<br />
0 a<br />
=<br />
750<br />
a = 750 tan 24<br />
0<br />
a ≈750(0.4452) ≈334<br />
The distance across the lake is approximately 334 yards.<br />
507<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
7.<br />
side opposite 14<br />
tanθ = =<br />
side adjacent 10<br />
Use a calculator in degree mode to find θ .<br />
Many Scientific Calculators<br />
Many Graphing Calculators<br />
−1<br />
TAN ( 14 10 ) ENTER<br />
÷ TAN ( 14 ÷ 10 ) ENTER<br />
The display should show approximately 54. Thus, the angle of elevation of the sun is approximately 54°.<br />
Exercise Set 4.3<br />
1.<br />
2.<br />
c<br />
= 9 + 12 = 225<br />
2 2 2<br />
c = 225 = 15<br />
opposite 9 3<br />
sinθ<br />
= = =<br />
hypotenuse 15 5<br />
adjacent 12 4<br />
cosθ<br />
= = =<br />
hypotenuse 15 5<br />
opposite 9 3<br />
tanθ<br />
= = =<br />
adjacent 12 4<br />
hypotenuse 15 5<br />
cscθ<br />
= = =<br />
opposite 9 3<br />
hypotenuse 15 5<br />
secθ<br />
= = =<br />
adjacent 12 4<br />
adjacent 12 4<br />
cotθ<br />
= = =<br />
opposite 9 3<br />
c<br />
= 6 + 8 = 100<br />
2 2 2<br />
c = 100 = 10<br />
opposite 6 3<br />
sinθ<br />
= = =<br />
hypotenuse 10 5<br />
adjacent 8 4<br />
cosθ<br />
= = =<br />
hypotenuse 10 5<br />
opposite 6 3<br />
tanθ<br />
= = =<br />
adjacent 8 4<br />
hypotenuse 10 5<br />
cscθ<br />
= = =<br />
opposite 6 3<br />
hypotenuse 10 5<br />
secθ<br />
= = =<br />
adjacent 8 4<br />
adjacent 8 4<br />
cotθ<br />
= = =<br />
opposite 6 3<br />
508<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.3<br />
3.<br />
2 2 2<br />
a + 21 = 29<br />
5.<br />
10 + b = 26<br />
2 2 2<br />
a<br />
2<br />
= 841− 441 = 400<br />
b<br />
2<br />
= 676 − 100 = 576<br />
a = 400 = 20<br />
opposite 20<br />
sinθ<br />
= =<br />
hypotenuse 29<br />
adjacent 21<br />
cosθ<br />
= =<br />
hypotenuse 29<br />
opposite 20<br />
tanθ<br />
= =<br />
adjacent 21<br />
hypotenuse 29<br />
cscθ<br />
= =<br />
opposite 20<br />
hypotenuse 29<br />
secθ<br />
= =<br />
adjacent 21<br />
adjacent 21<br />
cotθ<br />
= =<br />
opposite 20<br />
b = 576 = 24<br />
opposite 10 5<br />
sinθ<br />
= = =<br />
hypotenuse 26 13<br />
adjacent 24 12<br />
cosθ<br />
= = =<br />
hypotenuse 26 13<br />
opposite 10 5<br />
tanθ<br />
= = =<br />
adjacent 24 12<br />
hypotenuse 26 13<br />
cscθ<br />
= = =<br />
opposite 10 5<br />
hypotenuse 26 13<br />
secθ<br />
= = =<br />
adjacent 24 12<br />
adjacent 24 12<br />
cotθ<br />
= = =<br />
opposite 10 5<br />
4.<br />
2 2 2<br />
a + 15 = 17<br />
6.<br />
2 2 2<br />
a + 40 = 41<br />
a<br />
2<br />
= 289 − 225 = 64<br />
a<br />
2<br />
= 1681− 1600 = 81<br />
a = 64 = 8<br />
opposite 8<br />
sinθ<br />
= =<br />
hypotenuse 17<br />
adjacent 15<br />
cosθ<br />
= =<br />
hypotenuse 17<br />
opposite 8<br />
tanθ<br />
= =<br />
adjacent 15<br />
hypotenuse 17<br />
cscθ<br />
= =<br />
opposite 8<br />
hypotenuse 17<br />
secθ<br />
= =<br />
adjacent 15<br />
adjacent 15<br />
cotθ<br />
= =<br />
opposite 8<br />
a = 81 = 9<br />
opposite 9<br />
sinθ<br />
= =<br />
hypotenuse 41<br />
adjacent 40<br />
cosθ<br />
= =<br />
hypotenuse 41<br />
opposite 9<br />
tanθ<br />
= =<br />
adjacent 40<br />
hypotenuse 41<br />
cscθ<br />
= =<br />
opposite 9<br />
hypotenuse 41<br />
secθ<br />
= =<br />
adjacent 40<br />
adjacent 40<br />
cotθ<br />
= =<br />
opposite 9<br />
509<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
7.<br />
8.<br />
a<br />
+ 21 = 35<br />
2 2 2<br />
a<br />
2<br />
= 1225 − 441 = 784<br />
a = 784 = 28<br />
opposite 28 4<br />
sinθ<br />
= = =<br />
hypotenuse 35 5<br />
adjacent 21 3<br />
cosθ<br />
= = =<br />
hypotenuse 35 5<br />
opposite 28 4<br />
tanθ<br />
= = =<br />
adjacent 21 3<br />
hypotenuse 35 5<br />
cscθ<br />
= = =<br />
opposite 28 4<br />
hypotenuse 35 5<br />
secθ<br />
= = =<br />
adjacent 21 3<br />
adjacent 21 3<br />
cotθ<br />
= = =<br />
opposite 28 4<br />
24 + b = 25<br />
2 2 2<br />
b<br />
2<br />
= 625 − 576 = 49<br />
b = 49 = 7<br />
opposite 24<br />
sinθ<br />
= =<br />
hypotenuse 25<br />
adjacent 7<br />
cosθ<br />
= =<br />
hypotenuse 25<br />
opposite 24<br />
tanθ<br />
= =<br />
adjacent 7<br />
hypotenuse 25<br />
cscθ<br />
= =<br />
opposite 24<br />
hypotenuse 25<br />
secθ<br />
= =<br />
adjacent 7<br />
adjacent 7<br />
cotθ<br />
= =<br />
opposite 24<br />
11.<br />
12.<br />
length of hypotenuse<br />
sec 45°=<br />
length of side adjacent to 45°<br />
2<br />
= = 2<br />
1<br />
length of hypotenuse<br />
csc 45°=<br />
length of side opposite 45°<br />
2<br />
= = 2<br />
1<br />
π<br />
13. tan = tan 60 °<br />
3<br />
length of side opposite 60°<br />
=<br />
length of side adjacent to 60°<br />
3<br />
= = 3<br />
1<br />
14.<br />
π length of side adjacent to 60°<br />
cot = cot 60°=<br />
3 length of side opposite 60°<br />
1 1 3 3<br />
= = ⋅ =<br />
3 3 3 3<br />
π π<br />
15. sin − cos = sin 45°− cos 45°<br />
4 4<br />
1 1<br />
= − = 0<br />
2 2<br />
π π<br />
16. tan + csc = tan 45°+ csc30°<br />
4 6<br />
1 2<br />
= + = 1 + 2 = 3<br />
1 1<br />
17. π π π ⎛ 3 ⎞⎛ 2 ⎞<br />
sin cos − tan = −1<br />
3 4 4 ⎜<br />
⎟⎜ 2 ⎟⎜ 2 ⎟<br />
⎝ ⎠⎝ ⎠<br />
9.<br />
length of side adjacent to 30°<br />
cos30°=<br />
length of hypotenuse<br />
3<br />
=<br />
2<br />
6<br />
= − 1<br />
4<br />
6 − 4<br />
=<br />
4<br />
10.<br />
length of side opposite 30°<br />
tan 30°=<br />
length of side adjacent to 30°<br />
18.<br />
π π π 3 3−<br />
3<br />
cos sec − cot = 1− =<br />
3 3 3 3 3<br />
1 1 3 3<br />
= = ⋅ =<br />
3 3 3 3<br />
510<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.3<br />
π π π ⎛ 2 ⎞⎛ 3 ⎞<br />
+ = +⎜<br />
3 4 6 ⎜<br />
⎟⎜<br />
2 ⎟⎜ 3 ⎟<br />
⎝ ⎠⎝ ⎠<br />
6<br />
= 2 3+<br />
6<br />
12 3 + 6<br />
=<br />
6<br />
19. 2 tan cos tan 2( 3 )<br />
20.<br />
π π π ⎛ 3 ⎞⎛2 3 ⎞<br />
6tan + sin sec = 6(1) +⎜<br />
4 3 6 ⎜<br />
⎟⎜<br />
2 ⎟⎜ 3 ⎟<br />
⎝ ⎠⎝ ⎠<br />
6<br />
= 6 + 6<br />
= 7<br />
21. sin 7°= cos(90°− 7 ° ) = cos83°<br />
22. sin19°= cos( 90°− 19° ) = cos71°<br />
23. csc 25° = sec(90° – 25 o ) = sec 65°<br />
24. csc35°= sec(90°− 35 ° ) = sec55°<br />
π ⎛π π ⎞<br />
25. tan = cot ⎜ − ⎟<br />
9 ⎝ 2 9 ⎠<br />
⎛9π<br />
2π<br />
⎞<br />
= cot ⎜ − ⎟<br />
⎝18 18 ⎠<br />
7π<br />
= cot 18<br />
26.<br />
27.<br />
7 2 5<br />
tan π ⎛<br />
cot π π ⎞ ⎛<br />
cot π π ⎞<br />
= ⎜ − ⎟= ⎜ − ⎟ = cot<br />
π<br />
7 ⎝ 2 7 ⎠ ⎝14 14 ⎠ 14<br />
2π ⎛π 2π<br />
⎞<br />
cos = sin ⎜ − ⎟<br />
5 ⎝ 2 5 ⎠<br />
⎛5π<br />
4π<br />
⎞<br />
= sin ⎜ − ⎟<br />
⎝ 10 10 ⎠<br />
π<br />
= sin 10<br />
3 3 4 3<br />
28. cos π ⎛<br />
sin π π ⎞ ⎛<br />
sin π π ⎞<br />
= ⎜ − ⎟= ⎜ − ⎟=<br />
sin<br />
π<br />
8 ⎝ 2 8 ⎠ ⎝ 8 8 ⎠ 8<br />
a<br />
29. tan 37°=<br />
250<br />
a = 250 tan 37°<br />
a ≈ 250(0.7536) ≈188 cm<br />
a<br />
30. tan 61°=<br />
10<br />
a = 10 tan 61°<br />
a ≈10(1.8040) ≈18 cm<br />
b<br />
31. cos34°=<br />
220<br />
b = 220cos34°<br />
b ≈220(0.8290) ≈182 in.<br />
a<br />
32. sin 34°=<br />
13<br />
a = 13sin 34°<br />
a ≈13(0.5592) ≈7 m<br />
16<br />
33. sin 23°=<br />
c<br />
16 16<br />
c = ≈ ≈ 41 m<br />
sin 23°<br />
0.3907<br />
23<br />
34. tan 44°=<br />
b<br />
23 23<br />
b = ≈ ≈ 24 yd<br />
tan 44°<br />
0.9657<br />
511<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
35. Scientific Calculator Graphing Calculator<br />
.2974<br />
1<br />
SIN − −<br />
SIN<br />
1<br />
.2974 ENTER<br />
Display<br />
(rounded to the nearest degree)<br />
17<br />
If sinθ<br />
= 0.2974, then θ ≈ 17 ° .<br />
36. Scientific Calculator Graphing Calculator<br />
Display<br />
(rounded to the nearest degree)<br />
.877 COS -1 COS -1 .877 ENTER 29<br />
If cosθ<br />
= 0.877, then θ ≈ 29°.<br />
37. Scientific Calculator Graphing Calculator<br />
1<br />
4.6252 TAN − −<br />
TAN<br />
1<br />
If tanθ<br />
= 4.6252, then θ ≈ 78 ° .<br />
4.6252 ENTER<br />
Display<br />
(rounded to the nearest degree)<br />
78<br />
38. Scientific Calculator Graphing Calculator<br />
Display<br />
(rounded to the nearest degree)<br />
26.0307 TAN -1 TAN -1 26.0307 ENTER 88<br />
If tanθ<br />
= 26.0307, then θ ≈ 88 ° .<br />
39. Scientific Calculator Graphing Calculator<br />
1<br />
.4112 COS − −<br />
COS<br />
1<br />
If cosθ<br />
= 0.4112, then θ ≈ 1.147 radians.<br />
.4112 ENTER<br />
Display<br />
(rounded to three places)<br />
1.147<br />
40. Scientific Calculator Graphing Calculator<br />
Display<br />
(rounded to three places)<br />
.9499 SIN -1 SIN -1 .9499 ENTER 1.253<br />
If sinθ<br />
= 0.9499, then θ = 1.253 radians.<br />
41. Scientific Calculator Graphing Calculator<br />
1<br />
.4169 TAN − −<br />
TAN<br />
1<br />
If tanθ<br />
= 0.4169, then θ ≈ 0.395 radians.<br />
.4169 ENTER<br />
Display<br />
(rounded to three places)<br />
.395<br />
42. Scientific Calculator Graphing Calculator<br />
.5117<br />
If tanθ<br />
= 0.5117, then θ = 0.473<br />
Display<br />
(rounded to three places)<br />
1<br />
TAN − TAN − 1 .5117 ENTER .473<br />
512<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.3<br />
43.<br />
π<br />
tan<br />
3 1 3 1<br />
− = −<br />
2 π<br />
sec<br />
2<br />
6<br />
1<br />
π<br />
cos 6<br />
3 1<br />
= −<br />
2<br />
1<br />
3<br />
2<br />
3 3<br />
= −<br />
2 2<br />
= 0<br />
44.<br />
45.<br />
46.<br />
1 2 1 2<br />
− = −<br />
π π<br />
cot csc<br />
4 6<br />
1 2<br />
= −<br />
1 1<br />
π π<br />
tan sin<br />
4 6<br />
1 1<br />
1 1 2<br />
1 2<br />
= −<br />
1 2<br />
= 1−1<br />
= 0<br />
2 2<br />
1+ sin 40°+ sin 50°<br />
2 2<br />
= 1+ sin (90°− 50 ° ) + sin 50°<br />
2 2<br />
= 1+ cos 50°+ sin 50°<br />
= 1+<br />
1<br />
= 2<br />
2 2<br />
1− tan 10°+ csc 80°<br />
2 2<br />
= 1− cot 80°+ csc 80°<br />
2 2<br />
= 1+ csc 80°− cot 80°<br />
= 1+<br />
1<br />
= 2<br />
47. csc37° sec53°− tan 53° cot 37°<br />
= sec53° sec53°− tan 53° tan 53°<br />
2 2<br />
= sec 53°− tan 53°<br />
= 1<br />
48. cos12° sin 78°+ cos 78° sin12°<br />
= sin 78° sin 78°+ cos 78° cos 78°<br />
2 2<br />
= sin 78°+ cos 78°<br />
= 1<br />
513<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
49. f ( θ) = 2cosθ − cos2θ<br />
⎛π ⎞ π ⎛ π ⎞<br />
f ⎜ 2cos cos 2<br />
6<br />
⎟= −<br />
6<br />
⎜ ⋅<br />
6<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
⎛ 3 ⎞ ⎛π<br />
⎞<br />
= 2 −cos<br />
⎜<br />
2 ⎟ ⎜<br />
3<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
2 3 1<br />
= −<br />
2 2<br />
2 3 −1<br />
=<br />
2<br />
θ<br />
50. f ( θ) = 2sinθ<br />
− sin 2<br />
π<br />
⎛π<br />
⎞ π<br />
3<br />
f ⎜ 2sin sin<br />
3<br />
⎟ = −<br />
⎝ ⎠ 3 2<br />
⎛ 3 ⎞ ⎛π<br />
⎞<br />
= 2 −sin<br />
⎜<br />
2 ⎟ ⎜<br />
6<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
2 3 1<br />
= −<br />
2 2<br />
2 3 −1<br />
=<br />
2<br />
51.<br />
52.<br />
⎛<br />
1<br />
tan<br />
π ⎞<br />
⎜ − θ = cot θ =<br />
2<br />
⎟<br />
⎝ ⎠ 4<br />
⎛π<br />
⎞ 1 1<br />
csc⎜<br />
− θ secθ<br />
3<br />
2<br />
⎟= = = =<br />
⎝ ⎠ cosθ<br />
1<br />
3<br />
a<br />
53. tan 40°=<br />
630<br />
a = 630 tan 40°<br />
a ≈630(0.8391) ≈529<br />
The distance across the lake is approximately 529 yards.<br />
h<br />
54. tan 40°=<br />
35<br />
h = 35tan 40°<br />
h ≈35(0.8391) ≈ 29<br />
The tree’s height is approximately 29 feet.<br />
55.<br />
125<br />
tanθ =<br />
172<br />
Use a calculator in degree mode to find θ .<br />
Many Scientific Calculators<br />
125 ÷ 172 = TAN −1<br />
Many Graphing Calculators<br />
−1<br />
TAN ( 125 ÷ 172 ) ENTER<br />
The display should show approximately 36. Thus, the angle of elevation of the sun is approximately 36°.<br />
514<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.3<br />
56.<br />
57.<br />
555<br />
tan 1320<br />
Use a calculator in degree mode to find θ .<br />
Many Scientific Calculators<br />
Many Graphing Calculators<br />
555 ÷ 1320 = TAN -1 TAN -1 ( 555 ÷ 1320 ) ENTER<br />
The display should show approximately 23. Thus, the angle of elevation is approximately 23°.<br />
500<br />
sin10°=<br />
c<br />
500 500<br />
c = ≈ ≈ 2880<br />
sin10°<br />
0.1736<br />
The plane has flown approximately 2880 feet.<br />
a<br />
58. sin 5°=<br />
5000<br />
a = 5000sin 5°≈ 5000(0.0872) = 436<br />
The driver’s increase in altitude was approximately 436 feet.<br />
59.<br />
60<br />
cosθ =<br />
75<br />
Use a calculator in degree mode to find θ .<br />
Many Scientific Calculators<br />
60 ÷ 75 = COS −1<br />
Many Graphing Calculators<br />
−1<br />
COS ( 60 ÷ 75 ) ENTER<br />
The display should show approximately 37. Thus, the angle between the wire and the pole is approximately 37°.<br />
55<br />
60. cosθ =<br />
80<br />
Use a calculator in degree mode to find θ .<br />
61. – 67. Answers may vary.<br />
Many Scientific Calculators<br />
Many Graphing Calculators<br />
55 ÷ 80 = COS -1 COS -1 ( 55 ÷ 80 ) ENTER<br />
The display should show approximately 47. Thus, the angle between the wire and the pole is approximately 47°.<br />
68.<br />
θ 0.4 0.3 0.2 0.1 0.01 0.001 0.0001 0.00001<br />
sinθ 0.3894 0.2955 0.1987 0.0998 0.0099998<br />
9.999998×<br />
10 −4<br />
9.99999998×<br />
10 −5<br />
1×<br />
10 −5<br />
sinθ<br />
θ<br />
0.9736 0.9851 0.9933 0.9983 0.99998 0.9999998 0.999999998 1<br />
sinθ<br />
approaches 1 as θ approaches 0.<br />
θ<br />
515<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
69.<br />
θ 0.4 0.3 0.2 0.1 0.01 0.001 0.0001 0.00001<br />
cosθ 0.92106 0.95534 0.98007 0.99500 0.99995 0.9999995 0.999999995 1<br />
cosθ<br />
− 1 –0.19735 –0.148878 –0.099667 –0.04996 –0.005 –0.0005 –0.00005 0<br />
θ<br />
cosθ<br />
−1<br />
approaches 0 as θ approaches 0.<br />
θ<br />
70. does not make sense; Explanations will vary. Sample explanation: An increase in the size of a triangle does not affect<br />
the ratios of the sides.<br />
71. does not make sense; Explanations will vary. Sample explanation: This value is irrational. Irrational numbers are<br />
rounded on calculators.<br />
72. does not make sense; Explanations will vary. Sample explanation: The sine and cosine are cofunctions of each other.<br />
The sine and cosine are not reciprocals of each other.<br />
73. makes sense<br />
74. false; Changes to make the statement true will vary. A sample change is:<br />
tan 45 ° ⎛45<br />
tan<br />
° ⎞<br />
≠ ⎜ ⎟<br />
tan15° ⎝15°<br />
⎠<br />
75. true<br />
76. false; Changes to make the statement true will vary. A sample change is:<br />
1 1 2<br />
sin 45°+ cos 45°= + = ≠ 1<br />
2 2 2<br />
77. true<br />
78. In a right triangle, the hypotenuse is greater than either other side. Therefore both<br />
less than 1 for an acute angle in a right triangle.<br />
opposite<br />
hypotenuse<br />
and<br />
adjacent<br />
hypotenuse<br />
must be<br />
79. Use a calculator in degree mode to generate the following table. Then use the table to describe what happens to the<br />
tangent of an acute angle as the angle gets close to 90°.<br />
θ 60 70 80 89 89.9 89.99 89.999 89.9999<br />
tanθ 1.7321 2.7475 5.6713 57 573 5730 57,296 572,958<br />
As θ approaches 90°, tanθ increases without bound. At 90°, tanθ is undefined.<br />
516<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
80. a. Let a = distance of the ship from the lighthouse.<br />
250<br />
tan 35°=<br />
a<br />
250 250<br />
a = ≈ ≈357<br />
tan 35°<br />
0.7002<br />
The ship is approximately 357 feet from the<br />
lighthouse.<br />
81. a.<br />
82. a.<br />
b. Let b = the plane’s height above the lighthouse.<br />
b<br />
tan 22°=<br />
357<br />
b = 357 tan 22°≈357(0.4040) ≈144<br />
144 + 250 = 394<br />
The plane is approximately 394 feet above the<br />
water.<br />
y<br />
r<br />
2 2<br />
b. First find r: r = x + y<br />
2 2<br />
r = ( − 3) + 4<br />
r = 5<br />
y 4<br />
= , which is positive.<br />
r 5<br />
x<br />
r<br />
2 2<br />
b. First find r: r = x + y<br />
r =<br />
2 2<br />
( − 3) + 5<br />
r = 34<br />
x −3 −3 34 −3 34<br />
= = ⋅ = , which is<br />
r 34 34 34 34<br />
negative.<br />
<br />
83. a. θ′ = 360 − 345 = 15<br />
b.<br />
5π 6π 5π π<br />
θ′ = π − = − =<br />
6 6 6 6<br />
Section 4.4<br />
Check Point Exercises<br />
1.<br />
2 2<br />
r = x + y<br />
2 2<br />
r = 1 + ( − 3) = 1+ 9 = 10<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y −3 3 10<br />
sinθ<br />
= = =−<br />
r 10 10<br />
x 1 10<br />
cosθ<br />
= = =<br />
r 10 10<br />
y −3<br />
tanθ<br />
= = = −3<br />
x 1<br />
r 10 10<br />
cscθ<br />
= = = −<br />
y −3 3<br />
r 10<br />
secθ<br />
= = = 10<br />
x 1<br />
x 1 1<br />
cotθ<br />
= = = −<br />
y −3 3<br />
2. a. θ = 0°= 0 radians<br />
The terminal side of the angle is on the positive<br />
x-axis. Select the point<br />
P = (1,0): x = 1, y = 0, r = 1<br />
Apply the definitions of the cosine and cosecant<br />
functions.<br />
x 1<br />
cos 0°= cos 0 = = = 1<br />
r 1<br />
r 1<br />
csc0°= csc0 = = , undefined<br />
y 0<br />
π<br />
b. θ = 90°= radians<br />
2<br />
The terminal side of the angle is on the positive<br />
y-axis. Select the point<br />
P = (0,1): x = 0, y = 1, r = 1<br />
Apply the definitions of the cosine and cosecant<br />
functions.<br />
π x 0<br />
cos90°= cos = = = 0<br />
2 r 1<br />
π r 1<br />
csc90°= csc = = = 1<br />
2 y 1<br />
517<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
c. θ = 180°= π radians<br />
The terminal side of the angle is on the negative<br />
x-axis. Select the point<br />
P = (–1,0): x =− 1, y = 0, r = 1<br />
Apply the definitions of the cosine and cosecant<br />
functions.<br />
x −1<br />
cos180°= cosπ<br />
= = =−1<br />
r 1<br />
r 1<br />
csc180°= csc π = = , undefined<br />
y 0<br />
d.<br />
3π<br />
θ = 270°= radians<br />
2<br />
The terminal side of the angle is on the negative<br />
y-axis. Select the point<br />
P = (0,–1): x = 0, y = − 1, r = 1<br />
Apply the definitions of the cosine and cosecant<br />
functions.<br />
3π<br />
x 0<br />
cos 270°= cos = = = 0<br />
2 r 1<br />
3π<br />
r 1<br />
csc 270°= csc = = =−1<br />
2 y −1<br />
3. Because sinθ<br />
< 0, θ cannot lie in quadrant I; all the<br />
functions are positive in quadrant I. Furthermore, θ<br />
cannot lie in quadrant II; sinθ is positive in quadrant<br />
II. Thus, with sinθ<br />
< 0, θ lies in quadrant III or<br />
quadrant IV. We are also given that cosθ < 0 .<br />
Because quadrant III is the only quadrant in which<br />
cosine is negative and the sine is negative, we<br />
conclude that θ lies in quadrant III.<br />
4. Because the tangent is negative and the cosine is<br />
negative, θ lies in quadrant II. In quadrant II, x is<br />
negative and y is positive. Thus,<br />
1 y 1<br />
tanθ =− = =<br />
3 x − 3<br />
x =− 3, y = 1<br />
Furthermore,<br />
2 2 2 2<br />
r = x + y = ( − 3) + 1 = 9+ 1=<br />
10<br />
Now that we know x, y, and r, we can find<br />
sinθ and secθ .<br />
y 1 1 10 10<br />
sinθ<br />
= = = ⋅ =<br />
r 10 10 10 10<br />
r 10 10<br />
secθ<br />
= = = −<br />
x −3 3<br />
5. a. Because 210° lies between 180° and 270°, it is<br />
in quadrant III. The reference angle is<br />
θ ′ = 210°− 180°= 30°.<br />
b. Because 7 π 3π<br />
6π<br />
lies between = and<br />
4<br />
2 4<br />
8π<br />
2π = , it is in quadrant IV. The reference<br />
4<br />
7π 8π 7π π<br />
angle is θ′ = 2π<br />
− = − = .<br />
4 4 4 4<br />
c. Because –240° lies between –180° and –270°, it<br />
is in quadrant II. The reference angle is<br />
θ = 240 − 180 = 60°.<br />
d. Because 3.6 lies between π ≈ 3.14 and<br />
3π ≈ 4.71 , it is in quadrant III. The reference<br />
2<br />
angle is θ′ = 3.6 −π<br />
≈ 0.46 .<br />
6. a. 665°− 360°= 305°<br />
This angle is in quadrant IV, thus the reference<br />
angle is θ′ = 360°− 305°= 55°.<br />
b.<br />
c.<br />
15 π 15 8 7<br />
− 2π<br />
= π − π =<br />
π<br />
4 4 4 4<br />
This angle is in quadrant IV, thus the reference<br />
7π 8π 7π π<br />
angle is θ′ = 2π<br />
− = − = .<br />
4 4 4 4<br />
11 11 12<br />
− π + 2⋅ 2π<br />
= − π + π =<br />
π<br />
3 3 3 3<br />
This angle is in quadrant I, thus the reference<br />
π<br />
angle is θ′ = .<br />
3<br />
7. a. 300° lies in quadrant IV. The reference angle is<br />
θ ′ = 360°− 300°= 60°.<br />
b.<br />
3<br />
sin 60°=<br />
2<br />
Because the sine is negative in quadrant IV,<br />
3<br />
sin 300°=− sin 60°=− .<br />
2<br />
5π lies in quadrant III. The reference angle is<br />
4<br />
5 5 4<br />
θ′ = π − π = π − π = π .<br />
4 4 4 4<br />
π<br />
tan = 1<br />
4<br />
Because the tangent is positive in quadrant III,<br />
5π<br />
π<br />
tan =+ tan = 1 .<br />
4 4<br />
518<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
c.<br />
8. a.<br />
b.<br />
π<br />
− lies in quadrant IV. The reference angle is<br />
6<br />
π<br />
θ ′ = .<br />
6<br />
π 2 3<br />
sec = 6 3<br />
Because the secant is positive in quadrant IV,<br />
⎛ π ⎞ π 2 3<br />
sec⎜− ⎟= + sec = .<br />
⎝ 6 ⎠ 6 3<br />
17 π 17 12 5<br />
− 2π<br />
= π − π = π lies in quadrant<br />
6 6 6 6<br />
5π<br />
π<br />
II. The reference angle is θ′ = π − = .<br />
6 6<br />
The function value for the reference angle is<br />
π 3<br />
cos = . 6 2<br />
Because the cosine is negative in quadrant II,<br />
17π 5π π 3<br />
cos = cos =− cos = − .<br />
6 6 6 2<br />
−22 π −22 24 2<br />
+ 8π<br />
= π + π = π lies in<br />
3 3 3 3<br />
quadrant II. The reference angle is<br />
2π<br />
π<br />
θ′ = π − = .<br />
3 3<br />
The function value for the reference angle is<br />
π 3<br />
sin = . 3 2<br />
Because the sine is positive in quadrant II,<br />
−22π 2π π 3<br />
sin = sin = sin = .<br />
3 3 3 2<br />
Exercise Set 4.4<br />
1. We need values for x, y, and r. Because<br />
P = (–4, 3) is a point on the terminal side of<br />
θ , x =− 4 and y = 3. Furthermore,<br />
r x y<br />
that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y 3<br />
sinθ<br />
= =<br />
r 5<br />
x −4 4<br />
cosθ<br />
= = = −<br />
r 5 5<br />
y 3 3<br />
tanθ<br />
= = =−<br />
x −4 4<br />
r 5<br />
cscθ<br />
= =<br />
y 3<br />
2 2 2 2<br />
= + = ( − 4) + 3 = 16+ 9 = 25 = 5Now<br />
r 5 5<br />
secθ<br />
= = =−<br />
x −4 4<br />
x −4 4<br />
cotθ<br />
= = = −<br />
y 3 3<br />
2. We need values for x, y, and r, Because P = (–12, 5)<br />
is a point on the terminal side of<br />
θ , x =− 12 and y = 5. Furthermore,<br />
r x y<br />
2 2 2 2<br />
= + = − + = +<br />
( 12) 5 144 25<br />
= 169 = 13<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y 5<br />
sinθ<br />
= =<br />
r 13<br />
x −12 12<br />
cosθ<br />
= = = −<br />
r 13 13<br />
y 5 5<br />
tanθ<br />
= = = −<br />
x −12 12<br />
r 13<br />
cscθ<br />
= =<br />
y 5<br />
r 13 13<br />
secθ<br />
= = =−<br />
x −12 12<br />
x −12 12<br />
cotθ<br />
= = −<br />
y 5 5<br />
519<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
3. We need values for x, y, and r. Because P = (2, 3) is a<br />
point on the terminal side of θ , x = 2 and y = 3 .<br />
Furthermore,<br />
2 2 2 2<br />
r = x + y = 2 + 3 = 4+ 9 = 13<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y 3 3 13 3 13<br />
sinθ<br />
= = = ⋅ =<br />
r 13 13 13 13<br />
x 2 2 13 2 13<br />
cosθ<br />
= = = ⋅ =<br />
r 13 13 13 13<br />
y 3<br />
tanθ<br />
= =<br />
x 2<br />
r 13<br />
cscθ<br />
= =<br />
y 3<br />
r 13<br />
secθ<br />
= =<br />
x 2<br />
x 2<br />
cotθ<br />
= =<br />
y 3<br />
4. We need values for x, y, and r, Because<br />
P = (3, 7) is a point on the terminal side of<br />
θ , x = 3 and y = 7. Furthermore,<br />
2 2 2 2<br />
r = x + y = 3 + 7 = 9+ 49 = 58<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y 7 7 58 7 58<br />
sinθ<br />
= = = ⋅ =<br />
r 58 58 58 58<br />
x 3 3 58 3 58<br />
cosθ<br />
= = = ⋅ =<br />
r 58 58 58 58<br />
y 7<br />
tanθ<br />
= =<br />
x 3<br />
r 58<br />
cscθ<br />
= =<br />
y 7<br />
r 58<br />
secθ<br />
= =<br />
x 3<br />
x 3<br />
cotθ<br />
= =<br />
y 7<br />
5. We need values for x, y, and r. Because P = (3, –3) is<br />
a point on the terminal side of θ , x = 3 and y =− 3 .<br />
2 2 2 2<br />
Furthermore, r = x + y = 3 + ( − 3) = 9+<br />
9<br />
= 18 = 3 2<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y −3 −1 2 2<br />
sinθ<br />
= = = − ⋅ = −<br />
r 3 2 2 2 2<br />
x 3 1 2 2<br />
cosθ<br />
= = = ⋅ =<br />
r 3 2 2 2 2<br />
y −3<br />
tanθ<br />
= = = −1<br />
x 3<br />
r 3 2<br />
cscθ<br />
= = = − 2<br />
y −3<br />
r 3 2<br />
secθ<br />
= = = 2<br />
x 3<br />
x 3<br />
cotθ<br />
= = = −1<br />
y −3<br />
6. We need values for x, y, and r, Because P = (5, –5) is<br />
a point on the terminal side of θ , x = 5 and y = –5 .<br />
Furthermore,<br />
2 2 2<br />
r = x + y = 5 + ( − 5) = 25 + 25 = 50<br />
= 5 2<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y −5 −1 2 2<br />
sinθ<br />
= = = ⋅ =−<br />
r 5 2 2 2 2<br />
x 5 1 2 2<br />
cosθ<br />
= = = ⋅ =<br />
r 5 2 2 2 2<br />
y −5<br />
tanθ<br />
= = = −1<br />
x 5<br />
r 5 2<br />
cscθ<br />
= = = − 2<br />
y −5<br />
r 5 2<br />
secθ<br />
= = = 2<br />
x 5<br />
x 5<br />
cotθ<br />
= = =−1<br />
y −5<br />
520<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
7. We need values for x, y, and r. Because P = (–2, –5)<br />
is a point on the terminal side of<br />
θ , x =− 2 and y =− 5. Furthermore,<br />
r x y<br />
2 2 2 2<br />
= + = ( − 2) + ( − 5) = 4+ 25 = 29Now<br />
that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y −5 −5 29 5 29<br />
sinθ<br />
= = = ⋅ = −<br />
r 29 29 29 29<br />
x −2 −2 29 2 29<br />
cosθ<br />
= = = ⋅ =−<br />
r 29 29 29 29<br />
y −5 5<br />
tanθ<br />
= = =<br />
x −2 2<br />
r 29 29<br />
cscθ<br />
= = = −<br />
y −5 5<br />
r 29 29<br />
secθ<br />
= = =−<br />
x −2 2<br />
x −2 2<br />
cotθ<br />
= = =<br />
y −5 5<br />
10. θ = π radians<br />
The terminal side of the angle is on the negative x-<br />
axis. Select the point P = (–1, 0): x =− 1, y = 0, r = 1<br />
Apply the definition of the tangent function.<br />
y 0<br />
tanπ = = = 0<br />
x −1<br />
11. θ = π radians<br />
The terminal side of the angle is on the negative x-<br />
axis. Select the point P = (–1, 0):<br />
x =− 1, y = 0, r = 1 Apply the definition of the secant<br />
function.<br />
r 1<br />
secπ = = = −1<br />
x −1<br />
12. θ = π radians<br />
The terminal side of the angle is on the negative x-<br />
axis. Select the point P = (–1, 0): x =− 1, y = 0, r = 1<br />
Apply the definition of the cosecant function.<br />
r 1<br />
csc π = = , undefined<br />
y 0<br />
8. We need values for x, y, and r, Because P = (–1, –3)<br />
is a point on the terminal side of<br />
θ , x = –1 and y = –3 . Furthermore,<br />
r x y<br />
2 2 2 2<br />
= + = − + − = + =<br />
( 1) ( 3) 1 9 10<br />
Now that we know x, y, and r, we can find the six<br />
trigonometric functions of θ .<br />
y −3 −3 10 3 10<br />
sinθ<br />
= = = ⋅ = −<br />
r 10 10 10 10<br />
x −1 −1 10 10<br />
cosθ<br />
= = = ⋅ = −<br />
r 10 10 10 10<br />
y −3<br />
tanθ<br />
= = = 3<br />
x −1<br />
r 10 10<br />
cscθ<br />
= = = −<br />
y −3 3<br />
r 10<br />
secθ<br />
= = =− 10<br />
x −1<br />
x −1 1<br />
cotθ<br />
= = =<br />
y −3 3<br />
9. θ = π radians<br />
The terminal side of the angle is on the negative x-<br />
axis. Select the point P = (–1, 0):<br />
x =− 1, y = 0, r = 1 Apply the definition of the<br />
cosine function.<br />
x −1<br />
cosπ<br />
= = = − 1<br />
r 1<br />
13.<br />
14.<br />
15.<br />
3π<br />
θ = radians<br />
2<br />
The terminal side of the angle is on the negative y-<br />
axis. Select the point P = (0, –1):<br />
x = 0, y = –1, r = 1 Apply the definition of the<br />
3π<br />
y −1<br />
tangent function. tan = = , undefined<br />
2 x 0<br />
3π<br />
θ = radians<br />
2<br />
The terminal side of the angle is on the negative y-<br />
axis. Select the point P = (0, –1): x = 0, y = − 1, r = 1<br />
Apply the definition of the cosine function.<br />
3π x 0<br />
cos = = = 0<br />
2 r 1<br />
π<br />
θ = radians<br />
2<br />
The terminal side of the angle is on the positive y-<br />
axis. Select the point P = (0, 1):<br />
x = 0, y = 1, r = 1 Apply the definition of the<br />
π x 0<br />
cotangent function. cot = = = 0<br />
2 y 1<br />
521<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
16.<br />
π<br />
θ = radians<br />
2<br />
The terminal side of the angle is on the positive y-<br />
axis. Select the point P = (0, 1): x = 0, y = 1, r = 1<br />
Apply the definition of the tangent function.<br />
π y 1<br />
tan = = , undefined<br />
2 x 0<br />
17. Because sinθ<br />
> 0, θ cannot lie in quadrant III or<br />
quadrant IV; the sine function is negative in those<br />
quadrants. Thus, with sinθ<br />
> 0, θ lies in quadrant I<br />
or quadrant II. We are also given that cosθ > 0 .<br />
Because quadrant I is the only quadrant in which the<br />
cosine is positive and sine is positive, we conclude<br />
that θ lies in quadrant I.<br />
18. Because sinθ<br />
< 0, θ cannot lie in quadrant I or<br />
quadrant II; the sine function is positive in those two<br />
quadrants. Thus, with sinθ<br />
< 0, θ lies in quadrant III<br />
or quadrant IV. We are also given that cosθ > 0.<br />
Because quadrant IV is the only quadrant in which<br />
the cosine is positive and the sine is negative, we<br />
conclude that θ lies in quadrant IV.<br />
19. Because sinθ<br />
< 0, θ cannot lie in quadrant I or<br />
quadrant II; the sine function is positive in those two<br />
quadrants. Thus, with sinθ < 0, θ lies in quadrant<br />
III or quadrant IV. We are also given that cosθ < 0 .<br />
Because quadrant III is the only quadrant in which<br />
the cosine is positive and the sine is negative, we<br />
conclude that θ lies in quadrant III.<br />
20. Because tanθ<br />
< 0, θ cannot lie in quadrant I or<br />
quadrant III; the tangent function is positive in those<br />
two quadrants. Thus, with tanθ<br />
< 0, θ lies in<br />
quadrant II or quadrant IV. We are also given that<br />
sinθ < 0 . Because quadrant IV is the only quadrant<br />
in which the sine is negative and the tangent is<br />
negative, we conclude that θ lies in quadrant IV.<br />
21. Because tanθ<br />
< 0, θ cannot lie in quadrant I or<br />
quadrant III; the tangent function is positive in those<br />
quadrants. Thus, with tanθ<br />
< 0, θ lies in quadrant II<br />
or quadrant IV. We are also given that cosθ < 0 .<br />
Because quadrant II is the only quadrant in which the<br />
cosine is negative and the tangent is negative, we<br />
conclude that θ lies in quadrant II.<br />
22. Because cotθ<br />
> 0, θ cannot lie in quadrant II or<br />
quadrant IV; the cotangent function is negative in<br />
those two quadrants. Thus, with cotθ<br />
> 0, θ lies in<br />
quadrant I or quadrant III. We are also given that<br />
secθ < 0 . Because quadrant III is the only quadrant<br />
in which the secant is negative and the cotangent is<br />
positive, we conclude that θ lies in quadrant III.<br />
23. In quadrant III x is negative and y is negative. Thus,<br />
cos = 3 x −3<br />
θ − = = , x = –3, r = 5. Furthermore,<br />
5 r 5<br />
2 2 2<br />
r = x + y<br />
2 2 2<br />
5 = ( − 3) + y<br />
2<br />
y = 25 − 9 = 16<br />
y =− 16 =−4<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −4 4<br />
sinθ<br />
= = = −<br />
r 5 5<br />
y −4 4<br />
tanθ<br />
= = =<br />
x −3 3<br />
r 5 5<br />
cscθ<br />
= = = −<br />
y −4 4<br />
r 5 5<br />
secθ<br />
= = = −<br />
x −3 3<br />
x −3 3<br />
cotθ<br />
= = =<br />
y −4 4<br />
24. In quadrant III, x is negative and y is negative. Thus,<br />
12 y −12<br />
sin θ =− = = , y =− 12, r = 13 .<br />
13 r 13<br />
Furthermore,<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
x + ( − 12) = 13<br />
2<br />
x = 169 − 144 = 25<br />
x =− 25 =−5<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
x −5 5<br />
cosθ<br />
= = = −<br />
r 13 13<br />
y −12 12<br />
tanθ<br />
= = =<br />
x −5 5<br />
r 13 13<br />
cscθ<br />
= = = −<br />
y −12 12<br />
r 13 13<br />
secθ<br />
= = =−<br />
x −5 5<br />
x −5 5<br />
cotθ<br />
= = =<br />
y −12 12<br />
522<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
25. In quadrant II x is negative and y is positive. Thus,<br />
5 y<br />
sin θ = = , y = 5, r = 13 . Furthermore,<br />
13 r<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
x + 5 = 13<br />
2<br />
x = 169 − 25 = 144<br />
x =− 144 =−12<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
x −12 12<br />
cosθ<br />
= = = −<br />
r 13 13<br />
y 5 5<br />
tanθ<br />
= = = −<br />
x −12 12<br />
r 13<br />
cscθ<br />
= =<br />
y 5<br />
r 13 13<br />
secθ<br />
= = = −<br />
x −12 12<br />
x −12 12<br />
cotθ<br />
= = =−<br />
y 5 5<br />
26. In quadrant IV, x is positive and y is negative. Thus,<br />
4 x<br />
cos θ = = , x = 4, r = 5 . Furthermore,<br />
5 r<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
4 + y = 5<br />
2<br />
y = 25 − 16 = 9<br />
y =− 9 =−3<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −3 3<br />
sinθ<br />
= = = −<br />
r 5 5<br />
y −3 3<br />
tanθ<br />
= = = −<br />
x 4 4<br />
r 5 5<br />
cscθ<br />
= = =−<br />
y −3 3<br />
r 5<br />
secθ<br />
= =<br />
x 4<br />
x 4 4<br />
cotθ<br />
= = = −<br />
y −3 3<br />
27. Because 270°< θ < 360 ° , θ is in quadrant IV. In<br />
quadrant IV x is positive and y is negative. Thus,<br />
8 x<br />
cos θ = = , x = 8,<br />
17 r<br />
r = 17. Furthermore<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
8 + y = 17<br />
2<br />
y = 289 − 64 = 225<br />
y =− 225 =−15<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −15 15<br />
sinθ<br />
= = = −<br />
r 17 17<br />
y −15 15<br />
tanθ<br />
= = = −<br />
x 8 8<br />
r 17 17<br />
cscθ<br />
= = =−<br />
y −15 15<br />
r 17<br />
secθ<br />
= =<br />
x 8<br />
x 8 8<br />
cotθ<br />
= = = −<br />
y −15 15<br />
28. Because 270°< θ < 360 ° , θ is in quadrant IV. In<br />
quadrant IV, x is positive and y is negative. Thus,<br />
1 x<br />
cos θ = = , x = 1, r = 3. Furthermore,<br />
3 r<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
1 + y = 3<br />
2<br />
y = 9− 1=<br />
8<br />
y =− 8 =−2 2<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −2 2 2 2<br />
sinθ<br />
= = =−<br />
r 3 3<br />
y −2 2<br />
tanθ<br />
= = = −2 2<br />
x 1<br />
r 3 3 2 3 2<br />
cscθ<br />
= = = ⋅ = −<br />
y −2 2 −2 2 2 4<br />
r 3<br />
secθ<br />
= = = 3<br />
x 1<br />
x 1 1 2 2<br />
cotθ<br />
= = = ⋅ =−<br />
y −2 2 − 2 2 2 4<br />
523<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
29. Because the tangent is negative and the sine is<br />
positive, θ lies in quadrant II. In quadrant II, x is<br />
negative and y is positive. Thus,<br />
2 y 2<br />
tan θ = − = = , x = − 3, y = 2. Furthermore,<br />
3 x −3<br />
r x y<br />
2 2 2 2<br />
= + = − + = + =<br />
( 3) 2 9 4 13<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y 2 2 13 2 13<br />
sinθ<br />
= = = ⋅ =<br />
r 13 13 13 13<br />
x −3 −3 13 3 13<br />
cosθ<br />
= = = ⋅ = −<br />
r 13 13 13 13<br />
r 13<br />
cscθ<br />
= =<br />
y 2<br />
r 13 13<br />
secθ<br />
= = = −<br />
x −3 3<br />
x −3 3<br />
cotθ<br />
= = = −<br />
y 2 2<br />
30. Because the tangent is negative and the sine is<br />
positive, θ lies in quadrant II. In quadrant II, x is<br />
negative and y is positive. Thus,<br />
1 y 1<br />
tan θ =− = = , y = 1, x =−3<br />
. Furthermore,<br />
3 x −3<br />
r x y<br />
2 2 2 2<br />
= + = − + = + =<br />
( 3) 1 9 1 10<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y 1 1 10 10<br />
sinθ<br />
= = = ⋅ =<br />
r 10 10 10 10<br />
x −3 −3 10 3 10<br />
cosθ<br />
= = = ⋅ = −<br />
r 10 10 10 10<br />
r 10<br />
cscθ<br />
= = = 10<br />
y 1<br />
r 10 10<br />
secθ<br />
= = = −<br />
x −3 3<br />
x −3<br />
cotθ<br />
= = =−3<br />
y 1<br />
31. Because the tangent is positive and the cosine is<br />
negative, θ lies in quadrant III. In quadrant III, x is<br />
4 y −4<br />
negative and y is negative. Thus, tan θ = = = ,<br />
3 x − 3<br />
x = –3, y = –4. Furthermore,<br />
2 2 2 2<br />
r = x + y = ( − 3) + ( − 4) = 9+<br />
16<br />
= 25 = 5<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −4 4<br />
sinθ<br />
= = = −<br />
r 5 5<br />
x −3 3<br />
cosθ<br />
= = =−<br />
r 5 5<br />
r 5 5<br />
cscθ<br />
= = = −<br />
y −4 4<br />
r 5 5<br />
secθ<br />
= = = −<br />
x −3 3<br />
x −3 3<br />
cotθ<br />
= = =<br />
y −4 4<br />
32. Because the tangent is positive and the cosine is<br />
negative, θ lies in quadrant III. In quadrant III, x is<br />
negative and y is negative. Thus,<br />
5 y −5<br />
tan θ = = = , x =− 12, y =−5<br />
. Furthermore,<br />
12 x −12<br />
2 2 2 2<br />
r = x + y = ( − 12) + ( − 5) = 144+<br />
25<br />
= 169 = 13<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −5 5<br />
sinθ<br />
= = =−<br />
r 13 13<br />
x −12 12<br />
cosθ<br />
= = = −<br />
r 13 13<br />
r 13 13<br />
cscθ<br />
= = = −<br />
y −5 5<br />
r 13 13<br />
secθ<br />
= = = −<br />
x −12 12<br />
x −12 12<br />
cotθ<br />
= = =<br />
y −5 5<br />
524<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
33. Because the secant is negative and the tangent is<br />
positive, θ lies in quadrant III. In quadrant III, x is<br />
negative and y is negative. Thus,<br />
r 3<br />
secθ =− 3 = = , x =− 1, r = 3 . Furthermore,<br />
x −1<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
( − 1) + y = 3<br />
2<br />
y = 9− 1=<br />
8<br />
y =− 8 =−2 2<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −2 2 2 2<br />
sinθ<br />
= = =−<br />
r 3 3<br />
x −1 1<br />
cosθ<br />
= = =−<br />
r 3 3<br />
y −2 2<br />
tanθ<br />
= = = 2 2<br />
x −1<br />
r 3 3 2 3 2<br />
cscθ<br />
= = = ⋅ = −<br />
y −2 2 −2 2 2 4<br />
x −1 1 2 2<br />
cotθ<br />
= = = ⋅ =<br />
y − 2 2 2 2 2 4<br />
34. Because the cosecant is negative and the tangent is<br />
positive, θ lies in quadrant III. In quadrant III, x is<br />
negative and y is negative. Thus,<br />
r 4<br />
cscθ =− 4 = = , y =− 1, r = 4 . Furthermore,<br />
y −1<br />
2 2 2<br />
x + y = r<br />
2 2 2<br />
x + ( − 1) = 4<br />
2<br />
x = 16 − 1 = 15<br />
x =− 15<br />
Now that we know x, y, and r, we can find the<br />
remaining trigonometric functions of θ .<br />
y −1 1<br />
sinθ<br />
= = = −<br />
r 4 4<br />
x − 15 15<br />
cosθ<br />
= = = −<br />
r 4 4<br />
y −1 1 15 15<br />
tanθ<br />
= = = ⋅ =<br />
x − 15 15 15 15<br />
r 4 4 15 4 15<br />
secθ<br />
= = = − ⋅ = −<br />
x − 15 15 15 15<br />
x − 15<br />
cotθ<br />
= = = 15<br />
y − 1<br />
35. Because 160° lies between 90° and 180°, it is in<br />
quadrant II. The reference angle is<br />
θ ′ = 180°− 160°= 20°.<br />
36. Because 170° lies between 90° and 180°, it is in<br />
quadrant II. The reference angle is<br />
θ ′ = 180°− 170°= 10°.<br />
37. Because 205° lies between 180° and 270°, it is in<br />
quadrant III. The reference angle is<br />
θ ′ = 205°− 180°= 25°.<br />
38. Because 210° lies between 180° and 270°, it is in<br />
quadrant III. The reference angle is<br />
θ ′ = 210°− 180°= 30°.<br />
39. Because 355° lies between 270° and 360°, it is in<br />
quadrant IV. The reference angle is<br />
θ ′ = 360°− 355°= 5°.<br />
40. Because 351° lies between 270° and 360°, it is in<br />
quadrant IV. The reference angle is<br />
θ ′ = 360°− 351°= 9°.<br />
41. Because 7 π 3π<br />
6π<br />
8π<br />
lies between = and 2π = , it<br />
4<br />
2 4<br />
4<br />
is in quadrant IV. The reference angle is<br />
7π 8π 7π π<br />
θ′ = 2π<br />
− = − = .<br />
4 4 4 4<br />
42. Because 5π 4 lies between π = 4π 4 and 3π 2 = 6π 4 , it<br />
is in quadrant III. The reference angle is<br />
θ ′ = 5π 4 − π = 5π 4 − 4π 4 = π 4 .<br />
43. Because 5 π π 3π<br />
lies between = and π =<br />
6<br />
2 6<br />
in quadrant II. The reference angle is<br />
5π 6π 5π π<br />
θ′ = π − = − = .<br />
6 6 6 6<br />
6π<br />
6<br />
, it is<br />
44. Because 5 π 10 π<br />
π 7π<br />
= lies between = and<br />
7 14<br />
2 14<br />
14π<br />
π = , it is in quadrant II. The reference angle is<br />
14<br />
5π 7π 5π 2π<br />
θ′ = π − = − = .<br />
7 7 7 7<br />
45. − 150°+ 360°= 210°<br />
Because the angle is in quadrant III, the reference<br />
angle is θ ′ = 210°− 180°= 30°.<br />
525<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
46. − 250°+ 360°= 110°<br />
Because the angle is in quadrant II, the reference<br />
angle is θ′ = 180°− 110°= 70°.<br />
47. − 335°+ 360°= 25°<br />
Because the angle is in quadrant I, the reference<br />
angle is θ′ = 25°.<br />
48. − 359°+ 360°= 1°<br />
Because the angle is in quadrant I, the reference<br />
angle is θ′ = 1°.<br />
57.<br />
58.<br />
11 11 16 5<br />
− π + 4π<br />
= − π + π =<br />
π<br />
4 4 4 4<br />
Because the angle is in quadrant III, the reference<br />
5π<br />
π<br />
angle is θ′ = − π = .<br />
4 4<br />
17 17 24 7<br />
− π + 4π<br />
=− π + π =<br />
π<br />
6 6 6 6<br />
Because the angle is in quadrant III, the reference<br />
7π<br />
π<br />
angle is θ′ = − π = .<br />
6 6<br />
49. Because 4.7 lies between π ≈ 3.14 and 3 π ≈ 4.71 , it<br />
2<br />
is in quadrant III. The reference angle is<br />
θ′ = 4.7 −π<br />
≈ 1.56 .<br />
50. Because 5.5 lies between 3 π ≈ 4.71 and 2π ≈ 6.28 ,<br />
2<br />
it is in quadrant IV. The reference angle is<br />
θ′ = 2π<br />
−5.5 ≈ 0.78 .<br />
51. 565°− 360°= 205°<br />
Because the angle is in quadrant III, the reference<br />
angle is θ ′ = 205°− 180°= 25°.<br />
52. 553°− 360°= 193°<br />
Because the angle is in quadrant III, the reference<br />
angle is θ′ = 193°− 180°= 13°.<br />
53.<br />
54.<br />
55.<br />
56.<br />
17 π 17 12 5<br />
− 2π<br />
= π − π =<br />
π<br />
6 6 6 6<br />
Because the angle is in quadrant II, the reference<br />
5π<br />
π<br />
angle is θ′ = π − = .<br />
6 6<br />
11 π 11 8 3<br />
− 2π<br />
= π − π =<br />
π<br />
4 4 4 4<br />
Because the angle is in quadrant II, the reference<br />
3π<br />
π<br />
angle is θ′ = π − = .<br />
4 4<br />
23 π 23 16 7<br />
− 4π<br />
= π − π =<br />
π<br />
4 4 4 4<br />
Because the angle is in quadrant IV, the reference<br />
7π<br />
π<br />
angle is θ′ = 2π<br />
− = .<br />
4 4<br />
17 π 17 12 5<br />
− 4π<br />
= π − π =<br />
π<br />
3 3 3 3<br />
Because the angle is in quadrant IV, the reference<br />
5π<br />
π<br />
angle is θ′ = 2π<br />
− = .<br />
3 3<br />
59.<br />
60.<br />
25 25 36 11<br />
− π + 6π<br />
=− π + π =<br />
π<br />
6 6 6 6<br />
Because the angle is in quadrant IV, the reference<br />
11π<br />
π<br />
angle is θ′ = 2π<br />
− = .<br />
6 6<br />
13 13 18 5<br />
− π + 6π<br />
= − π + π =<br />
π<br />
3 3 3 3<br />
Because the angle is in quadrant IV, the reference<br />
5π<br />
π<br />
angle is θ′ = 2π<br />
− = .<br />
3 3<br />
61. 225° lies in quadrant III. The reference angle is<br />
θ ′ = 225°− 180°= 45°.<br />
2<br />
cos 45°=<br />
2<br />
Because the cosine is negative in quadrant III,<br />
2<br />
cos 225 ° = − cos 45 ° = − .<br />
2<br />
62. 300° lies in quadrant IV. The reference angle is<br />
θ ′ = 360°− 300°= 60°<br />
.<br />
3<br />
sin 60°=<br />
2<br />
Because the sine is negative in quadrant IV,<br />
3<br />
sin 300°=− sin 60°=− .<br />
2<br />
63. 210° lies in quadrant III. The reference angle is<br />
210 180 30<br />
θ ′ = °− °= °.<br />
3<br />
tan 30°=<br />
3<br />
Because the tangent is positive in quadrant III,<br />
3<br />
tan 210 ° = tan 30°= .<br />
3<br />
526<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
64. 240° lies in quadrant III. The reference angle is<br />
θ ′ = 240°− 180°= 60°<br />
.<br />
sec60°=<br />
2<br />
Because the secant is negative in quadrant III,<br />
sec 240°=− sec60°− 2 .<br />
65. 420° lies in quadrant I. The reference angle is<br />
420 360 60<br />
θ ′ = °− °= °.<br />
tan 60°=<br />
3<br />
Because the tangent is positive in quadrant I,<br />
tan 420 ° = tan 60 ° = 3 .<br />
66. 405° lies in quadrant I. The reference angle is<br />
θ ′ = 405°− 360°= 45°<br />
.<br />
tan 45°=<br />
1<br />
Because the tangent is positive in quadrant I,<br />
tan 405 ° =tan45 ° =1.<br />
67.<br />
2π lies in quadrant II. The reference angle is<br />
3<br />
2π 3π 2π π<br />
θ′ = π − = − = .<br />
3 3 3 3<br />
π 3<br />
sin = 3 2<br />
Because the sine is positive in quadrant II,<br />
2π π 3<br />
sin =sin = .<br />
3 3 2<br />
70.<br />
71.<br />
72.<br />
7π lies in quadrant IV. The reference angle is<br />
4<br />
7π 8π 7π π<br />
θ′ = 2 π − = − = .<br />
4 4 4 4<br />
π<br />
cot = 1<br />
4<br />
Because the cotangent is negative in quadrant IV,<br />
7π<br />
π<br />
cot = − cot = − 1 .<br />
4 4<br />
9π lies in quadrant I. The reference angle is<br />
4<br />
9 9 8<br />
θ′ = π − 2π<br />
= π − π = π .<br />
4 4 4 4<br />
π<br />
tan = 1<br />
4<br />
Because the tangent is positive in quadrant I,<br />
9π π<br />
tan =tan = 1<br />
4 4<br />
9π lies on the positive y-axis. The reference angle is<br />
2<br />
9 9 8<br />
θ′ = π − 4π<br />
= π − π = π .<br />
2 2 2 2<br />
π 9π Because tan is undefined, tan is also<br />
2 2<br />
undefined.<br />
68.<br />
69.<br />
3π lies in quadrant II. The reference angle is<br />
4<br />
3π 4π 3π π<br />
θ′ = π − = − = .<br />
4 4 4 4<br />
π 2<br />
cos = 4 2<br />
Because the cosine is negative in quadrant II,<br />
3π π 2<br />
cos =– cos =− .<br />
4 4 2<br />
7π lies in quadrant III. The reference angle is<br />
6<br />
7 7 6<br />
θ′ = π − π = π − π = π .<br />
6 6 6 6<br />
π<br />
csc = 2<br />
6<br />
Because the cosecant is negative in quadrant III,<br />
7π<br />
π<br />
csc = − csc =− 2 .<br />
6 6<br />
73. –240° lies in quadrant II. The reference angle is<br />
240 180 60<br />
θ ′ = °− °= °.<br />
3<br />
sin 60°=<br />
2<br />
Because the sine is positive in quadrant II,<br />
3<br />
sin( − 240 ° )= sin 60 ° = . 2<br />
74. –225° lies in quadrant II. The reference angle is<br />
θ ′ = 225°− 180°= 45 ° .<br />
2<br />
sin 45°=<br />
2<br />
Because the sine is positive in quadrant II,<br />
2<br />
sin( − 225 ° ) = sin 45°= .<br />
2<br />
527<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
75.<br />
76.<br />
π<br />
− lies in quadrant IV. The reference angle is<br />
4<br />
π<br />
θ ′ = .<br />
4<br />
π<br />
tan = 1<br />
4<br />
Because the tangent is negative in quadrant IV,<br />
⎛ π ⎞ π<br />
tan ⎜− ⎟= − tan =−1<br />
⎝ 4 ⎠ 4<br />
π<br />
− lies in quadrant IV. The reference angle is<br />
6<br />
π π 3<br />
θ = . tan =<br />
6 6 3<br />
Because the tangent is negative in quadrant IV,<br />
⎛ π ⎞ 3<br />
tan ⎜− ⎟ =− .<br />
⎝ 6 ⎠ 3<br />
77. sec 495°= sec135°=−<br />
2<br />
78.<br />
79.<br />
80.<br />
2 3<br />
sec510°= sec150°=−<br />
3<br />
19π<br />
7π<br />
cot = cot = 3<br />
6 6<br />
13π<br />
π 3<br />
cot = cot =<br />
3 3 3<br />
87.<br />
π π 3π<br />
sin cosπ − cos sin<br />
3 3 2<br />
⎛ 3 ⎞ ⎛1⎞<br />
= ( −1) − ( −1<br />
⎜<br />
)<br />
2 ⎟ ⎜<br />
2<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
3 1<br />
=− +<br />
2 2<br />
1−<br />
3<br />
=<br />
2<br />
π π<br />
88. sin cos 0 − sin cosπ<br />
4 6<br />
⎛ 2 ⎞ ⎛1⎞<br />
= () 1 − ( −1<br />
⎜<br />
)<br />
2 ⎟ ⎜<br />
2<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
2 1<br />
= +<br />
2 2<br />
2 + 1<br />
=<br />
2<br />
89.<br />
11π 5π 11π 5π<br />
sin cos + cos sin<br />
4 6 4 6<br />
⎛ 2 ⎞⎛ 3 ⎞ ⎛ 2 ⎞⎛1⎞<br />
= − + −<br />
⎜ 2 ⎟⎜ 2 ⎟ ⎜ 2<br />
⎟⎜⎟ 2<br />
⎟<br />
⎝ ⎠⎝ ⎠ ⎝ ⎠ ⎝ ⎠<br />
6 2<br />
=− −<br />
4 4<br />
6 + 2<br />
=−<br />
4<br />
81.<br />
82.<br />
83.<br />
84.<br />
23π<br />
7π<br />
2<br />
cos = cos =<br />
4 4 2<br />
35π<br />
11π<br />
3<br />
cos = cos =<br />
6 6 2<br />
⎛ 17π<br />
⎞ 7π<br />
3<br />
tan ⎜− tan<br />
6<br />
⎟= =<br />
⎝ ⎠ 6 3<br />
⎛ 11π<br />
⎞ π<br />
tan ⎜− = tan = 1<br />
4<br />
⎟<br />
⎝ ⎠ 4<br />
90.<br />
17π 5π 17π 5π<br />
sin cos + cos sin<br />
3 4 3 4<br />
⎛ 3 ⎞⎛ 2 ⎞ ⎛1⎞⎛ 2 ⎞<br />
= − − + −<br />
⎜ 2 ⎟⎜ 2 ⎟ ⎜<br />
2<br />
⎟<br />
⎜ 2 ⎟<br />
⎝ ⎠⎝ ⎠ ⎝ ⎠⎝ ⎠<br />
6 2<br />
= −<br />
4 4<br />
6 − 2<br />
=<br />
4<br />
85.<br />
⎛ 17π<br />
⎞ π 3<br />
sin ⎜− sin<br />
3<br />
⎟ = =<br />
⎝ ⎠ 3 2<br />
86.<br />
⎛ 35π<br />
⎞ π 1<br />
sin ⎜− sin<br />
6<br />
⎟ = =<br />
⎝ ⎠ 6 2<br />
528<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
PreCalculus 4E Section 4.4<br />
91.<br />
92.<br />
93.<br />
94.<br />
3 15 5<br />
sin π ⎛<br />
tan π ⎞ ⎛<br />
cos<br />
π ⎞<br />
2<br />
⎜− − −<br />
4<br />
⎟ ⎜<br />
3<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
⎛1<br />
⎞<br />
= ( −1)( 1)<br />
− ⎜<br />
2 ⎟<br />
⎝ ⎠<br />
1<br />
=−1−<br />
2<br />
2 1<br />
=− −<br />
2 2<br />
3<br />
=−<br />
2<br />
3 8 5<br />
sin π ⎛<br />
tan π ⎞ ⎛<br />
cos<br />
π ⎞<br />
2<br />
⎜− + −<br />
3<br />
⎟ ⎜<br />
6<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
⎛ 3 ⎞<br />
= ( − 1)( 3)<br />
+ ⎜<br />
−<br />
2 ⎟<br />
⎝ ⎠<br />
3<br />
=− 3 −<br />
2<br />
2 3 3<br />
=− −<br />
2 2<br />
3 3<br />
=−<br />
2<br />
⎛4 4<br />
f π π ⎞ ⎛<br />
f π ⎞ ⎛<br />
f<br />
π ⎞<br />
⎜ +<br />
3 6<br />
⎟+ ⎜ +<br />
3<br />
⎟ ⎜<br />
6<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ ⎝ ⎠<br />
⎛4π π ⎞ 4π π<br />
= sin ⎜ + sin sin<br />
3 6<br />
⎟+ +<br />
⎝ ⎠ 3 6<br />
3π 4π π<br />
= sin + sin + sin<br />
2 3 6<br />
⎛ 3 ⎞ ⎛1⎞<br />
= ( − 1)<br />
+ − +<br />
⎜<br />
2 ⎟ ⎜<br />
2<br />
⎟<br />
⎝ ⎠ ⎝ ⎠<br />
3 + 1<br />
=−<br />
2<br />
⎛5 5<br />
g π π ⎞ ⎛<br />
g π ⎞ ⎛<br />
g<br />
π ⎞<br />
⎜ +<br />
6 6<br />
⎟+ ⎜ +<br />
6<br />
⎟ ⎜<br />
6<br />
⎟<br />
⎝ ⎠ ⎝ ⎠ ⎝ ⎠<br />
⎛5π π ⎞ 5π π<br />
= cos ⎜ + cos cos<br />
6 6<br />
⎟+ +<br />
⎝ ⎠ 6 6<br />
5π<br />
π<br />
= cosπ<br />
+ cos + cos<br />
6 6<br />
⎛ 3 ⎞ ⎛ 3 ⎞<br />
= ( − 1)<br />
+ − +<br />
⎜ 2 ⎟ ⎜ 2 ⎟<br />
⎝ ⎠ ⎝ ⎠<br />
=−1<br />
95. ( )<br />
⎛17π<br />
⎞ ⎛ ⎛17π<br />
⎞⎞<br />
h g ⎜ = h⎜g<br />
⎟<br />
3<br />
⎟ ⎜<br />
3<br />
⎟<br />
⎝ ⎠ ⎝ ⎝ ⎠⎠<br />
⎛ ⎛17π<br />
⎞⎞<br />
= 2⎜cos⎜<br />
3<br />
⎟⎟<br />
⎝ ⎝ ⎠⎠<br />
⎛1<br />
⎞<br />
= 2 ⎜ 2 ⎟<br />
⎝ ⎠<br />
= 1<br />
⎛11π<br />
⎞ ⎛ ⎛11π<br />
⎞⎞<br />
h f ⎜ = h⎜ f ⎟<br />
4<br />
⎟ ⎜<br />
4<br />
⎟<br />
⎝ ⎠ ⎝ ⎝ ⎠⎠<br />
⎛ ⎛11π<br />
⎞⎞<br />
= 2⎜sin⎜<br />
⎟<br />
4<br />
⎟<br />
⎝ ⎝ ⎠⎠<br />
96. ( )<br />
⎛ 2 ⎞<br />
= 2<br />
⎜<br />
2 ⎟<br />
⎝ ⎠<br />
= 2<br />
97. The average rate of change is the slope of the line<br />
, ( ) x , f( x )<br />
through the points ( x f x ) and ( )<br />
m =<br />
f( x2) − f( x1)<br />
x − x<br />
2 1<br />
1 1<br />
⎛3π<br />
⎞ ⎛5π<br />
⎞<br />
sin ⎜ sin<br />
2<br />
⎟−<br />
⎜<br />
4<br />
⎟<br />
=<br />
⎝ ⎠ ⎝ ⎠<br />
3π<br />
5π<br />
−<br />
2 4<br />
⎛ 2 ⎞<br />
−1− −<br />
⎜<br />
2 ⎟<br />
=<br />
⎝ ⎠<br />
π<br />
4<br />
2<br />
− 1+<br />
= 2<br />
π<br />
4<br />
⎛ 2 ⎞<br />
4 − 1+<br />
⎜<br />
2 ⎟<br />
=<br />
⎝ ⎠<br />
⎛π<br />
⎞<br />
4⎜<br />
4<br />
⎟<br />
⎝ ⎠<br />
2 2 − 4<br />
=<br />
π<br />
2 2<br />
529<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.
<strong>Trigonometric</strong> <strong>Functions</strong><br />
98. The average rate of change is the slope of the line<br />
through the points ( x1, g( x<br />
1)<br />
) and ( x2, g( x<br />
2)<br />
)<br />
gx (<br />
2) − gx (<br />
1)<br />
m =<br />
x2 − x1<br />
⎛3π<br />
⎞<br />
cos ( π ) − cos ⎜<br />
4<br />
⎟<br />
=<br />
⎝ ⎠<br />
3π<br />
π −<br />
4<br />
⎛ 2 ⎞<br />
−1− ⎜<br />
−<br />
2 ⎟<br />
=<br />
⎝ ⎠<br />
π<br />
4<br />
⎛ 2 ⎞<br />
4 − 1+<br />
⎜<br />
2 ⎟<br />
=<br />
⎝ ⎠<br />
⎛π<br />
⎞<br />
4⎜<br />
4<br />
⎟<br />
⎝ ⎠<br />
2 2 − 4<br />
=<br />
π<br />
99.<br />
2<br />
π<br />
sinθ = when the reference angle is and θ is<br />
2<br />
4<br />
in quadrants I or II.<br />
QI<br />
QII<br />
π<br />
π<br />
θ = θ = π −<br />
4 4<br />
3π<br />
=<br />
4<br />
π 3π<br />
θ = ,<br />
4 4<br />
1<br />
π<br />
100. cosθ = when the reference angle is and θ is in<br />
2<br />
3<br />
quadrants I or IV.<br />
QI<br />
QIV<br />
π<br />
π<br />
θ = θ = 2π<br />
−<br />
3 3<br />
5π<br />
=<br />
3<br />
π 5π<br />
θ = ,<br />
3 3<br />
2<br />
π<br />
101. sinθ =− when the reference angle is and<br />
2<br />
4<br />
θ is in quadrants III or IV.<br />
QIII<br />
QIV<br />
π<br />
π<br />
θ = π + θ = 2π<br />
−<br />
4 4<br />
5π<br />
7π<br />
= =<br />
4 4<br />
5π<br />
7π<br />
θ = ,<br />
4 4<br />
1<br />
π<br />
102. cosθ =− when the reference angle is and θ is<br />
2<br />
3<br />
in quadrants II or III.<br />
QII<br />
QIII<br />
π<br />
π<br />
θ = π − θ = π +<br />
3 3<br />
2π<br />
4π<br />
= =<br />
3 3<br />
2π<br />
4π<br />
θ = ,<br />
3 3<br />
π<br />
103. tanθ =− 3 when the reference angle is and θ is 3<br />
in quadrants II or IV.<br />
QII<br />
QIV<br />
π<br />
π<br />
θ = π − θ = 2π<br />
−<br />
3 3<br />
2π<br />
5π<br />
= =<br />
3 3<br />
2π<br />
5π<br />
θ = ,<br />
3 3<br />
3<br />
π<br />
104. tanθ =− when the reference angle is and<br />
3<br />
6<br />
θ is in quadrants II or IV.<br />
QII<br />
QIV<br />
π<br />
π<br />
θ = π − θ = 2π<br />
−<br />
6 6<br />
5π<br />
11π<br />
= =<br />
6 6<br />
5π<br />
11π<br />
θ = ,<br />
6 6<br />
105. – 109. Answers may vary.<br />
110. does not make sense; Explanations will vary.<br />
Sample explanation: Sine is defined for all values of<br />
the angle.<br />
530<br />
Copyright © 2010 Pearson Education, Inc. Publishing as Prentice Hall.