Chapter 4 Shear Forces and Bending Moments

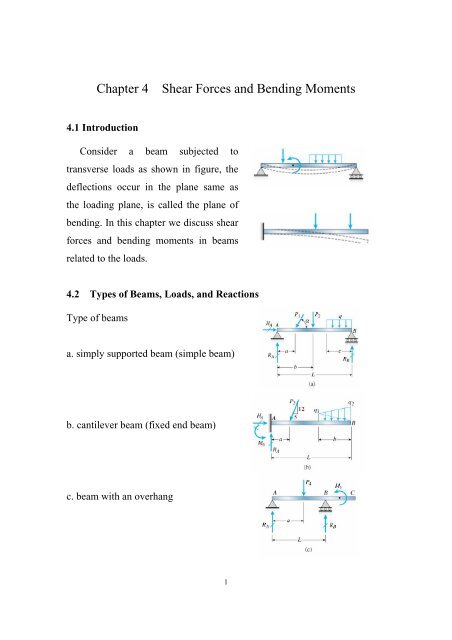
Chapter 4Shear Forces and Bending Moments4.1 IntroductionConsider a beam subjected totransverse loads as shown in figure, thedeflections occur in the plane same asthe loading plane, is called the plane ofbending. In this chapter we discuss shearforces and bending moments in beamsrelated to the loads.4.2 Types of Beams, Loads, and ReactionsType of beamsa. simply supported beam (simple beam)b. cantilever beam (fixed end beam)c. beam with an overhang1

M = 0 M + 2 (q 0 x / L) (x) (x / 3) = 0M = - q 0 x 3 / (6 L)M max = - q 0 L 2 / 6Example 4-3an overhanging beam ABC issupported to an uniform load of intensityq and a concentrated load P, calculatethe shear force V and the bendingmoment M at Dfrom equations of equilibrium, it is foundR A = 40 kNR B = 48 kNat section D F y = 0 40 - 28 - 6 x 5 - V = 0V = - 18 kN M = 0- 40 x 5 + 28 x 2 + 6 x 5 x 2.5 + M = 0M = 69 kN-mfrom the free body diagram of the right-hand part, same results can beobtained4.4 Relationships Between Loads, Shear Forces, and Bending Momentsconsider an element of a beam of lengthdx subjected to distributed loads qequilibrium of forces in vertical direction5

concentrated loadsequilibrium of forceV - P - (V + V 1 ) = 0or V 1 = - Pi.e. an abrupt change in the shear force occursat any point where a concentrated load actsequilibrium of moment- M - P (dx/2) - (V + V 1 ) dx + M + M 1 = 0or M 1 = P (dx/2) + V dx + V 1 dx j 0since the length dx of the element is infinitesimally small, i.e. M 1is also infinitesimally small, thus, the bending moment does not change aswe pass through the point of application of a concentrated loadloads in the form of couplesequilibrium of force V 1 = 0i.e. no change in shear force at the point ofapplication of a coupleequilibrium of moment- M + M 0 - (V + V 1 ) dx + M + M 1 = 0or M 1 = - M 0the bending moment changes abruptly at a point of application of acouple7

4.5 Shear-Force and Bending-Moment Diagramsconcentrated loadsconsider a simply support beam ABwith a concentrated load PR A = P b / L R B = P a / Lfor 0 < x < aV = R A = P b / LM = R A x = P b x / Lnote that dM / dx = P b / L = Vfora < x < LV = R A - P = - P a / LM = R A x - P (x - a) = P a (L - x) / Lnote that dM / dx = - P a / L = VwithM max = P a b / Luniform loadconsider a simple beam AB with auniformly distributed load of constantintensity q8

R A = R B = q L / 2V = R A - q x = q L / 2 - q xM = R A x - q x (x/2) = q L x / 2 - q x 2 / 2note thatdM / dx = q L / 2 - q x / 2 = VM max = q L 2 / 8 at x = L / 2several concentrated loadsfor 0 < x < a 1 V = R A M = R A xM 1 = R A a 1for a 1 < x < a 2 V = R A - P 1M = R A x - P 1 (x - a 1 )M 2 - M 1 = (R A - P 1 )(a 2 - a 1 )similarly for othersM 2 = M max because V = 0 at that pointExample 4-4construct the shear-force and bending-moment diagrams for the simple beamABR A = q b (b + 2c) / 2LR B = q b (b + 2a) / 2Lfor 0 < x < aV = R AM = R A x9

for a < x < a + bV = R A - q (x - a)M = R A x - q (x - a) 2 / 2for a + b < x < LV = - R B M = R B (L - x)maximum moment occurs where V = 0i.e.x 1 = a + b (b + 2c) / 2LM max = q b (b + 2c) (4 a L + 2 b c + b 2 ) / 8L 2for a = c, x 1 = L / 2M max = q b (2L - b) / 8for b = L, a = c = 0(uniform loading over the entire span)M max = q L 2 / 8Example 4-5construct the V- and M-dia for thecantilever beam supported to P 1 and P 2R B = P 1 + P 2M B = P 1 L + P 2 bfor 0 < x < aV = - P 1M = - P 1 xfora < x < LV = - P 1 - P 2 M= - P 1 x - P 2 (x - a)10

Example 4-6construct the V- and M-dia for thecantilever beam supporting to a constant uniformload of intensity qR B = q L M B = q L 2 / 2then V = - q x M = - q x 2 / 2alternative methodV max = - q L M max = - q L 2 / 2xV - V A = V - 0 = V = -∫ q dx = - q x0M - M A = M - 0 = M =x-∫ V dx = - q x 2 / 20Example 4-7an overhanging beam is subjected to auniform load of q = 1 kN/m on ABand a couple M 0 = 12 kN-m on midpointof BC, construct the V- and M-dia forthe beamR B = 5.25 kNR C = 1.25 kNshear force diagramV = - q xV = constanton ABon BC11

ending moment diagramM B = - q b 2 / 2 = - 1 x 4 2 / 2 = - 8 kN-mthe slope of M on BC is constant (1.25 kN), the bending momentjust to the left of M 0 isM = - 8 + 1.25 x 8 = 2 kN-mthe bending moment just to the right of M 0isM = 2 - 12 = - 10 kN-mand the bending moment at point C isM C = - 10 + 1.25 x 8 = 0as expected12