Mathematical modelling and simulation of an ... - IMProVe2011

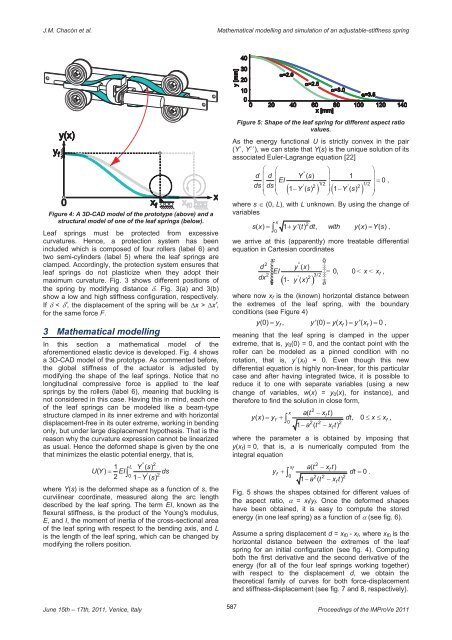
J.M. Chacón et al.Mathematical modelling and simulation of an adjustable-stiffness springFigure 4: A 3D-CAD model of the prototype (above) and astructural model of one of the leaf springs (below).Leaf springs must be protected from excessivecurvatures. Hence, a protection system has beenincluded which is composed of four rollers (label 6) andtwo semi-cylinders (label 5) where the leaf springs areclamped. Accordingly, the protection system ensures thatleaf springs do not plasticize when they adopt theirmaximum curvature. Fig. 3 shows different positions ofthe spring by modifying distance . Fig. 3(a) and 3(b)show a low and high stiffness configuration, respectively.If < ', the displacement of the spring will be x > x',for the same force F.3 Mathematical modellingIn this section a mathematical model of theaforementioned elastic device is developed. Fig. 4 showsa 3D-CAD model of the prototype. As commented before,the global stiffness of the actuator is adjusted bymodifying the shape of the leaf springs. Notice that nolongitudinal compressive force is applied to the leafsprings by the rollers (label 6), meaning that buckling isnot considered in this case. Having this in mind, each oneof the leaf springs can be modeled like a beam-typestructure clamped in its inner extreme and with horizontaldisplacement-free in its outer extreme, working in bendingonly, but under large displacement hypothesis. That is thereason why the curvature expression cannot be linearizedas usual. Hence the deformed shape is given by the onethat minimizes the elastic potential energy, that is,'' 21 L Y ( s)U( Y) EI2 ds0 ' 21 Y ( s)where Y(s) is the deformed shape as a function of s, thecurvilinear coordinate, measured along the arc lengthdescribed by the leaf spring. The term EI, known as theflexural stiffness, is the product of the Young's modulus,E, and I, the moment of inertia of the cross-sectional areaof the leaf spring with respect to the bending axis, and Lis the length of the leaf spring, which can be changed bymodifying the rollers position.Figure 5: Shape of the leaf spring for different aspect ratiovalues.As the energy functional U is strictly convex in the pair(Y´, Y´´), we can state that Y(s) is the unique solution of itsassociated Euler-Lagrange equation [22] ''d d Y ( s) 1 EIdsds ' 2 ' 21 Y ( s) 1 Y ( s)1/2 1/2 0 ,where s (0, L), with L unknown. By using the change ofvariablesx2s( x) 1 y '( t) dt, with y( x) Y( s ) ,0we arrive at this (apparently) more treatable differentialequation in Cartesian coordinatesæ ö2 ''d y ( x)÷EI 0, 0 x x2 3/2fdx' 2÷= < < ,ç ( 1 - y ( x)çè ) ø÷where now x f is the (known) horizontal distance betweenthe extremes of the leaf spring, with the boundaryconditions (see Figure 4)y(0) y , y '(0) y( x ) y '( x ) 0 ,f f fmeaning that the leaf spring is clamped in the upperextreme, that is, y 0 (0) = 0, and the contact point with theroller can be modeled as a pinned condition with norotation, that is, y´(x f ) = 0. Even though this newdifferential equation is highly non-linear, for this particularcase and after having integrated twice, it is possible toreduce it to one with separate variables (using a newchange of variables, w(x) = y 0 (x), for instance), andtherefore to find the solution in close form,2x a( t xft)y( x) yf dt, 0 x x0f ,2 2 21 a ( t x t)where the parameter a is obtained by imposing thaty(x f ) = 0, that is, a is numerically computed from theintegral equationyfx f2a( t x t)0 2 2 21 a ( t xft)ffdt 0 .Fig. 5 shows the shapes obtained for different values ofthe aspect ratio, = x f /y f . Once the deformed shapeshave been obtained, it is easy to compute the storedenergy (in one leaf spring) as a function of (see fig. 6).Assume a spring displacement d = x f0 - x f , where x f0 is thehorizontal distance between the extremes of the leafspring for an initial configuration (see fig. 4). Computingboth the first derivative and the second derivative of theenergy (for all of the four leaf springs working together)with respect to the displacement d, we obtain thetheoretical family of curves for both force-displacementand stiffness-displacement (see fig. 7 and 8, respectively).June 15th – 17th, 2011, Venice, Italy587Proceedings of the IMProVe 2011