Generalizing the Bardos-LeRoux-Nédélec boundary condition for ...

The problem BLN condition & Alternatives Effective BC graph Definition Uniqueness Definition Bis Existence & Convergence of Approximations
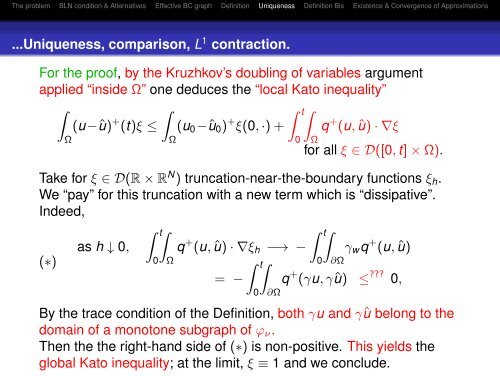
...Uniqueness, comparison, L 1 contraction.
For the proof, by the Kruzhkov’s doubling of variables argument
applied “inside Ω” one deduces the “local Kato inequality”
(u−û) +
(t)ξ ≤ (u0−û0) + t
ξ(0, ·) + q + (u, û) · ∇ξ
Ω
Ω
0
Ω
for all ξ ∈ D([0, t] × Ω).
Take for ξ ∈ D(R × RN ) truncation-near-the-boundary functions ξh.
We “pay” for this truncation with a new term which is “dissipative”.
Indeed,
t
(∗)
as h ↓ 0,
0
q
Ω
+ t
(u, û) · ∇ξh −→ −
0
γwq
∂Ω
+ t
(u, û)
= − q + (γu, γû) ≤ ??? 0,
By the trace condition of the Definition, both γu and γû belong to the
domain of a monotone subgraph of ϕν.
Then the the right-hand side of (∗) is non-positive. This yields the
global Kato inequality; at the limit, ξ ≡ 1 and we conclude.
0
∂Ω