Motion of Projectiles

3.5 Motion in a Plane with Constant Acceleration 65
3.5 MOTION IN A PLANE WITH
CONSTANT ACCELERATION
If an object moves in the xy-plane with constant acceleration, then both a x and a y are
constant. By looking separately at the motion along two perpendicular axes, the y-
direction and the x-direction, each component becomes a one-dimensional problem,
which we already know how to solve. We can apply any of the constant acceleration
relationships from Section 2.5 separately to the x-components and to the y-components.
It is generally easiest to choose the axes so that the acceleration has only one
nonzero component. Suppose we choose the axes so that the acceleration is in the positive
or negative y-direction. Then a x = 0 and v x is constant. With this choice, the constant
acceleration relationships [Eqs. (2-12) through (2-16)] become
x-axis: a x = 0
y-axis: constant a y
∆v x = 0 (v x is constant) ∆v y = a y ∆t (3-10)
∆x = v x ∆t ∆y = 1 2 (v fy + v iy ) ∆t (3-11)
∆y = v iy ∆t + 1 2 a y (∆t) 2 (3-12)
vfy 2 – viy 2 = 2a y ∆y (3-13)
Why are only two equations shown in the column for the x-axis? The other two are
redundant when a x = 0.
Note that there is no mixing of components in Eqs. (3-10) through (3-13). Each
equation pertains either to the x-components or to the y-components; none contains the
x-component of one vector quantity and the y-component of another. The only quantity
that appears in both x- and y-component equations is the time interval—a scalar.
Motion of Projectiles
An object in free fall near the Earth’s surface has a constant acceleration. As long as air
resistance is negligible, the constant downward pull of gravity gives the object a constant
downward acceleration with magnitude g. In Section 2.7 we considered objects in
free fall, but only when they had no horizontal velocity component, so they moved
straight up or straight down. Now we consider objects (called projectiles) in free fall
that have a nonzero horizontal velocity component. The motion of a projectile takes
place in a vertical plane.
Suppose some medieval marauders are attacking a castle. They have a catapult that
propels large stones into the air to bombard the walls of the castle (Fig. 3.16). Picture a
stone leaving the catapult with initial velocity v i . (v i is the initial velocity for the time
interval during which it moves as a projectile. It is also the final velocity for the time
interval during which it is in contact with the catapult.) The angle of elevation is the
angle of the initial velocity above the horizontal. Once the stone is in the air, the only
force acting on it is the downward gravitational force, provided that the air resistance
has a negligible effect on the motion. The trajectory (path) of the stone is shown in
Fig. 3.17. The positive x-axis is chosen in the horizontal direction (to the right) and the
positive y-axis is upward.
If the initial velocity v i is at an angle q above the horizontal, then resolving it into
components gives
v ix = v i cos q and v iy = v i sin q (3-14)
(+y-axis up, q measured from the horizontal x-axis)
Figure 3.16 A medieval
catapult.
θ
v i

66 Chapter 3 Motion in a Plane
y
v y
v y
v y = 0
Figure 3.17 Motion diagram
showing the trajectory of a projectile.
The position is drawn at equal
time intervals. Superimposed are
the velocity vectors along with
their x- and y-components.
v iy
v ix
v
v ix ix
v v y ix
v ix
θ
v y
v ix
v ix
v fy
x
The horizontal and vertical
motions of a projectile can be
treated separately; they are
independent of each other.
With the y-axis pointing up, a y = –g because the acceleration is downward (in the –y
direction). The acceleration has no x-component (a x = 0), so the stone’s horizontal velocity
component v x is constant. The vertical velocity component v y changes at a constant
rate, exactly as if the stone were propelled straight up with an initial speed of v iy . The initially
positive v y decreases until, at the top of flight, v y = 0. Then the pull of gravity makes
the projectile fall back downward. During the downward trip, v y is still changing at the
same constant rate with which it changed on the way up and at the top of the path. The
acceleration has the same constant value—magnitude and direction—for the entire path.
The displacement of the projectile at any instant is the vector sum of the displacements
in the two mutually perpendicular directions. The motion of a projectile when air
resistance is negligible is the superposition of horizontal motion with constant velocity
and vertical motion with constant acceleration. The vertical and horizontal motions
each proceed independently, as if the other motion were not present. In the experiment
of Fig. 3.18, one ball was dropped and, at the same instant, another was projected horizontally.
The strobe photo shows snapshots of the two balls at equally spaced time intervals.
The vertical motion of the two is identical; at every instant, the two are at the same
height. The fact that they have different horizontal motion does not affect their vertical
motion. (This statement would not be true if air resistance were significant.)
Figure 3.18 Independence of
horizontal and vertical motion of
a projectile in the absence of air
resistance. The vertical motion
of the projectile (white) is the
same as that of an object (red)
that falls straight down.
PHYSICS AT HOME
Take a nickel and a penny to a room with a high table or countertop. Place the
penny at the edge of the table and then slide the nickel so it collides with the penny.
Listen for the sound of the two coins hitting the floor. The two coins will slide off
the table with different horizontal velocities but will land at the same time.

3.5 Motion in a Plane with Constant Acceleration 67
Conceptual Example 3.4
Trajectory of a Projectile
The graph of an equation of the form
y = kx 2 , k = a nonzero constant
is a parabola. Show that the trajectory of a projectile is a
parabola. [Hint: Choose the origin at the highest point of
the trajectory and let t i = 0 at that instant.]
Strategy and Solution We start at the high point of
the path and look at displacements from there. The horizontal
displacement is proportional to the elapsed time t
since the horizontal velocity is constant. The vertical displacement
is the average vertical velocity component
times the elapsed time t. The average vertical velocity
component is itself proportional to t since it changes at a
constant rate. Therefore, the vertical displacement is proportional
to t 2 . Thus, the vertical displacement y is proportional
to the square of the horizontal displacement x
and y = kx 2 , where k is a constant of proportionality. The
path followed by a projectile in free fall is a parabola.
Discussion The same conclusion can be drawn algebraically.
With the +y-axis upward and the origin and t = 0
at the top of flight, x i , y i , and v iy are all zero. Then x = v ix t
and
y = v iy t + 1 2 a yt 2 = – 1 2 gt 2 = – 1 2 x g
g v
x 2
2
ix = –
2v
So y is proportional to x 2 and the constant of proportionality
is –g/(2v 2 ix).
Conceptual Practice Problem 3.4
Stones
2
ix
Throwing
You stand at the edge of a cliff and throw stones horizontally
into the river below. To double the horizontal displacement
of a stone from the cliff to where it lands, by
what factor must you increase the stone’s initial speed?
Neglect air resistance.
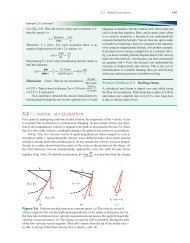
Figure 3.19 shows graphs of the x- and y-components of the velocity and position of
a projectile as functions of time. In this case, the projectile is launched above flat ground
at t = 0 and returns to the same elevation at a later time t f . Note that the y-component
graphs are symmetrical about the vertical line through the highest point in the trajectory.
The y-component of velocity decreases linearly from its initial value; the slope of the line
is a y = –g. When v y = 0, the projectile is at the apex of its trajectory. Then v y continues to
decrease at the same rate and is now negative with its magnitude getting larger and larger.
At t f , when the projectile has returned to its original altitude, the y-component of the
velocity has the same magnitude as at t = 0 but with the opposite sign (v y = –v iy ).
The graph of y(t) indicates that the projectile moves upward, quickly at first and
then gradually slowing, until it reaches the maximum height. The slope of the tangent to
the y(t) graph at any particular moment of time is v y at that instant. At the highest point
of the y(t) graph, the tangent is horizontal and v y = 0. After that, gravity makes the projectile
start to fall downward.
The horizontal velocity is constant, so the graph of v x (t) is a horizontal line. The horizontal
position x increases uniformly in time because the object is moving with a constant v x .
Vertical velocity v y
v iy
0
v fy
0
1
–
2t f
t f
Time
Horizontal velocity v x
v ix
0
0
1
–
2t f
t f
Time
Vertical position y
0
0
1
–
2t f
t f
Time
Horizontal position x
0
0
1
–
2t f
t f
Time
Figure 3.19 Projectile
motion: separate vertical and
horizontal quantities versus time.

68 Chapter 3 Motion in a Plane
Example 3.5
Attacking the Castle Walls
The catapult used by the marauders hurls a stone
with a velocity of 50.0 m/s at a 30.0° angle of elevation
(Fig. 3.20). (a) What is the maximum height
reached by the stone? (b) What is its range (defined as
the horizontal distance traveled when the stone returns to
its original height)? (c) How long has the stone been in
the air when it returns to its original height?
Strategy The problem gives both the magnitude and
direction of the initial velocity of the stone. Ignoring air
resistance, the stone has a constant downward acceleration
once it has been launched—until it hits the ground or
some obstacle. We choose the positive y-axis upward and
the positive x-axis in the direction of horizontal motion of
the stone (toward the castle). When the stone reaches its
maximum height, the velocity component in the y-
direction is zero since the stone goes no higher. When the
stone returns to its original height, ∆y = 0 and v y = –v iy .
The range can be found once the time of flight t f is
known—time is the quantity that connects the x-
component equations to the y-component equations.
Therefore, we solve (c) before (b). One way to find t f is to
find the time to reach maximum height and then double it
(see Fig. 3.19). (Other methods include setting ∆y = 0 or
setting v y = –v iy .)
Solution (a) First we find the x- and y-components of
the initial velocity for an angle of elevation q = 30.0°.
v iy = v i sin q and v ix = v i cos q
The maximum height is the vertical displacement ∆y
when v fy = 0.
∆y = 1 2 (v fy + v iy ) ∆t = 1 2 (0 + v i sin q) ∆t
Eliminating the time interval using v fy – v iy = a y ∆t yields
∆y = 1 2 (v i sin q) 0–v a i sin q
y
= – (v i sin
q) 2
2ay
–(50.0 m/s × sin 30.0°) 2
= = 31.9 m
2 × (–9.80 m/s 2 )
The maximum height of the projectile is 31.9 m above its
launch height.
(c) The time of flight (t f ) is twice the time it takes the projectile
to reach its maximum height. The time to reach the
maximum height can be found from
v fy = 0 = v iy + a y ∆t
Solving for ∆t,
∆t = – viy
a
y
The time of flight is
–50.0 m/s × sin 30.0°
t f = 2 ∆t = 2 × = 5.10 s
–9.80 m/s 2
(b) The range is
∆x = v ix t f = (50.0 m/s × cos 30.0°) × 5.10 s = 221 m
Discussion
Quick check: using
y f – y i = v iy ∆t + 1 2 a y (∆t) 2
we can check that ∆y = 31.9 m when ∆t = 1 2 × 5.10 s and
that ∆y = 0 when ∆t = 5.10 s. Here we check the first of
these:
∆y = (50.0 m/s × sin 30.0°) × 2.55 s + 1 2 × (–9.80 m/s 2 ) × (2.55 s) 2
= 63.8 m + (–31.9 m) = 31.9 m
v ix
v iy
v i
Maximum
height
30.0°
Initial launch height
Range
Figure 3.20
A catapult projects a stone into the air in an attack on a castle wall.
Continued on next page

3.5 Motion in a Plane with Constant Acceleration 69
Example 3.5 Continued
which is correct. This is not an independent check, since
this equation can be derived from the others, but it can
reveal algebra or calculation errors.
Since we analyze the horizontal motion independently
from the vertical motion, we start by resolving the
given initial velocity into x- and y-components. Time is
what connects the horizontal and vertical motions.
Practice Problem 3.5
for Arrows
Maximum Height
Archers have joined in the attack on the castle and are
shooting arrows over the walls. If the angle of elevation
for an arrow is 45°, find an expression for the maximum
height of the arrow in terms of v i and g. [Hint: Simplify
the expression using sin 45° = cos 45° = 1/2.]
PHYSICS AT HOME
On a warm day, take a garden hose and aim the nozzle so that the water streams
upward at an angle above the horizontal. Set the nozzle for a fast, narrow stream for
best effect. Once the water leaves the nozzle, it becomes a projectile subject only to
the force of gravity (neglecting the small effect of air resistance). The continuous
stream of water lets us see the parabolic path easily. Stand in one place and try aiming
the nozzle at different angles of elevation to find an angle that gives the maximum
range. Aim for a particular spot on the ground (at a distance less than the
maximum range) and see if you can find two different angles of elevated nozzle position
that allow the stream to hit the target spot (see Fig. 3.21).
100
75°
60°
y (m)
50
45°
30°
0
0
50
15°
Figure 3.21 Parabolic trajectories of projectiles launched with the same initial speed
(v i = 44.3 m/s) at five different angles. The ranges of projectiles launched at angles q
and 90° – q are the same. The maximum range occurs for q = 45°.
100
x (m)
150
200

70 Chapter 3 Motion in a Plane
Conceptual Example 3.6
Monkey and Hunter
An inexperienced hunter aims and shoots an arrow
straight at a coconut that is being held by a monkey sitting
in a tree (Fig. 3.22). At the same instant that the
arrow leaves the bow, the monkey drops the coconut.
Neglecting air resistance, does the arrow hit the coconut,
the monkey, or neither?
Strategy and Solution If there were no gravity, the
arrow would fly straight to the monkey and coconut
(along the dashed line from the bow to the monkey on
the branch in Fig. 3.22). Since gravity gives the dropped
coconut and the released arrow the same constant acceleration
downward, they each fall the same vertical distance
below the positions they would have had with no
gravity. The coconut falls as shown by the dashed red
line; the distance fallen at 0.25-s intervals is marked
along a vertical axis. At the same time, the arrow drops
below the blue dashed line by the amounts marked along
its indicated trajectory at 0.25-s intervals.
The arrow ends up hitting the coconut no matter what
the initial velocity of the arrow. The higher the velocity of
the arrow, the sooner they meet and the shorter the vertical
distance that the coconut falls before being hit.
Discussion An experienced hunter would have aimed
above the initial position of the coconut to compensate
for the amount his arrow would drop during the time of
flight; he would have missed the dropping coconut but
might have hit the monkey unless the monkey jumped
down to retrieve the coconut.
Conceptual Practice Problem 3.6 Changes in
Position and Velocity for Consecutive Arrows
An arrow is shot into the air. One second later, a second arrow
is shot with the same initial velocity. While the two are both in
the air, does the difference in their positions (r 2 – r 1 ) stay constant
or does it change with time? Does the difference in their
velocities (v 2 – v 1 ) stay constant or does it change with time?
0.3 m
t = 0 s
t = 0.25 s
1.2 m
t = 0.50 s
2.8 m
t = 0.75 s
1.2 m
2.8 m
0.3 m
t = 0.25 s
t = 0.50 s
t = 0.75 s
4.9 m
t = 1.00 s
Figure 3.22
A monkey drops a coconut at the very instant an arrow is shot toward the coconut. In each quarter second, the coconut and arrow
have fallen the same distance below where their positions would be if there were no gravity.

3.6 Velocity Is Relative; Reference Frames 71
Example 3.7
A Bullet Fired Horizontally
A bullet is fired horizontally from the top of a cliff that is
20.0 m above a long lake. If the muzzle speed of the bullet
is 500.0 m/s, how far from the bottom of the cliff does the
bullet strike the surface of the lake? Ignore air resistance.
Strategy We need to find the total time of flight so that
we can find the horizontal displacement. The bullet is
starting from the high point of the parabolic path because
v iy = 0. As usual in projectile problems, we choose the y-
axis to be the positive vertical direction.
Known: ∆y = –20.0 m; v iy = 0; v ix = 500.0 m/s. To find: ∆x.
Solution The vertical displacement through which
the bullet falls is 20.0 m. The relationship between ∆y
and ∆t is
∆y = 1 2 (v fy + v iy ) ∆t
Substituting v iy = 0 and v fy = v iy + a y ∆t = a y ∆t yields
∆y = 1 2 a y(∆t) 2 ⇒∆t = 2 ∆y
a
y
The horizontal displacement of the bullet is
∆x = v ix ∆t = v ix 2 ∆y
a
y
= 500.0 m/s × 2 × (–
2
0 .0 m)
– 9. 80
2 = 1.01 km
m/s
Discussion How did we know to start with the y-
component equation when the question asks about the
horizontal displacement? The question gives v ix and asks
for ∆x. The missing information needed is the time during
which the bullet is in the air; the time can be found
from analysis of the vertical motion.
We neglected air resistance in this problem, which is
not very realistic. The actual distance would be less than
1.01 km.
Practice Problem 3.7
Bullet Velocity
Find the horizontal and vertical components of the bullet’s
velocity just before it hits the surface of the lake. At
what angle does it strike the surface?
At the beginning of the chapter, we asked why the clam does not fall straight down
when the gull lets go. The gull is flying horizontally with the clam, so the clam has the
same horizontal velocity as the gull. When the gull lets go, the net force on the clam is
downward due to gravity. The clam falls toward Earth, but since a x = 0 the clam retains
the same horizontal component of velocity as the gull. Therefore, the clam is a projectile
starting at the top of its parabolic trajectory.
3.6 VELOCITY IS RELATIVE; REFERENCE FRAMES
The idea of relativity arose in physics centuries before Einstein’s theory. Nicole Oresme
(1323–1382) wrote that motion of one object can only be perceived relative to some
other object. Until now, we have tacitly assumed in most situations that displacements,
velocities, and accelerations should be measured in a reference frame attached to
Earth’s surface—that is, by choosing an origin fixed in position relative to Earth’s surface
and a set of axes whose directions are fixed relative to Earth’s surface. After learning
about relative velocities, we will take another look at this assumption.
Relative Velocity
Suppose Wanda is walking down the aisle of a train moving along the track at a constant
velocity (Fig. 3.23). Imagine asking, “How fast is Wanda walking?” This question is not
well defined. Do we mean her speed as measured by Tim, a passenger on the train, or
her speed as measured by Greg, who is standing on the g –
round and looking into the train
as it passes by? The answer to the question “How fast?” depends on the observer.
Figure 3.24 shows Wanda walking from one end of the car to the other during a time
interval ∆t. The displacement of Wanda as measured by Tim—her displacement relative
to the train—is ∆r WT = v WT ∆t. During the same time interval, the train’s displacement
relative to the ground is ∆r TG = v TG ∆t. As measured by Greg, Wanda’s displacement is