Molecular modelling of entangled polymer fluids under flow The ...
Molecular modelling of entangled polymer fluids under flow The ...
Molecular modelling of entangled polymer fluids under flow The ...
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
2.5. BRANCHED POLYMERS 39<br />
By a force balance at the branch points, it can be shown that the maximum tension<br />
which the backbone can sustain is determined by the number <strong>of</strong> arms, q. This results in<br />
the condition that λ cannot exceed q. When λ reaches q the backbone stretch becomes<br />
fixed and the arms segments are drawn into the tube.<br />
<strong>The</strong> length <strong>of</strong> arm material<br />
drawn into the tube is denoted by s c (see figure 2.13). Blackwell et al. (2000) have<br />
modified this onset <strong>of</strong> maximum stretch and their adaption will be discussed below.<br />
McLeish and Larson (1998) provided two non-linear versions <strong>of</strong> their model. In the full<br />
model the orientation equation is the integral equation which was originally derived by<br />
Doi and Edwards (1986) in the context <strong>of</strong> reptation <strong>of</strong> linear <strong>polymer</strong>s. To ease the<br />
computational effort an approximate closed form differential equation for the dynamics<br />
<strong>of</strong> S<br />
≈<br />
was suggested by Harlen. This differential approximation has the same asymptotic<br />
behaviour <strong>under</strong> many simple <strong>flow</strong>s as the integral version. I will use the differential<br />
approximation throughout. This approach disregards the contribution to stress <strong>of</strong> oriented<br />
arm segments hence removing s c as a dynamical variable. <strong>The</strong> evolution <strong>of</strong> S<br />
≈<br />
and λ are governed by differential equations in table 2.2 and the resulting stress tensor,<br />
σ<br />
≈<br />
, is calculated from these quantities. An important feature <strong>of</strong> this model, which<br />
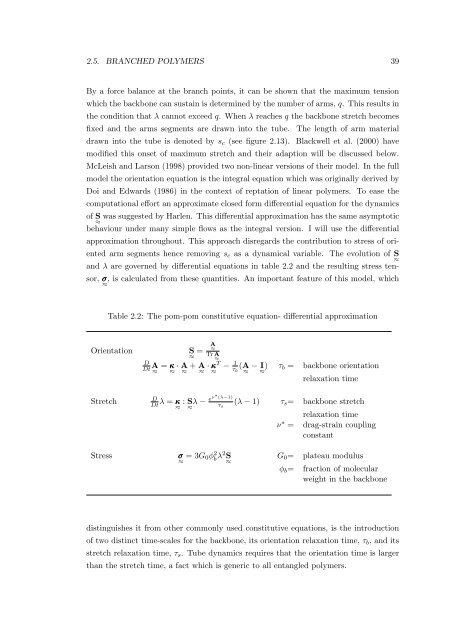
Table 2.2: <strong>The</strong> pom-pom constitutive equation- differential approximation<br />
Orientation S<br />
≈<br />
= A ≈<br />
Tr A ≈<br />
D<br />
Dt A ≈ = κ ≈<br />
· A<br />
≈<br />
+ A<br />
≈<br />
· κ<br />
≈ T − 1 τ b<br />
(A<br />
≈<br />
− I<br />
≈<br />
) τ b = backbone orientation<br />
relaxation time<br />
Stretch<br />
D<br />
Dt λ = κ : Sλ − eν∗ (λ−1)<br />
≈ ≈ τ s<br />
(λ − 1) τ s = backbone stretch<br />
ν ∗ =<br />
relaxation time<br />
drag-strain coupling<br />
constant<br />
Stress σ<br />
≈<br />
= 3G 0 φ 2 b λ2 S<br />
≈<br />
G 0 = plateau modulus<br />
φ b =<br />
fraction <strong>of</strong> molecular<br />
weight in the backbone<br />
distinguishes it from other commonly used constitutive equations, is the introduction<br />
<strong>of</strong> two distinct time-scales for the backbone, its orientation relaxation time, τ b , and its<br />
stretch relaxation time, τ s . Tube dynamics requires that the orientation time is larger<br />
than the stretch time, a fact which is generic to all <strong>entangled</strong> <strong>polymer</strong>s.