Vol. 10 No 5 - Pi Mu Epsilon

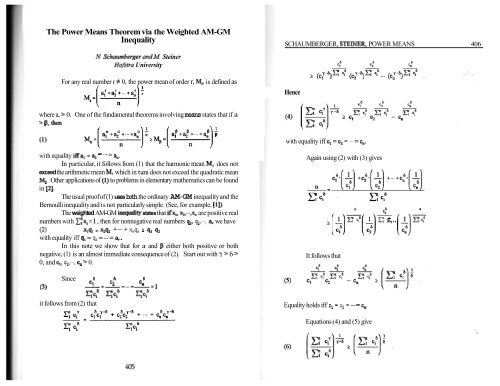
The Power Means Theorem via the Weighted AM-GMInequalityN. Schaumberger andM SteinerHofstra UniversityFor any real number r + 0, the power mean of order r, & is defined asSCHAUMBERGER, STEINER, POWER MEANS 406Hencewhere a, > 0. One of the fundamental theorems involving means states that if a> 0, thenwith equality iff c, = c, = - = cn.with equality iffa, = = - =In particular, it follows from (1) that the harmonic mean M-, does notexceed the arithmetic mean Mi which in turn does not exceed the quadratic meanM2. Other applications of (1) to problems in elementary mathematics can be foundin [2],The usual proof of (1) uses both the ordinary AM-GM inequality and theBernoulli inequality and is not particularly simple. (See, for example, [I]).The weighted AM-GM inequahty states that ifx,, x2,-,x,, are positive realnumbers with Exi = 1, then for nonnegative real numbers q,, q,,-, a, we have(2) x1q1 + X A + + Xnqn 2 q1 q2with equality iff q, = q, = --- = a,.In this note we show that for a and P either both positive or bothnegative, (1) is an almost immediate consequence of (2). Start out with y > 6 :0, and c,, c2,-, cn > 0.Since6c; 2 cn(3)+.+.+GC.6 zc.6it follows from (2) thatwAgain using (2) with (3) givesIt follows thatEquality holds iff cl = c, = - = c,,.[d-(2) [Fb 6 6-c2-1 r^f 1 Fi st,..Equations (4) and (5) give