Manin obstruction to strong approximation for homogeneous spaces

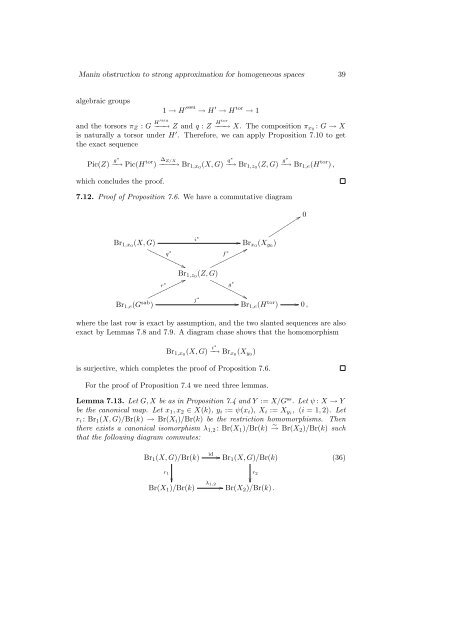
<strong>Manin</strong> <strong>obstruction</strong> <strong>to</strong> strong approximation for homogeneous spaces 39algebraic groups1 → H ′ ssu → H ′ → H <strong>to</strong>r → 1and the <strong>to</strong>rsors π Z : G H′ssu−−→ Z and q : Z H<strong>to</strong>r−−→ X. The composition π x0 : G → Xis naturally a <strong>to</strong>rsor under H ′ . Therefore, we can apply Proposition 7.10 <strong>to</strong> getthe exact sequencePic(Z) −→ g∗Pic(H <strong>to</strong>r ) ∆ Z/X−−−−→ Br 1,x0 (X, G) −→ q∗Br 1,z0 (Z, G) −→ g∗Br 1,e (H <strong>to</strong>r ) ,which concludes the proof.7.12. Proof of Proposition 7.6. We have a commutative diagram iBr 1,x0 (X, G)∗ Br x0 (X y0 )q∗f∗Br 1,z0 (Z, G)r g ∗∗Br 1,e (G sab j ∗) Br 1,e (H <strong>to</strong>r ) 0 ,where the last row is exact by assumption, and the two slanted sequences are alsoexact by Lemmas 7.8 and 7.9. A diagram chase shows that the homomorphismBr 1,x0 (X, G) −→ i∗Br x0 (X y0 )is surjective, which completes the proof of Proposition 7.6.For the proof of Proposition 7.4 we need three lemmas.Lemma 7.13. Let G, X be as in Proposition 7.4 and Y := X/G ss . Let ψ : X → Ybe the canonical map. Let x 1 , x 2 ∈ X(k), y i := ψ(x i ), X i := X yi , (i = 1, 2). Letr i : Br 1 (X, G)/Br(k) → Br(X i )/Br(k) be the restriction homomorphisms. Thenthere exists a canonical isomorphism λ 1,2 : Br(X 1 )/Br(k) ∼ → Br(X 2 )/Br(k) suchthat the following diagram commutes:0Br 1 (X, G)/Br(k)idBr 1 (X, G)/Br(k)(36)r 1Br(X 1 )/Br(k)r 2λ 1,2 Br(X 2 )/Br(k) .