Manin obstruction to strong approximation for homogeneous spaces

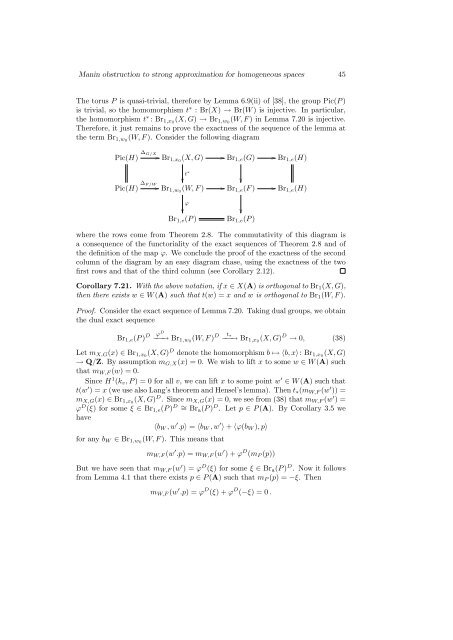
<strong>Manin</strong> <strong>obstruction</strong> <strong>to</strong> strong approximation for homogeneous spaces 45The <strong>to</strong>rus P is quasi-trivial, therefore by Lemma 6.9(ii) of [38], the group Pic(P )is trivial, so the homomorphism t ∗ : Br(X) → Br(W ) is injective. In particular,the homomorphism t ∗ : Br 1,x0 (X, G) → Br 1,w0 (W, F ) in Lemma 7.20 is injective.Therefore, it just remains <strong>to</strong> prove the exactness of the sequence of the lemma atthe term Br 1,w0 (W, F ). Consider the following diagramPic(H) ∆ G/X Br 1,x0 (X, G)Br 1,e (G)Br 1,e (H)t ∗Pic(H) ∆ F/W Br 1,w0 (W, F )Br 1,e (F )Br 1,e (H)ϕBr 1,e (P ) Br 1,e (P )where the rows come from Theorem 2.8. The commutativity of this diagram isa consequence of the func<strong>to</strong>riality of the exact sequences of Theorem 2.8 and ofthe definition of the map ϕ. We conclude the proof of the exactness of the secondcolumn of the diagram by an easy diagram chase, using the exactness of the twofirst rows and that of the third column (see Corollary 2.12).Corollary 7.21. With the above notation, if x ∈ X(A) is orthogonal <strong>to</strong> Br 1 (X, G),then there exists w ∈ W (A) such that t(w) = x and w is orthogonal <strong>to</strong> Br 1 (W, F ).Proof. Consider the exact sequence of Lemma 7.20. Taking dual groups, we obtainthe dual exact sequenceBr 1,e (P ) D−−→ ϕDBr 1,w0 (W, F ) Dt ∗−−→ Br 1,x0 (X, G) D → 0, (38)Let m X,G (x) ∈ Br 1,x0 (X, G) D denote the homomorphism b ↦→ 〈b, x〉: Br 1,x0 (X, G)→ Q/Z. By assumption m G,X (x) = 0. We wish <strong>to</strong> lift x <strong>to</strong> some w ∈ W (A) suchthat m W,F (w) = 0.Since H 1 (k v , P ) = 0 for all v, we can lift x <strong>to</strong> some point w ′ ∈ W (A) such thatt(w ′ ) = x (we use also Lang’s theorem and Hensel’s lemma). Then t ∗ (m W,F (w ′ )) =m X,G (x) ∈ Br 1,x0 (X, G) D . Since m X,G (x) = 0, we see from (38) that m W,F (w ′ ) =ϕ D (ξ) for some ξ ∈ Br 1,e (P ) D ∼ = Br a (P ) D . Let p ∈ P (A). By Corollary 3.5 wehave〈b W , w ′ .p〉 = 〈b W , w ′ 〉 + 〈ϕ(b W ), p〉for any b W ∈ Br 1,w0 (W, F ). This means thatm W,F (w ′ .p) = m W,F (w ′ ) + ϕ D (m P (p))But we have seen that m W,F (w ′ ) = ϕ D (ξ) for some ξ ∈ Br a (P ) D . Now it followsfrom Lemma 4.1 that there exists p ∈ P (A) such that m P (p) = −ξ. Thenm W,F (w ′ .p) = ϕ D (ξ) + ϕ D (−ξ) = 0 .