The spectrum of delay-differential equations: numerical methods - KTH

36 Chapter 2. Computing the spectrum
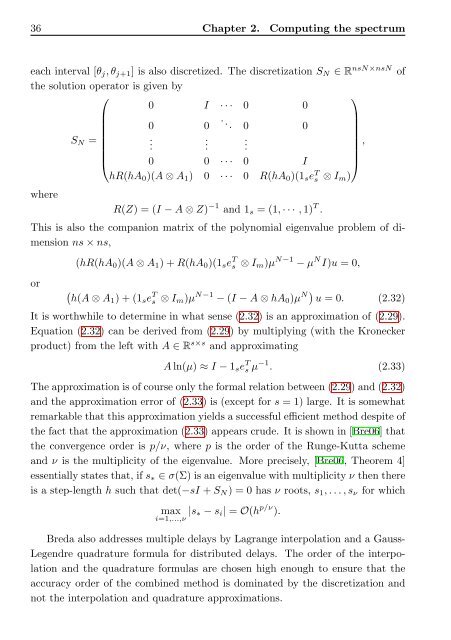
each interval [θj, θj+1] is also discretized. The discretization SN ∈ RnsN×nsN of
the solution operator is given by
⎛
⎞
0 I · · · 0 0
⎜
. ⎟
⎜ 0 0 .. 0 0 ⎟
⎜
SN = ⎜
. . .
⎟ ,
. . .
⎟
⎜
⎟
⎝
⎠
where
0 0 · · · 0 I
hR(hA0)(A ⊗ A1) 0 · · · 0 R(hA0)(1se T s ⊗ Im)
R(Z) = (I − A ⊗ Z) −1 and 1s = (1, · · · , 1) T .
This is also the companion matrix of the polynomial eigenvalue problem of dimension
ns × ns,
or
(hR(hA0)(A ⊗ A1) + R(hA0)(1se T s ⊗ Im)µ N−1 − µ N I)u = 0,
� h(A ⊗ A1) + (1se T s ⊗ Im)µ N−1 − (I − A ⊗ hA0)µ N� u = 0. (2.32)
It is worthwhile to determine in what sense (2.32) is an approximation of (2.29).
Equation (2.32) can be derived from (2.29) by multiplying (with the Kronecker
product) from the left with A ∈ R s×s and approximating
A ln(µ) ≈ I − 1se T s µ −1 . (2.33)
The approximation is of course only the formal relation between (2.29) and (2.32)
and the approximation error of (2.33) is (except for s = 1) large. It is somewhat
remarkable that this approximation yields a successful efficient method despite of
the fact that the approximation (2.33) appears crude. It is shown in [Bre06] that
the convergence order is p/ν, where p is the order of the Runge-Kutta scheme
and ν is the multiplicity of the eigenvalue. More precisely, [Bre06, Theorem 4]
essentially states that, if s∗ ∈ σ(Σ) is an eigenvalue with multiplicity ν then there
is a step-length h such that det(−sI + SN) = 0 has ν roots, s1, . . . , sν for which
max
i=1,...,ν |s∗ − si| = O(h p/ν ).
Breda also addresses multiple delays by Lagrange interpolation and a Gauss-
Legendre quadrature formula for distributed delays. The order of the interpolation
and the quadrature formulas are chosen high enough to ensure that the
accuracy order of the combined method is dominated by the discretization and
not the interpolation and quadrature approximations.