Lecture 5 Bonds to Bands

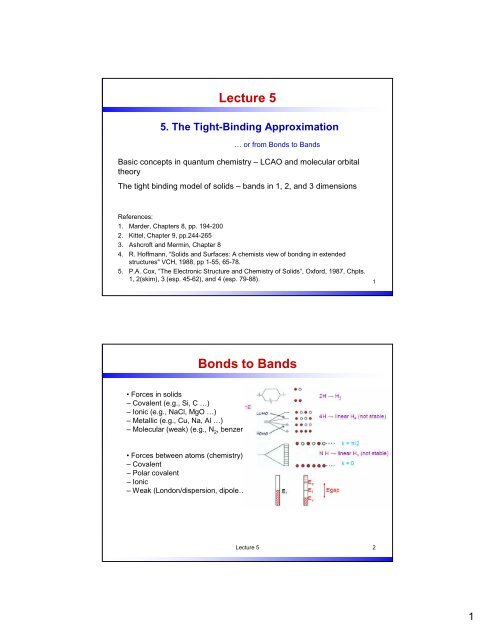
Lecture 55. The Tight-Binding Approximation… or from Bonds to BandsBasic concepts in quantum chemistry – LCAO and molecular orbitaltheoryThe tight binding model of solids – bands in 1, 2, and 3 dimensionsReferences:1. Marder, Chapters 8, pp. 194-2002. Kittel, Chapter 9, pp.244-2653. Ashcroft and Mermin, Chapter 84. R. Hoffmann, “Solids and Surfaces: A chemists view of bonding in extendedstructures" VCH, 1988, pp 1-55, 65-78.5. P.A. Cox, “The Electronic Structure and Chemistry of Solids”, Oxford, 1987, Chpts.1, 2(skim), 3 (esp. 45-62), and 4 (esp. Lecture 79-88). 5 1Bonds to Bands• Forces in solids– Covalent (e.g., Si, C …)– Ionic (e.g., NaCl, MgO …)– Metallic (e.g., Cu, Na, Al …)– Molecular (weak) (e.g., N 2, benzene)• Forces between atoms (chemistry)– Covalent– Polar covalent– Ionic– Weak (London/dispersion, dipole…)Lecture 5 21

But for most problems we use sameapproximation methods:Two main approaches in electronic structure calculations:– Build up from atom: atomic orbitals + …– Infinite solid down: plane waves + …Two methods used in quantum mechanics are variational and perturbationtheory methodsPerturbation theory:H = H o + λ H 1 + λ 2 H 2 + …H o Ψ no= E noΨ no(often known)Lecture 53Variational MethodVariational MethodUse matrix notations Ψ = | Ψ>Η| Ψ> = Ε| Ψ> (time indep. Sch. Eqn.)For stationary stats, if Ψ is normalized, well-behaved function, it can be shownthat< Ψ *|Η | Ψ> = < Ψ *|Ε| Ψ> = Ε ← (variational integral)⇒ Minimize variational integral to get ground state ΨsΨ* | H | ΨE =← (overlap integral)Ψ*|ΨLecture 542

Linear Combination of Atomic Orbitals (LCAO)Best to start to solve Sch. Eq. by choosing good ψ’s!ψ = ∑nAny ψ can be expanded from set of orthonormal functions φ n(satisfyingappropriate boundary conditions)c φn nIn quantum physics/chemistry often use hydrogen-like “atomic orbitals” φ n(s, p, d…) to study molecules (molecular ψ’s)This approach is called LCAO – linear combination of atomic orbitalsLecture 55LCAOGeneral problem is to minimize variational integral by finding coefficients c nthatmake variational integral stationary:∂cn= 0!∂EHΨ = EΨ ⇒H( H − E)∑n∑n∑n∑n∑nc φ = E∑∑c φ = 0c ( Hφ− Eφ) = 0cccn* *n(∫φmHφndV∫[ H − Eδ]nnnnnnmn= 0[ H − ES ] = 0mnnnnnmnmnc φnn* *− φ EφdV ) = 0mnif Φmnorthonormal, if notLecture 563

Bonds to Bands• Determinant becomes:HH( HABAAAA(1 − S− E− ES− E)(H2AB) EAB2BBHABH+ (2H− ESBB− E− E)− ( HABAB− 2HAB= 0AA− ESAB) E + ( H2) = 02AA− H2AB) = 0• Solve quadratic eqs and get 2 solutions:HE =HE =AAAA− HABS(1 − S− HABS(1 − SHAA+ HE1=1+SABABABHAA− HE2=1−SABABABABAB+ HAB− H)(1 + S )− HAB+ H)(1 + S )( + )(-)ABABAAAASSABAB+ sol' n− sol' nLecture 5Energy diagram:ψ1=1( φISA2−φISB)ψ2=1( φISA2+ φISB)βαE 2E 17Molecular system H 2+• Most simple molecular system H 2+(molecular ion with one electron)• For trial functions choose 2 simple 1s atomic orbitalsLecture 584

Definitions• ψ 1is called bonding orbital• ψ 2is called anti-bonding orbital• Remember: each orbital can have 2 electronse.g., HydrogenNitrogenLecture 59NitrogenLecture 5105

Correlation DiagramsHomonuclear diatomicsHeteronuclear diatomicsLecture 511Some symmetry considerations• Strength of bond due to:- overlap of wavefunctions in space- inv. prop. to energy difference of non-interacting orbitals- electron + core repulsionsLecture 5126

Extended Hückel Theory - EHT• Quantum chemical approximation technique (semi-empirical)• Study only valence (i.e., bonding )orbitals• Solve the problem with LCAO variational method, but using ideas fromperturbation theory• The initial state orbitals are atomic Slater type orbitals – STOsXi= Arn−1nexp 0 Y( θ,ϕ)• The initial state energies, H iiare taken from experiments or some othercalculations5r−lmLecture 513Extended Hückel Theory - EHT• Intuitive idea is that the strength of a bond, as represented by the offdiagonalmatrix elements Hij, should be proportional to the extent of overlap(Sij) and the mean energy of the interacting orbitals(Hii+Hjj)/2Hii+ HHij= K2• K is an empirical parameter between 1 and 2• Problems:- H ijis not exact- no charge self consistency- H ijincreases with overlap causing system to show minimum energy whenatoms collapse- no core-core repulsionjjSij• But… EHT does usually show qualitative correct trends in an easilyinterpretable mannerLecture 5147

Orbitals and Bands in 1D1. Equally spaced H atoms2. Isomorphic p-system of a non-bond-alternating delocalized polyene3. Chain of Pt II square-planar complexesLecture 515Lecture 5168

Examples(a) E(k) curve showing the allowed k valuesfor a chain with N = 8 atoms;(b) Orbital energies for eight-atom chain,showing clustering at the top and bottomof the band;(c) Density of states for a chain with verylarge NLecture 5X-ray photoelectron spectrum of thelong chain alkane C 36H 74, showingthe density of states in the 2s band.(J.J.Pireau et.al.,Phys.Rev.A,14 (1976),2133.)19Energy as a function of k for bands of sand ρ−σ orbitals in a linear chainOverlap of ρ−σ orbitals for k = 0and k= ±π/aLecture 52010

Crystal Orbital Overlap Population (COOP)• Better than DOS for determining extent of bonding and anti-bondinginteractionsLecture 521p and d orbitals in linear chainp and d orbitals (in addition to s) – for linear chainLecture 52211

A polymer - linear array of PtH 4 molecules• Monomer-monomer separation ~ 3 Å• The major overlap between d z2and p zorbitalsLecture 523Band structure and DOS for PtH 42-The DOS curves are broadened so that the two-peaked shape of the xy peak inthe DOS is not resolvedLecture 52412

Buckling of chainBuckling of 1D chain to minimize E (periodicity changes - doubles!)Lecture 525Energy minimization for 1D chain - Peierls instabilitySolid-state chemistry analog of Jahn-Teller effectLecture 52613

Peierls Distortion• Qualitative electronic structure and energy minimization of cyclobutadiene⇒ Symmetry breakingLecture 5272D array of s orbitalsLecture 52814

2D array of p orbitalsLecture 529Schematic band structure of a planar square lattice of atoms bearing ns and nporbitalsThe s and p levels have a large separation that the s and p band do not overlapLecture 53015