Hubbard Model for Asymmetric Ultracold Fermionic ... - KOMET 337

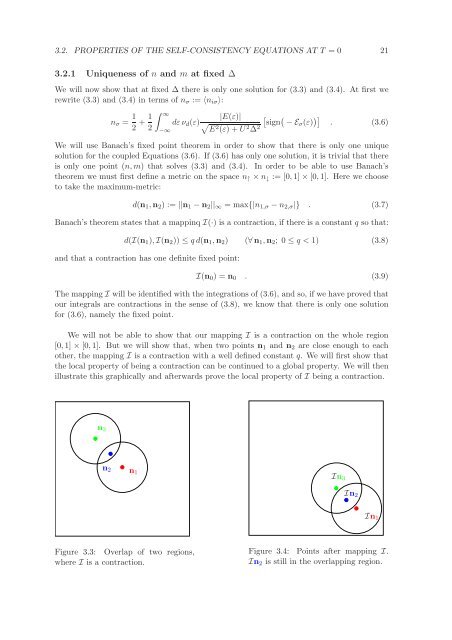
3.2. PROPERTIES OF THE SELF-CONSISTENCY EQUATIONS AT T = 0 213.2.1 Uniqueness of n and m at fixed ∆We will now show that at fixed ∆ there is only one solution for (3.3) and (3.4). At first werewrite (3.3) and (3.4) in terms of n σ := 〈n iσ 〉:n σ = 1 2 + 1 2∫ ∞−∞|E(ε)| [ (dεν d (ε) √ sign − Eσ (ε) )] . (3.6)E 2 (ε) + U 2 ∆ 2We will use Banach’s fixed point theorem in order to show that there is only one uniquesolution for the coupled Equations (3.6). If (3.6) has only one solution, it is trivial that thereis only one point (n,m) that solves (3.3) and (3.4). In order to be able to use Banach’stheorem we must first define a metric on the space n ↑ × n ↓ := [0,1] × [0,1]. Here we chooseto take the maximum-metric:d(n 1 ,n 2 ) := ||n 1 − n 2 || ∞ = max{|n 1,σ − n 2,σ |} . (3.7)Banach’s theorem states that a mappinq I(·) is a contraction, if there is a constant q so that:d(I(n 1 ), I(n 2 )) ≤ q d(n 1 ,n 2 ) (∀n 1 ,n 2 ; 0 ≤ q < 1) (3.8)and that a contraction has one definite fixed point:I(n 0 ) = n 0 . (3.9)The mapping I will be identified with the integrations of (3.6), and so, if we have proved thatour integrals are contractions in the sense of (3.8), we know that there is only one solutionfor (3.6), namely the fixed point.We will not be able to show that our mapping I is a contraction on the whole region[0,1] × [0,1]. But we will show that, when two points n 1 and n 2 are close enough to eachother, the mapping I is a contraction with a well defined constant q. We will first show thatthe local property of being a contraction can be continued to a global property. We will thenillustrate this graphically and afterwards prove the local property of I being a contraction.n 3n 2 n 1In 3In 2In 1Figure 3.3: Overlap of two regions,where I is a contraction.Figure 3.4: Points after mapping I.In 2 is still in the overlapping region.