Chemical Potential, Fugacity, Ideal Solutions, Activity, and Excess ...

Chemical Potential, Fugacity, Ideal Solutions, Activity, and Excess
Gibbs Energy
Prof. Geof Silcox
Chemical Engineering Thermodynamics (CH EN 3853)
University of Utah
2012 June 15
1.0 Mixtures of Ideal Gases, the Gibbs Energy, and the Chemical Potential
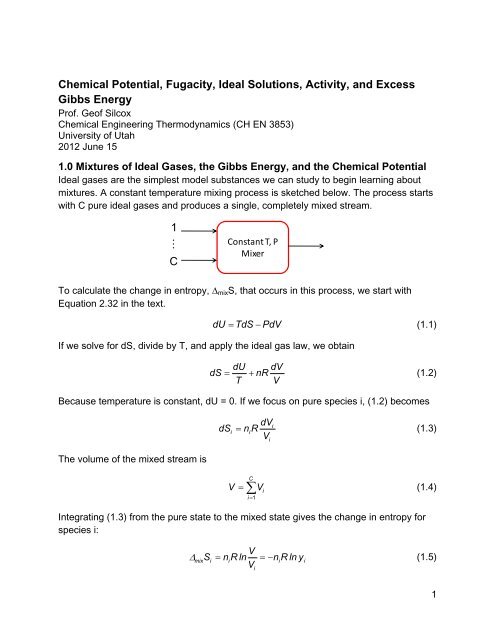
Ideal gases are the simplest model substances we can study to begin learning about
mixtures. A constant temperature mixing process is sketched below. The process starts
with C pure ideal gases and produces a single, completely mixed stream.
To calculate the change in entropy, �mixS, that occurs in this process, we start with
Equation 2.32 in the text.
dU � TdS � PdV
(1.1)
If we solve for dS, divide by T, and apply the ideal gas law, we obtain
dU dV
dS � � nR
(1.2)
T V
Because temperature is constant, dU = 0. If we focus on pure species i, (1.2) becomes
The volume of the mixed stream is
1
�
C
Constant T, P
Mixer
dV
dS n R
V
i � i
i
(1.3)
i
C
V � � Vi
Integrating (1.3) from the pure state to the mixed state gives the change in entropy for
species i:
i �1
mix i i i i
Vi
(1.4)
V
� S � nRln � � nRlny
(1.5)
1

According to (1.5) the entropy change of mixing is always a positive number. The
change in entropy for all species summed together is
or
ig
mixS ig
S
C
ig
ns i i R
C
niln yi
i�1 i�1
� � � � �
The corresponding change in enthalpy is
or
Since G = H – TS, it follows that
� � (1.6)
ig
�
C
i
ig
i �
C
i ln i
i�1 i�1
� � (1.7)
S ns R n y
C
ig ig ig
mixH H nh i i
i �1
� � �� �0
(1.8)
C
ig ig
i i
i �1
H � � nh
(1.9)
ig
�
C
i
ig
i �
C
i ln i
i�1 i�1
� � (1.10)
g y g RT y y
The partial molar equation (6.5, p. 74 of the text), written for the ideal gas Gibbs energy,
is
Comparison of (1.10) and (1.11) gives
ig
�
C
i
ig
i �
C
i
ig
i
i�1 i�1
� � (1.11)
g y g y �
ig ig ig
g � � � g � RTln y
(1.12)
i i i i
Note that (1.12) implies that as the mole fraction, yi, approaches zero,
minus infinity.
Recall that (1.12) is at pressure P. It is convenient to define
different pressure, P 0 . For pure i,
ig
� i relative to
ig
� i approaches
ig
g i at a
dgi � �sidT� vidP (1.13)
2

For a pure ideal gas, and holding T constant, (1.13) is
Integration of (1.14) from P 0 to P gives
ig 0
where i � �
dg �vdP� RTd P
(1.14)
ig ig
iT , i ln
ig ig 0 P
gi �P��gi �P � � RTln (1.15)
0
P
g P is a function of temperature alone. Substituting (1.12) in (1.15) gives
ig 0
Note that i � �
ig ig 0 �yiP� �i
�gi �P , T��RTln� o
P
�
� � (1.16)
g P ,T is independent of composition but is different for every ideal gas. A
convenient choice for P 0 is 1 bar whence (1.16) becomes
�
� y P �
�g �1 bar, T��RTln� 1 bar
�
� � (1.17)
ig ig i
i i
For simplicity, we will not carry the 1 bar that appears in (1.17). For a pure ideal gas,
(1.17) becomes
ig ig
i i
� � ln�
�
� � g T � RT P
(1.18)
2.0 Fugacity
The definition of fugacity is chosen to make (1.17) valid for a real mixture of gases or
liquids:
ig
� �g ( T) � RTlnf (2.1)
i i i
This is (7.1), p. 89 of your text. For a pure substance, (2.1) becomes
ig o
g � g ( T) � RTlnf (2.2)
i i i
The mixture fugacity coefficient, ˆ � i , is a dimensionless property defined by subtracting
(1.17) from (2.1) to give
and
ig fi
ig
� ln ln ˆ
i � �i �RT � �i � RT �i
(2.3)
yP
i
3

For a pure substance, subtracting (1.18) from (2.2) gives
where the pure species fugacity coefficient is
As P approaches zero, (2.4) and (2.6) approach one.
Equation (2.2) can be subtracted from (2.1) to give
ˆ
fi
�i � (2.4)
y P
i
o
ig fi
gi � gi � RTln (2.5)
P
o
fi
�i � (2.6)
P
ln i f
� � g � RT (2.7)
f
i i o
i
where / o
fi fi � ai
is called the activity. The activity is useful in the treatment of chemical
equilibrium.
3.0 Ideal Solutions
Ideal solutions are defined as solutions for which
is
�
C
i
is
i and v �
C
i i
i�1 i�1
� � (3.1)
h x h xv
From the discussion above of mixtures of ideal gases, and by analogy with (1.10),
is
g �
C
xigi �RT
C
xi ln xi
i�1 i�1
� � (3.2)
where gi is the Gibbs energy, kJ/mol, of pure i. For a liquid or solid solution, gi is the
Gibbs energy of the pure liquid or solid. The use of gi for the liquid or solid distinguishes
(3.2) from (1.10).
From (1.11) and (3.2),
4.0 Excess Gibbs Energy
The excess Gibbs energy is defined by
is
� � g � RTln x
(3.3)
i i i
4

The activity coefficient is defined by
Comparison of (4.2) with (1.11) shows that
E � �
�G
� �
� �n
�
E is
g � g � g
(4.1)
C
E
g � RT� x ln�
(4.2)
i TPn , , j�i From (3.2), (4.1), and (4.2) it follows that
i �1
i i
E E
�g � � �RTln�
i i i
is E
g � g �g �
C
xigi �RT C
xi ln xi �RT
C
xi ln�
i
i�1 i�1 i�1
Comparison of (4.4) with (1.11) shows that
Subtracting (2.2) from (2.1) yields
Comparison of (4.5) and (4.6) shows that
(4.3)
� � � (4.4)
� � g � RTln x�
(4.5)
i i i i
ln i f
� � g � RT (4.6)
f
i i o
i
f a
� � � (4.7)
i i
i o
xifixi where ai is the activity and �i is the activity coefficient. For an ideal solution, ai � xi,
f � xf , and � � 1.
o
i i i
i
5