5128_Ch06_pp320-376
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
Section 6.1 Slope Fields and Euler’s Method 325<br />
EXAMPLE 8<br />
Matching Slope Fields with Differential Equations<br />
Use slope analysis to match each of the following differential equations with one of the<br />
slope fields (a) through (d). (Do not use your graphing calculator.)<br />
1. d y<br />
x y 2. d y<br />
xy 3. d y<br />
x dx<br />
dx<br />
dx<br />
y 4. d y<br />
y dx<br />
x <br />
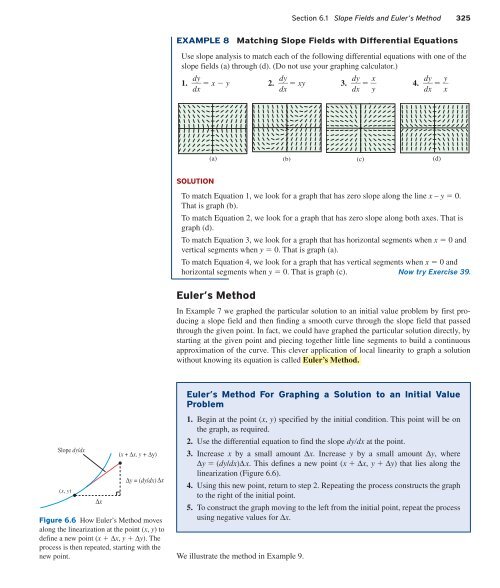
(a) (b) (c) (d)<br />
SOLUTION<br />
To match Equation 1, we look for a graph that has zero slope along the line x – y 0.<br />
That is graph (b).<br />
To match Equation 2, we look for a graph that has zero slope along both axes. That is<br />
graph (d).<br />
To match Equation 3, we look for a graph that has horizontal segments when x 0 and<br />
vertical segments when y 0. That is graph (a).<br />
To match Equation 4, we look for a graph that has vertical segments when x 0 and<br />
horizontal segments when y 0. That is graph (c). Now try Exercise 39.<br />
Euler’s Method<br />
In Example 7 we graphed the particular solution to an initial value problem by first producing<br />
a slope field and then finding a smooth curve through the slope field that passed<br />
through the given point. In fact, we could have graphed the particular solution directly, by<br />
starting at the given point and piecing together little line segments to build a continuous<br />
approximation of the curve. This clever application of local linearity to graph a solution<br />
without knowing its equation is called Euler’s Method.<br />
Slope dy/dx<br />
(x, y)<br />
Δx<br />
(x + Δx, y + Δy)<br />
Δy = (dy/dx) Δx<br />
Figure 6.6 How Euler’s Method moves<br />
along the linearization at the point (x, y) to<br />
define a new point (x Δx, y Δy). The<br />
process is then repeated, starting with the<br />
new point.<br />
Euler’s Method For Graphing a Solution to an Initial Value<br />
Problem<br />
1. Begin at the point (x, y) specified by the initial condition. This point will be on<br />
the graph, as required.<br />
2. Use the differential equation to find the slope dydx at the point.<br />
3. Increase x by a small amount Δx. Increase y by a small amount Δy, where<br />
Δy (dy/dx)Δx. This defines a new point (x Δx, y Δy) that lies along the<br />
linearization (Figure 6.6).<br />
4. Using this new point, return to step 2. Repeating the process constructs the graph<br />
to the right of the initial point.<br />
5. To construct the graph moving to the left from the initial point, repeat the process<br />
using negative values for Δx.<br />
We illustrate the method in Example 9.