Sullivan Microsite TE SAMPLE
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
<strong>Sullivan</strong> AP˙<strong>Sullivan</strong>˙Chapter01 October 8, 2016 17:4<br />
Section 1.3 • Continuity 105<br />
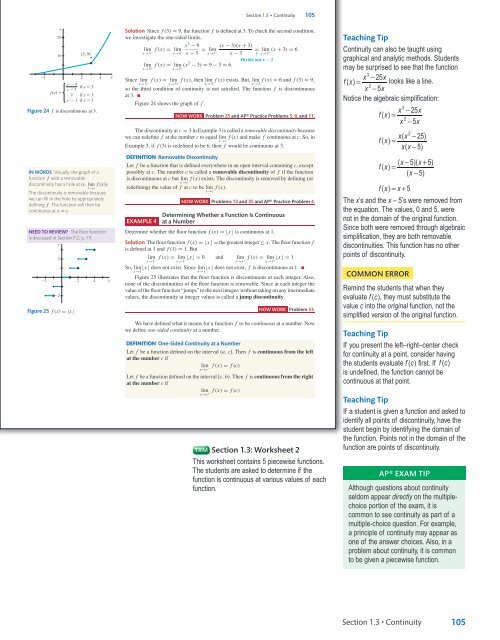
2<br />
y<br />
20<br />
10<br />
(3, 9)<br />
2 4<br />
x 2 9<br />
if x 3<br />
x 3<br />
f (x) <br />
9 if x 3<br />
x 2 3 if x 3<br />
Figure 24 f is discontinuous at 3.<br />
IN WORDS Visually, the graph of a<br />
function f with a removable<br />
discontinuity has a hole at (c, lim x→c<br />
f (x)).<br />
The discontinuity is removable because<br />
we can fill in the hole by appropriately<br />
defining f . The function will then be<br />
continuous at x = c.<br />
NEED TO REVIEW? The floor function<br />
is discussed in Section P.2, p. 19.<br />
y<br />
2<br />
2<br />
2<br />
Figure 25 f (x) =x<br />
2<br />
4<br />
x<br />
x<br />
Solution Since f (3) = 9, the function f is defined at 3. To check the second condition,<br />
we investigate the one-sided limits.<br />
Teaching Tip<br />
x 2 − 9<br />
lim f (x) = lim<br />
x→3 −<br />
x→3 − x − 3 = lim (x − 3)(x + 3)<br />
= lim<br />
x→3 − x − 3 x→3−(x + 3) = 6<br />
↑<br />
Divide out x − 3<br />
lim f (x) = lim<br />
x→3 +<br />
x→3 +(x 2 − 3) = 9 − 3 = 6<br />
3<br />
Since lim f (x) = lim f (x), then lim f (x) exists. But, lim f (x) = 6 and f (3) = 9,<br />
x −25x<br />
x→3 −<br />
x→3 +<br />
x→3 x→3 fx ( ) =<br />
2<br />
so the third condition of continuity is not satisfied. The function f is discontinuous<br />
x −5x<br />
at 3. ■<br />
Figure 24 shows the graph of f .<br />
NOW WORK Problem 25 and AP® Practice Problems 5, 8, and 11.<br />
fx<br />
The discontinuity at c = 3 in Example 3 is called a removable discontinuity because<br />
we can redefine f at the number c to equal lim f (x) and make f continuous at c. So, in<br />
x→c fx<br />
Example 3, if f (3) is redefined to be 6, then f would be continuous at 3.<br />
DEFINITION Removable Discontinuity<br />
Let f be a function that is defined everywhere in an open interval containing c, except<br />
fx<br />
possibly at c. The number c is called a removable discontinuity of f if the function<br />
is discontinuous at c but lim f (x) exists. The discontinuity is removed by defining (or<br />
x→c<br />
redefining) the value of f at c to be lim f (x).<br />
x→c fx<br />
NOW WORK Problems 13 and 35 and AP® Practice Problem 4.<br />
Determining Whether a Function Is Continuous<br />
EXAMPLE 4 at a Number<br />
Determine whether the floor function f (x) =x is continuous at 1.<br />
Solution The floor function f (x) =x =the greatest integer ≤ x. The floor function f<br />
is defined at 1 and f (1) = 1. But<br />
lim f (x) = lim<br />
x→1 −<br />
x→1−x =0 and lim f (x) = lim<br />
x→1 +<br />
x→1 +x<br />
=1<br />
So, limx does not exist. Since limx does not exist, f is discontinuous at 1. ■<br />
x→1 x→1<br />
Figure 25 illustrates that the floor function is discontinuous at each integer. Also,<br />
none of the discontinuities of the floor function is removable. Since at each integer the<br />
value of the floor function “jumps” to the next integer, without taking on any intermediate<br />
values, the discontinuity at integer values is called a jump discontinuity.<br />
evaluate fc<br />
NOW WORK Problem 53.<br />
We have defined what it means for a function f to be continuous at a number. Now<br />
we define one-sided continuity at a number.<br />
Teaching Tip<br />
DEFINITION One-Sided Continuity at a Number<br />
Let f be a function defined on the interval (a, c]. Then f is continuous from the left<br />
at the number c if<br />
lim f (x) = f (c)<br />
x→c −<br />
Let f be a function defined on the interval [c, b). Then f is continuous from the right<br />
at the number c if<br />
lim f (x) = f (c)<br />
x→c +<br />
TRM Section 1.3: Worksheet 2<br />
This worksheet contains 5 piecewise functions.<br />
The students are asked to determine if the<br />
function is continuous at various values of each<br />
function.<br />
Continuity can also be taught using<br />
graphical and analytic methods. Students<br />
may be surprised to see that the function<br />
looks like a line.<br />
Notice the algebraic simplification:<br />
3<br />
x −25x<br />
( ) =<br />
2<br />
x −5x<br />
2<br />
xx ( −25)<br />
( ) =<br />
xx ( −5)<br />
( x− 5)( x+<br />
5)<br />
( ) =<br />
( x −5)<br />
( ) = x + 5<br />
The x’s and the x – 5’s were removed from<br />
the equation. The values, 0 and 5, were<br />
not in the domain of the original function.<br />
Since both were removed through algebraic<br />
simplification, they are both removable<br />
discontinuities. This function has no other<br />
points of discontinuity.<br />
Common Error<br />
Remind the students that when they<br />
(), they must substitute the<br />
value c into the original function, not the<br />
simplified version of the original function.<br />
If you present the left–right–center check<br />
for continuity at a point, consider having<br />
the students evaluate f() c first. If f()<br />
c<br />
is undefined, the function cannot be<br />
continuous at that point.<br />
Teaching Tip<br />
If a student is given a function and asked to<br />
identify all points of discontinuity, have the<br />
student begin by identifying the domain of<br />
the function. Points not in the domain of the<br />
function are points of discontinuity.<br />
AP® Exam Tip<br />
Although questions about continuity<br />
seldom appear directly on the multiplechoice<br />
portion of the exam, it is<br />
common to see continuity as part of a<br />
multiple-choice question. For example,<br />
a principle of continuity may appear as<br />
one of the answer choices. Also, in a<br />
problem about continuity, it is common<br />
to be given a piecewise function.<br />
Section 1.3 • Continuity 105<br />
<strong>TE</strong>_<strong>Sullivan</strong>_Chapter01_PART I.indd 2<br />
11/01/17 9:57 am