Sullivan Microsite TE SAMPLE

Sullivan AP˙Sullivan˙Chapter01 October 8, 2016 17:4
Sullivan
106 Chapter 1 • Limits and Continuity
AP® CaLC skill builder
for example 5
Determining Whether a Function Is
Continuous on a Closed Interval
⎧
⎪
fx ( ) = ⎨
⎪
⎩
2
x −3x−4
x − 4
k
x ≠ 4
x = 4
Determine the value of k that makes the
function f defined above continuous at
x = 4.
Solution
The function will be continuous at x = 4 if
lim fx ( ) = lim fx ( ) = f (4).
− +
x→4 x→4
In Example 4, we showed that the floor function f (x) =x is discontinuous at
x = 1. But since
f (1) =1 =1 and lim f (x) =x =1
x→1 +
the floor function is continuous from the right at 1. In fact, the floor function is
discontinuous at each integer n, but it is continuous from the right at every integer n.
(Do you see why?)
2 Determine Intervals on Which a Function Is Continuous
So far, we have considered only continuity at a number c. Now, we use one-sided
continuity to define continuity on an interval.
DEFINITION Continuity on an Interval
• A function f is continuous on an open interval (a, b) if f is continuous at every
number in (a, b).
• A function f is continuous on an interval [a, b) if f is continuous on the open
interval (a, b) and continuous from the right at the number a.
• A function f is continuous on an interval (a, b] if f is continuous on the open
interval (a, b) and continuous from the left at the number b.
• A function f is continuous on a closed interval [a, b] if f is continuous on the
open interval (a, b), continuous from the right at a, and continuous from the left
at b.
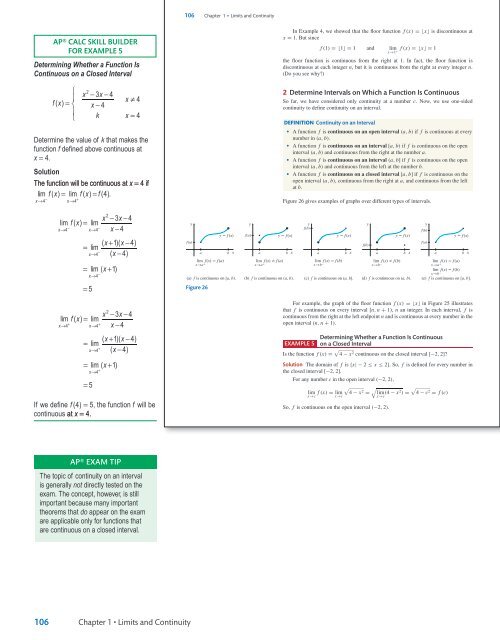
Figure 26 gives examples of graphs over different types of intervals.
x
lim fx ( ) = lim
−
−
x→4 x→4
−3x−4
x −4
( x+ 1)( x−4)
= lim
−
x→4
( x −4)
= lim ( x + 1)
−
x→4
2
y
f(a)
y f (x)
a
b x
lim f(x) f (a)
x→a
(a) f is continuous on [a, b).
y
f(a)
y f (x)
a
b x
lim f(x) f (a)
x→a
(b) f is continuous on (a, b).
y
f(b)
y f (x)
a
b x
lim f(x) f (b)
x→b
(c) f is continuous on (a, b].
y
y f (x)
f(b)
a
b x
lim f(x) f (b)
x→b
(d) f is continuous on (a, b).
y
f(b)
y f (x)
f(a)
a
b x
lim f (x) f (a)
x→a
lim f (x) f (b)
x→b
(e) f is continuous on [a, b].
= 5
Figure 26
x
lim fx ( ) = lim
+ +
x→4 x→4
−3x−4
x −4
( x+ 1)( x−4)
= lim
+
x→4
( x −4)
= lim ( x + 1)
+
x→4
= 5
If we define f (4) = 5, the function f will be
continuous at x = 4.
2
For example, the graph of the floor function f (x) =x in Figure 25 illustrates
that f is continuous on every interval [n, n + 1), n an integer. In each interval, f is
continuous from the right at the left endpoint n and is continuous at every number in the
open interval (n, n + 1).
EXAMPLE 5
Determining Whether a Function Is Continuous
on a Closed Interval
Is the function f (x) = √ 4 − x 2 continuous on the closed interval [−2, 2]?
Solution The domain of f is {x|−2 ≤ x ≤ 2}. So, f is defined for every number in
the closed interval [−2, 2].
For any number c in the open interval (−2, 2),
lim
x→c
f (x) = lim
x→c
So, f is continuous on the open interval (−2, 2).
√
4 − x
2
= √ lim
x→c
(4 − x 2 ) = √ 4 − c 2 = f (c)
AP® Exam Tip
The topic of continuity on an interval
is generally not directly tested on the
exam. The concept, however, is still
important because many important
theorems that do appear on the exam
are applicable only for functions that
are continuous on a closed interval.
106
Chapter 1 • Limits and Continuity
TE_Sullivan_Chapter01_PART I.indd 3
11/01/17 9:58 am