Surface algorithms using bounds on derivatives

298 D. Filip et aL / <strong>Surface</strong> <strong>algorithms</strong>
(a)
B
R 2
A C A ~11
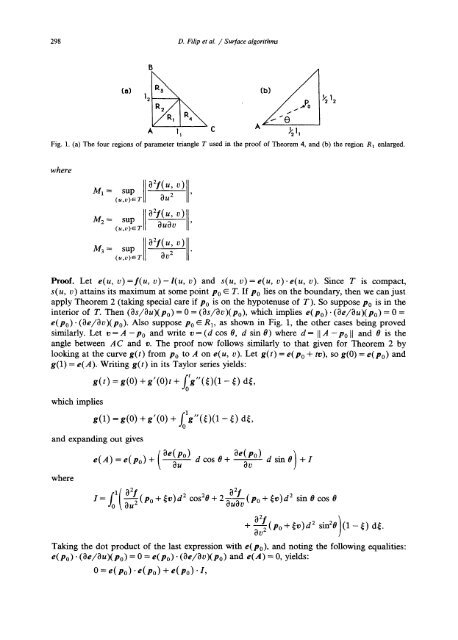
Fig. 1. (a) The four regions of parameter triangle T used in the proof of Theorem 4, and (b) the region R 1 enlarged.
where
= 0 2i( u, o)l
M1 (u,v)ETSUp an 2 ] ,
sup I°V(u'°),
(u,o)~T OUOO
343= sup
L
O2f(u' v). I
(u,v)~T 0V2 l"
Proof. Let e(u, v)=f(u, v)-l(u, v) and s(u, v)=e(u, v).e(u, v). Since T is compact,
s(u, v) attains its maximum at some point P0 ~ T. If P0 lies on the boundary, then we can just
apply Theorem 2 (taking special care if P0 is on the hypotenuse of T). So suppose P0 is in the
interior of T. Then (3s/Ou)(po) = 0 = (3s/Ov)(po), which implies e(po) . (Oe/Ou)(Po) = 0 =
e(po). (3e/Ov)(po). Also suppose Po ~ R1, as shown in Fig. 1, the other cases being proved
similarly. Let v=A-Po and write v=(d cos 0, d sin 0) where d= IIA -P0 II and 0 is the
angle between A C and v. The proof now follows similarly to that given for Theorem 2 by
looking at the curve g(t) from Po to A on e(u, v). Let g(t) = e(Po + tv), so g(0) = e(Po) and
g(1) = e(A). Writing g(t) in its Taylor series yields:
which implies
,(t) =,(0) + g'(0)t +
g(a) = g(0) + g'(0) +
and expanding out gives
where
e(A) = e(Po) + ( Oe(po)
Ou
1( 02! (
I = fo --'--~ Ou
fotg"(f)(1 ~) d~,
fo'g"(f)(1 f) d~,
d cos 0 + )(Oe'P°-----------" d sin OI ~ + I
0v /
02I
Po + ~v) d2 c°s20 + 2 3--~v (Po + t;v) d2 sin 0 cos 0
+ Ov 202Izkpo + ~v)d 2 sin20)(1 -- ~) d~.
Taking the dot product of the last expression with e(po), and noting the following equalities:
e(Po) . (8e/au)(po) = 0 = e(Po) . (3e/3v)(po) and e( A) = 0, yields:
O=e(Po) .e(po) + e(Po). I,