CHAPTER 3 - Educators

94 CHAPTER 3/COST-ESTIMATION TECHNIQUES
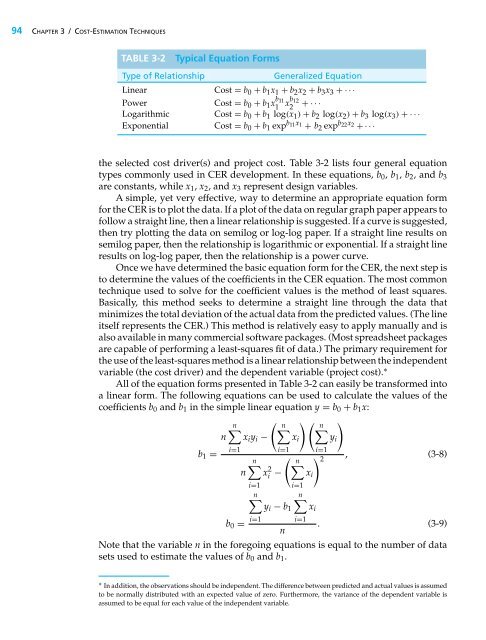
TABLE 3-2 Typical Equation Forms
Type of Relationship Generalized Equation
Linear Cost = b0 + b1x1 + b2x2 + b3x3 +···
Power Cost = b0 + b1x b11
1 xb12
2 +···
Logarithmic Cost = b0 + b1 log(x1) + b2 log(x2) + b3 log(x3) +···
Exponential Cost = b0 + b1 expb11x1 + b2 expb22x2 +···
the selected cost driver(s) and project cost. Table 3-2 lists four general equation
types commonly used in CER development. In these equations, b0, b1, b2, and b3
are constants, while x1, x2, and x3 represent design variables.
A simple, yet very effective, way to determine an appropriate equation form
for the CER is to plot the data. If a plot of the data on regular graph paper appears to
follow a straight line, then a linear relationship is suggested. If a curve is suggested,
then try plotting the data on semilog or log-log paper. If a straight line results on
semilog paper, then the relationship is logarithmic or exponential. If a straight line
results on log-log paper, then the relationship is a power curve.
Once we have determined the basic equation form for the CER, the next step is
to determine the values of the coefficients in the CER equation. The most common
technique used to solve for the coefficient values is the method of least squares.
Basically, this method seeks to determine a straight line through the data that
minimizes the total deviation of the actual data from the predicted values. (The line
itself represents the CER.) This method is relatively easy to apply manually and is
also available in many commercial software packages. (Most spreadsheet packages
are capable of performing a least-squares fit of data.) The primary requirement for
the use of the least-squares method is a linear relationship between the independent
variable (the cost driver) and the dependent variable (project cost). ∗
All of the equation forms presented in Table 3-2 can easily be transformed into
a linear form. The following equations can be used to calculate the values of the
coefficients b0 and b1 in the simple linear equation y = b0 + b1x:
n
n
n
n xiyi −
b1 =
i=1
n
i=1
xi
n
x 2 i −
n
i=1
n
i=1
n
xi
yi − b1 xi
i=1 i=1
i=1
2 yi
, (3-8)
b0 =
. (3-9)
n
Note that the variable n in the foregoing equations is equal to the number of data
sets used to estimate the values of b0 and b1.
∗ In addition, the observations should be independent. The difference between predicted and actual values is assumed
to be normally distributed with an expected value of zero. Furthermore, the variance of the dependent variable is
assumed to be equal for each value of the independent variable.