Métodos miméticos de pasos fraccionarios - E-Archivo - Universidad ...

§2.3 spatial semidiscretization: a cell-node mfd method 17
( i− 1
2
( i− 1
2
• •
, j+ 1
2
•
, j− 1
2
) ( i+ 1
2
⊗
) (i+ 1
2
, j+ 1
2
•
, j− 1
2
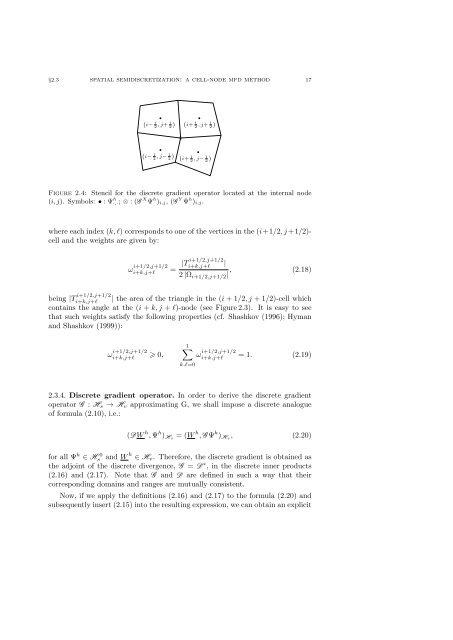
Figure 2.4: Stencil for the discrete gradient operator located at the internal node
(i, j). Symbols: • : Ψ h ·,·; ⊗ : (G X Ψ h )i,j, (G Y Ψ h )i,j.
where each index (k, ℓ) corresponds to one of the vertices in the (i+1/2, j +1/2)cell
and the weights are given by:
ω i+1/2,j+1/2
i+k,j+ℓ
= |T i+1/2,j+1/2
)
)
i+k,j+ℓ |
, (2.18)
2 |Ωi+1/2,j+1/2| being |T i+1/2,j+1/2
i+k,j+ℓ | the area of the triangle in the (i + 1/2, j + 1/2)-cell which
contains the angle at the (i + k, j + ℓ)-node (see Figure 2.3). It is easy to see
that such weights satisfy the following properties (cf. Shashkov (1996); Hyman
and Shashkov (1999)):
ω i+1/2,j+1/2
i+k,j+ℓ
0,
1∑
k,ℓ=0
ω i+1/2,j+1/2
i+k,j+ℓ = 1. (2.19)
2.3.4. Discrete gradient operator. In order to derive the discrete gradient
operator G : Hs → Hv approximating G, we shall impose a discrete analogue
of formula (2.10), i.e.:
(DW h , Ψ h )Hs = (W h , G Ψ h )Hv, (2.20)
for all Ψ h ∈ H 0
s and W h ∈ Hv. Therefore, the discrete gradient is obtained as
the adjoint of the discrete divergence, G = D ∗ , in the discrete inner products
(2.16) and (2.17). Note that G and D are defined in such a way that their
corresponding domains and ranges are mutually consistent.
Now, if we apply the definitions (2.16) and (2.17) to the formula (2.20) and
subsequently insert (2.15) into the resulting expression, we can obtain an explicit