C H A P T E R 2 Polynomial and Rational Functions
C H A P T E R 2 Polynomial and Rational Functions
C H A P T E R 2 Polynomial and Rational Functions
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
204 Chapter 2 <strong>Polynomial</strong> <strong>and</strong> <strong>Rational</strong> <strong>Functions</strong><br />
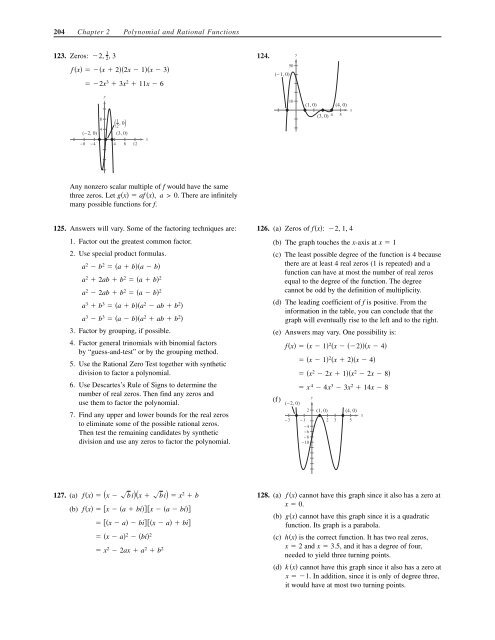
123. Zeros:<br />
f x x 22x 1x 3<br />
8<br />
4<br />
(−2, 0)<br />
−8<br />
2, 1<br />
2 , 3<br />
2x 3 3x 2 11x 6<br />
−4<br />
y<br />
((<br />
1<br />
, 0<br />
2<br />
(3, 0)<br />
4 8 12<br />
x<br />
Any nonzero scalar multiple of f would have the same<br />
three zeros. Let gx af x, a > 0. There are infinitely<br />
many possible functions for f.<br />
125. Answers will vary. Some of the factoring techniques are:<br />
1. Factor out the greatest common factor.<br />
2. Use special product formulas.<br />
127. (a)<br />
a 2 b 2 a ba b<br />
a 2 2ab b 2 a b 2<br />
a 2 2ab b 2 a b 2<br />
a 3 b 3 a ba 2 ab b 2 <br />
a 3 b 3 a ba 2 ab b 2 <br />
3. Factor by grouping, if possible.<br />
4. Factor general trinomials with binomial factors<br />
by “guess-<strong>and</strong>-test” or by the grouping method.<br />
5. Use the <strong>Rational</strong> Zero Test together with synthetic<br />
division to factor a polynomial.<br />
6. Use Descartes’s Rule of Signs to determine the<br />
number of real zeros. Then find any zeros <strong>and</strong><br />
use them to factor the polynomial.<br />
7. Find any upper <strong>and</strong> lower bounds for the real zeros<br />
to eliminate some of the possible rational zeros.<br />
Then test the remaining c<strong>and</strong>idates by synthetic<br />
division <strong>and</strong> use any zeros to factor the polynomial.<br />
(b)<br />
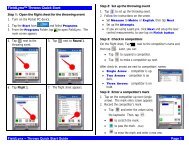
124.<br />
(−1, 0)<br />
50<br />
10<br />
y<br />
(1, 0) (4, 0)<br />
x<br />
(3, 0) 4 5<br />
126. (a) Zeros of fx: 2, 1, 4<br />
(b) The graph touches the x-axis at x 1<br />
(c) The least possible degree of the function is 4 because<br />
there are at least 4 real zeros (1 is repeated) <strong>and</strong> a<br />
function can have at most the number of real zeros<br />
equal to the degree of the function. The degree<br />
cannot be odd by the definition of multiplicity.<br />
(d) The leading coefficient of f is positive. From the<br />
information in the table, you can conclude that the<br />
graph will eventually rise to the left <strong>and</strong> to the right.<br />
(e) Answers may vary. One possibility is:<br />
(f)<br />
fx x 1 2 x 2x 4<br />
x 1 2 x 2x 4<br />
x 2 2x 1x 2 2x 8<br />
x 4 4x 3 3x 2 14x 8<br />
y<br />
(−2, 0)<br />
2 (1, 0) (4, 0)<br />
−3 −1<br />
−4<br />
−6<br />
−8<br />
−10<br />
2 3 5<br />
x2 2ax a2 b2 x a2 bi2 fx x bix bi x<br />
fx x a bix a bi<br />
x a bix a bi<br />
2 b 128. (a) f x<br />
cannot have this graph since it also has a zero at<br />
x 0.<br />
(b) gx cannot have this graph since it is a quadratic<br />
function. Its graph is a parabola.<br />
(c) hx is the correct function. It has two real zeros,<br />
x 2 <strong>and</strong> x 3.5, <strong>and</strong> it has a degree of four,<br />
needed to yield three turning points.<br />
(d) k x cannot have this graph since it also has a zero at<br />
x 1. In addition, since it is only of degree three,<br />
it would have at most two turning points.<br />
x