Analytical modeling for heat transfer in sheared flows of nanofluids
Analytical modeling for heat transfer in sheared flows of nanofluids
Analytical modeling for heat transfer in sheared flows of nanofluids
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
CLAUDIO FERRARI et al. PHYSICAL REVIEW E 86, 016302 (2012)<br />
important at distances 2 smaller than the particle diameter 2R<br />
<strong>for</strong> both k = 5 and 100. Thus any nonl<strong>in</strong>ear dependence <strong>of</strong><br />
Nu on ϕ can appear only if the particles are close enough<br />
to overlap the deviation from the l<strong>in</strong>ear temperature pr<strong>of</strong>ile.<br />
We see from our numerics that this <strong>in</strong>teraction causes a 10%<br />
deviation from the straight l<strong>in</strong>e <strong>in</strong> Nu versus the ϕ dependence<br />
<strong>for</strong> volume fractions <strong>of</strong> about 0.1 and more <strong>for</strong> k 100. For<br />
ϕ>0.1, there is some nonl<strong>in</strong>ear dependence <strong>of</strong> Nu on ϕ<br />
but it practically co<strong>in</strong>cides <strong>for</strong> the random and the periodic<br />
distributions <strong>of</strong> spherical particles. Notice that the situation<br />
is different <strong>for</strong> elongated particles: the randomness <strong>of</strong> the<br />
particle orientations is not captured by a periodic simulation.<br />
Such simulations describe only very dilute suspension, without<br />
nonl<strong>in</strong>ear effects <strong>in</strong> ϕ.<br />
III. TOWARD AN ANALYTICAL MODEL OF THE HEAT<br />
FLUX<br />
In this section, we beg<strong>in</strong> by collect<strong>in</strong>g the required <strong>in</strong><strong>for</strong>mation<br />
about the dependence <strong>of</strong> Kj on the various parameters<br />
(2). To do this we consider simple limit<strong>in</strong>g cases, <strong>for</strong> which<br />
some <strong>of</strong> these parameters are set to zero. We start with the case<br />
<strong>of</strong> spherical particles.<br />
A. Spherical nanoparticles<br />
1. Test case: spherical nanoparticles <strong>in</strong> a fluid at rest<br />
Here we consider the simplest case <strong>of</strong> a spherical particle<br />
(r = 1) <strong>in</strong> a fluid at rest (Re = Pe = 0). Chiew and Glandt [18]<br />
suggested the follow<strong>in</strong>g <strong>for</strong>mula <strong>for</strong> this case:<br />
3 K1(k)<br />
K(k,ϕ) <br />
3 − K1(k)ϕ , K1(k)<br />
k − 1<br />
= 3 , (7a)<br />
k + 2<br />
Nu = 1 + 3 K1(k) ϕ<br />
. (7b)<br />
3 − K1(k) ϕ<br />
We notice that the expression <strong>for</strong> K1(k) which controls<br />
the very dilute limit (ϕ → 0) is the same as <strong>in</strong> the Maxwell<br />
model [19]. Obviously, <strong>for</strong> k = 1 one has zero effect, i.e.,<br />
Nu = 1. For k →∞, K1 = 3 and one has maximal possible<br />
enhancement (at fixed ϕ ≪ 1). For k = 2, one has 25% <strong>of</strong> this<br />
value, k = 4 gives already 50% <strong>of</strong> the effect, k = 10 ⇒ 75%,<br />
k = 20 ⇒ 86%, and k = 100 ⇒ 97%. Clearly, the larger k the<br />
better, but <strong>in</strong>creas<strong>in</strong>g k above 100 is not effective.<br />
Actually, Eq. (7) <strong>in</strong>cludes also <strong>in</strong><strong>for</strong>mation about the<br />
nonl<strong>in</strong>ear dependence K(ϕ). However, this dependence is<br />
very weak <strong>in</strong> the relevant range <strong>of</strong> parameters: e.g., <strong>for</strong><br />
k = 10 and ϕ = 0.2 the difference between K(ϕ) and its l<strong>in</strong>ear<br />
approximation is only 15%. For smaller k, this difference is<br />
even smaller. The physical reason <strong>for</strong> that was expla<strong>in</strong>ed at the<br />
end <strong>of</strong> the preced<strong>in</strong>g section.<br />
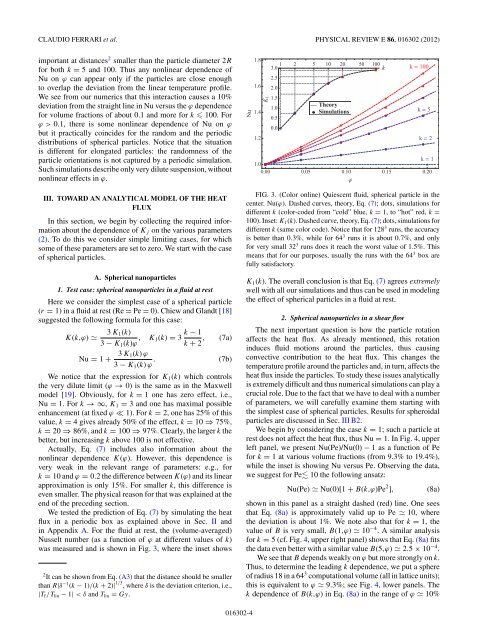
We tested the prediction <strong>of</strong> Eq. (7) by simulat<strong>in</strong>g the <strong>heat</strong><br />
flux <strong>in</strong> a periodic box as expla<strong>in</strong>ed above <strong>in</strong> Sec. II and<br />
<strong>in</strong> Appendix A. For the fluid at rest, the (volume-averaged)<br />
Nusselt number (as a function <strong>of</strong> ϕ at different values <strong>of</strong> k)<br />
was measured and is shown <strong>in</strong> Fig. 3, where the <strong>in</strong>set shows<br />
2 It can be shown from Eq. (A3) that the distance should be smaller<br />
than R|δ −1 (k − 1)/(k + 2)| 1/3 ,whereδ is the deviation criterion, i.e.,<br />
|Tf/Tl<strong>in</strong> − 1|