A NULLSTELLENSATZ FOR AMOEBAS

410 KEVIN PURBHOO
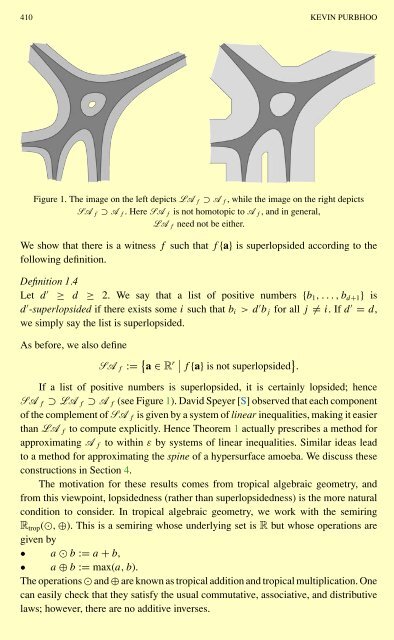
Figure 1. The image on the left depicts LA f ⊃ A f , while the image on the right depicts
SA f ⊃ A f . Here SA f is not homotopic to A f , and in general,
LA f need not be either.
We show that there is a witness f such that f {a} is superlopsided according to the
following definition.
Definition 1.4
Let d ′ ≥ d ≥ 2. We say that a list of positive numbers {b 1 ,...,b d+1 } is
d ′ -superlopsided if there exists some i such that b i >d ′ b j for all j ≠ i. Ifd ′ = d,
we simply say the list is superlopsided.
As before, we also define
SA f := { a ∈ R r ∣ ∣ f {a} is not superlopsided } .
If a list of positive numbers is superlopsided, it is certainly lopsided; hence
SA f ⊃ LA f ⊃ A f (see Figure 1). David Speyer [S] observed that each component
of the complement of SA f is given by a system of linear inequalities, making it easier
than LA f to compute explicitly. Hence Theorem 1 actually prescribes a method for
approximating A f to within ε by systems of linear inequalities. Similar ideas lead
to a method for approximating the spine of a hypersurface amoeba. We discuss these
constructions in Section 4.
The motivation for these results comes from tropical algebraic geometry, and
from this viewpoint, lopsidedness (rather than superlopsidedness) is the more natural
condition to consider. In tropical algebraic geometry, we work with the semiring
R trop (⊙, ⊕). This is a semiring whose underlying set is R but whose operations are
given by
• a ⊙ b := a + b,
• a ⊕ b := max(a,b).
The operations ⊙ and ⊕ are known as tropical addition and tropical multiplication. One
can easily check that they satisfy the usual commutative, associative, and distributive
laws; however, there are no additive inverses.