A NULLSTELLENSATZ FOR AMOEBAS
A NULLSTELLENSATZ FOR AMOEBAS
A NULLSTELLENSATZ FOR AMOEBAS
You also want an ePaper? Increase the reach of your titles
YUMPU automatically turns print PDFs into web optimized ePapers that Google loves.
ENDOSCOPIC LIFTING 459<br />
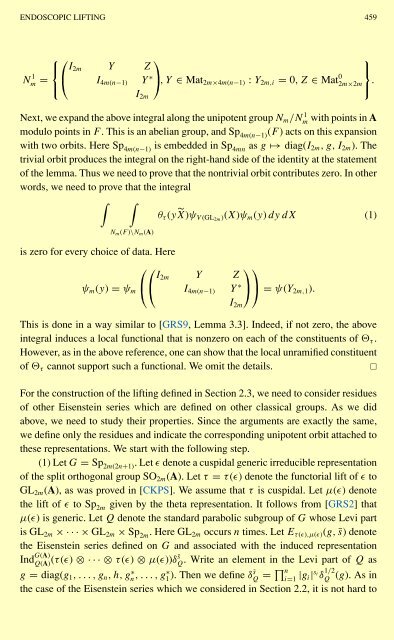
⎧⎛<br />
⎞<br />
⎫<br />
⎨ I 2m Y Z<br />
⎬<br />
N 1 m = ⎝ I<br />
⎩<br />
4m(n−1) Y ∗ ⎠,Y ∈ Mat 2m×4m(n−1) : Y 2m,i = 0,Z ∈ Mat 0 2m×2m⎭ .<br />
I 2m<br />
Next, we expand the above integral along the unipotent group N m /Nm 1 with points in A<br />
modulo points in F . This is an abelian group, and Sp 4m(n−1) (F ) acts on this expansion<br />
with two orbits. Here Sp 4m(n−1) is embedded in Sp 4mn as g ↦→ diag(I 2m ,g,I 2m ).The<br />
trivial orbit produces the integral on the right-hand side of the identity at the statement<br />
of the lemma. Thus we need to prove that the nontrivial orbit contributes zero. In other<br />
words, we need to prove that the integral<br />
∫ ∫<br />
θ τ (y˜X)ψ V (GL2m )(X)ψ m (y) dy dX (1)<br />
N m (F )\N m (A)<br />
is zero for every choice of data. Here<br />
⎛⎛<br />
⎞⎞<br />
I 2m Y Z<br />
ψ m (y) = ψ m<br />
⎝⎝<br />
I 4m(n−1) Y ∗ ⎠⎠ = ψ(Y 2m,1 ).<br />
I 2m<br />
This is done in a way similar to [GRS9, Lemma 3.3]. Indeed, if not zero, the above<br />
integral induces a local functional that is nonzero on each of the constituents of τ .<br />
However, as in the above reference, one can show that the local unramified constituent<br />
of τ cannot support such a functional. We omit the details.<br />
<br />
For the construction of the lifting defined in Section 2.3, we need to consider residues<br />
of other Eisenstein series which are defined on other classical groups. As we did<br />
above, we need to study their properties. Since the arguments are exactly the same,<br />
we define only the residues and indicate the corresponding unipotent orbit attached to<br />
these representations. We start with the following step.<br />
(1) Let G = Sp 2m(2n+1) .Letɛ denote a cuspidal generic irreducible representation<br />
of the split orthogonal group SO 2m (A). Letτ = τ(ɛ) denote the functorial lift of ɛ to<br />
GL 2m (A), aswasprovedin[CKPS]. We assume that τ is cuspidal. Let µ(ɛ) denote<br />
the lift of ɛ to Sp 2m given by the theta representation. It follows from [GRS2] that<br />
µ(ɛ) is generic. Let Q denote the standard parabolic subgroup of G whose Levi part<br />
is GL 2m ×···×GL 2m × Sp 2m . Here GL 2m occurs n times. Let E τ(ɛ),µ(ɛ) (g, ¯s) denote<br />
the Eisenstein series defined on G and associated with the induced representation<br />
Ind G(A)<br />
Q(A) (τ(ɛ) ⊗ ··· ⊗ τ(ɛ) ⊗ µ(ɛ))δ¯s Q . Write an element in the Levi part of Q as<br />
g = diag(g 1 ,...,g n ,h,gn ∗,...,g∗ 1 ). Then we define δ¯s Q = ∏ n<br />
i=1 |g i| s i δ1/2 Q (g). Asin<br />
the case of the Eisenstein series which we considered in Section 2.2, it is not hard to