A NULLSTELLENSATZ FOR AMOEBAS

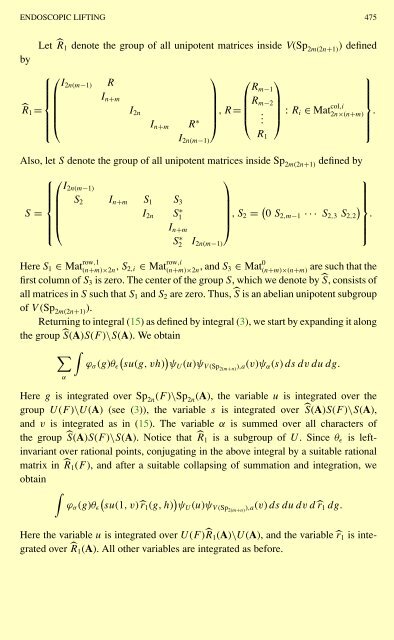
ENDOSCOPIC LIFTING 475
by
Let ̂R 1 denote the group of all unipotent matrices inside V(Sp 2m(2n+1) ) defined
⎧⎛
⎪⎨
̂R 1 =
⎜
⎝
⎪⎩
I 2n(m−1)
⎞
R
⎛
I n+m I 2n ⎟
I n+m R ∗ ⎠ ,R= ⎜
⎝
I 2n(m−1)
R m−1
R m−2
.
R 1
⎞
⎟
⎠ : R i ∈ Mat col,i
2n×(n+m)
Also, let S denote the group of all unipotent matrices inside Sp 2m(2n+1) defined by
⎧⎛
⎪⎨
S =
⎜
⎝
⎪⎩
I 2n(m−1)
S 2 I n+m S 1 S 3
I 2n S1
∗
I n+m
S2
∗
⎫
⎪⎬
.
⎞
⎫
⎟
⎠ ,S 2 = ( )
⎪⎬
0 S 2,m−1 ··· S 2,3 S 2,2 .
⎪⎭
I 2n(m−1)
Here S 1 ∈ Mat row,1
(n+m)×2n ,S 2,i ∈ Mat row,i
(n+m)×2n ,andS 3 ∈ Mat 0 (n+m)×(n+m)
are such that the
first column of S 3 is zero. The center of the group S, which we denote by Ŝ, consists of
all matrices in S such that S 1 and S 2 are zero. Thus, Ŝ is an abelian unipotent subgroup
of V (Sp 2m(2n+1) ).
Returning to integral (15) as defined by integral (3), we start by expanding it along
the group Ŝ(A)S(F )\S(A). We obtain
∑
∫
( )
ϕ σ (g)θ ɛ su(g, vh) ψU (u)ψ V (Sp2(m+n) ),a(v)ψ α (s) ds dv dudg.
α
Here g is integrated over Sp 2n (F )\Sp 2n (A), the variable u is integrated over the
group U(F )\U(A) (see (3)), the variable s is integrated over Ŝ(A)S(F )\S(A),
and v is integrated as in (15). The variable α is summed over all characters of
the group Ŝ(A)S(F )\S(A). Notice that ̂R 1 is a subgroup of U. Sinceθ ɛ is leftinvariant
over rational points, conjugating in the above integral by a suitable rational
matrix in ̂R 1 (F ), and after a suitable collapsing of summation and integration, we
obtain
∫
(
ϕ σ (g)θ ɛ su(1,v)̂r1 (g, h) ) ψ U (u)ψ V (Sp2(m+n) ),a(v) ds dudv d̂r 1 dg.
⎪⎭
Here the variable u is integrated over U(F )̂R 1 (A)\U(A), and the variable ̂r 1 is integrated
over ̂R 1 (A). All other variables are integrated as before.