A NULLSTELLENSATZ FOR AMOEBAS

480 DAVID GINZBURG
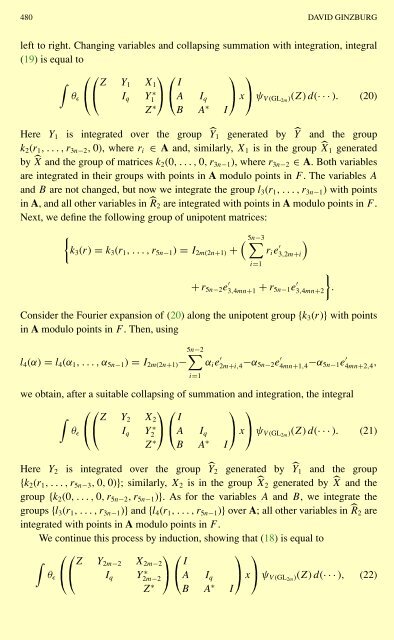
left to right. Changing variables and collapsing summation with integration, integral
(19) is equal to
⎛⎛
⎞ ⎛ ⎞ ⎞
∫ Z Y 1 X 1 I
θ ɛ
⎝⎝
I q Y1
∗ ⎠ ⎝A
I q
⎠ x⎠ ψ V (GL2m )(Z) d(···). (20)
Z ∗ B A ∗ I
Here Y 1 is integrated over the group Ŷ 1 generated by Ŷ and the group
k 2 (r 1 ,...,r 3n−2 , 0), where r i ∈ A and, similarly, X 1 is in the group ̂X 1 generated
by ̂X and the group of matrices k 2 (0,...,0,r 3n−1 ), where r 3n−2 ∈ A. Both variables
are integrated in their groups with points in A modulo points in F . The variables A
and B are not changed, but now we integrate the group l 3 (r 1 ,...,r 3n−1 ) with points
in A, and all other variables in ̂R 2 are integrated with points in A modulo points in F .
Next, we define the following group of unipotent matrices:
{
( 5n−3
∑ )
k 3 (r) = k 3 (r 1 ,...,r 5n−1 ) = I 2m(2n+1) + r i e ′ 3,2m+i
i=1
+ r 5n−2 e ′ 3,4mn+1 + r 5n−1e ′ 3,4mn+2
Consider the Fourier expansion of (20) along the unipotent group {k 3 (r)} with points
in A modulo points in F . Then, using
5n−2
∑
l 4 (α) = l 4 (α 1 ,...,α 5n−1 ) = I 2m(2n+1) − α i e ′ 2m+i,4 −α 5n−2e ′ 4mn+1,4 −α 5n−1e ′ 4mn+2,4 ,
i=1
we obtain, after a suitable collapsing of summation and integration, the integral
⎛⎛
⎞ ⎛ ⎞ ⎞
∫ Z Y 2 X 2 I
θ ɛ
⎝⎝
I q Y2
∗ ⎠ ⎝A
I q
⎠ x⎠ ψ V (GL2m )(Z) d(···). (21)
Z ∗ B A ∗ I
Here Y 2 is integrated over the group Ŷ 2 generated by Ŷ 1 and the group
{k 2 (r 1 ,...,r 5n−3 , 0, 0)}; similarly, X 2 is in the group ̂X 2 generated by ̂X and the
group {k 2 (0,...,0,r 5n−2 ,r 5n−1 )}. As for the variables A and B, we integrate the
groups {l 3 (r 1 ,...,r 3n−1 )} and {l 4 (r 1 ,...,r 5n−1 )} over A; all other variables in ̂R 2 are
integrated with points in A modulo points in F .
We continue this process by induction, showing that (18) is equal to
⎛⎛
⎞ ⎛ ⎞ ⎞
∫ Z Y 2m−2 X 2m−2 I
θ ɛ
⎝⎝
I q Y2m−2
∗ ⎠ ⎝A
I q
⎠ x⎠ ψ V (GL2m )(Z) d(···), (22)
Z ∗ B A ∗ I
}
.