Barycentric Coordinates for Arbitrary Polygons in the Plane
Barycentric Coordinates for Arbitrary Polygons in the Plane
Barycentric Coordinates for Arbitrary Polygons in the Plane
Create successful ePaper yourself
Turn your PDF publications into a flip-book with our unique Google optimized e-Paper software.
20 K. Hormann<br />
v j+2<br />
v<br />
Ψ^<br />
r j+1<br />
α j+1<br />
^<br />
α α +<br />
r^<br />
j ^<br />
α j–1<br />
α –<br />
r j<br />
v j–1<br />
v^<br />
v j<br />
v j+1<br />
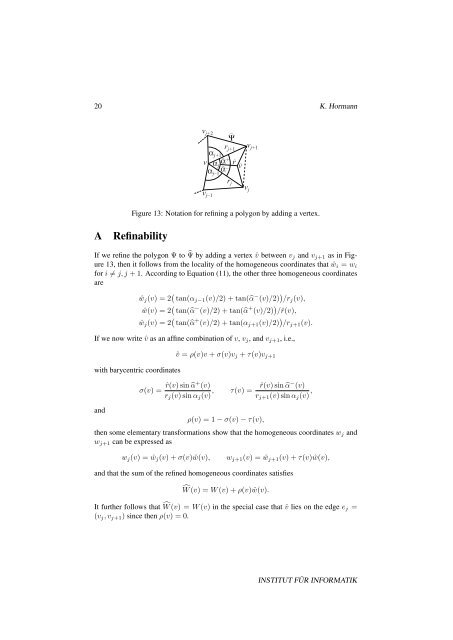
Figure 13: Notation <strong>for</strong> ref<strong>in</strong><strong>in</strong>g a polygon by add<strong>in</strong>g a vertex.<br />
A<br />
Ref<strong>in</strong>ability<br />
If we ref<strong>in</strong>e <strong>the</strong> polygon Ψ to ̂Ψ by add<strong>in</strong>g a vertex ˆv between v j and v j+1 as <strong>in</strong> Figure<br />
13, <strong>the</strong>n it follows from <strong>the</strong> locality of <strong>the</strong> homogeneous coord<strong>in</strong>ates that ŵ i = w i<br />
<strong>for</strong> i ≠ j, j + 1. Accord<strong>in</strong>g to Equation (11), <strong>the</strong> o<strong>the</strong>r three homogeneous coord<strong>in</strong>ates<br />
are<br />
ŵ j (v) = 2 ( tan(α j−1 (v)/2) + tan(̂α − (v)/2) ) /r j (v),<br />
ŵ(v) = 2 ( tan(̂α − (v)/2) + tan(̂α + (v)/2) ) /ˆr(v),<br />
ŵ j (v) = 2 ( tan(̂α + (v)/2) + tan(α j+1 (v)/2) ) /r j+1 (v).<br />
If we now write ˆv as an aff<strong>in</strong>e comb<strong>in</strong>ation of v, v j , and v j+1 , i.e.,<br />
with barycentric coord<strong>in</strong>ates<br />
and<br />
ˆv = ρ(v)v + σ(v)v j + τ(v)v j+1<br />
σ(v) = ˆr(v) s<strong>in</strong> ̂α+ (v)<br />
r j (v) s<strong>in</strong> α j (v) , τ(v) = ˆr(v) s<strong>in</strong> ̂α− (v)<br />
r j+1 (v) s<strong>in</strong> α j (v) ,<br />
ρ(v) = 1 − σ(v) − τ(v),<br />
<strong>the</strong>n some elementary trans<strong>for</strong>mations show that <strong>the</strong> homogeneous coord<strong>in</strong>ates w j and<br />
w j+1 can be expressed as<br />
w j (v) = ŵ j (v) + σ(v)ŵ(v),<br />
w j+1 (v) = ŵ j+1 (v) + τ(v)ŵ(v),<br />
and that <strong>the</strong> sum of <strong>the</strong> ref<strong>in</strong>ed homogeneous coord<strong>in</strong>ates satisfies<br />
Ŵ (v) = W (v) + ρ(v)ŵ(v).<br />
It fur<strong>the</strong>r follows that Ŵ (v) = W (v) <strong>in</strong> <strong>the</strong> special case that ˆv lies on <strong>the</strong> edge e j =<br />
(v j , v j+1 ) s<strong>in</strong>ce <strong>the</strong>n ρ(v) = 0.<br />
INSTITUT FÜR INFORMATIK