Barycentric Coordinates for Arbitrary Polygons in the Plane

BARYCENTRIC COORDINATES FOR ARBITRARY POLYGONS 5
r i+1
v i–1
v
v i+1
α
α i
r i–1 i–1
A i Ψ
v i
A i–1 r i
v
B i
Ψ
C i
v i–1
v i
v i+1
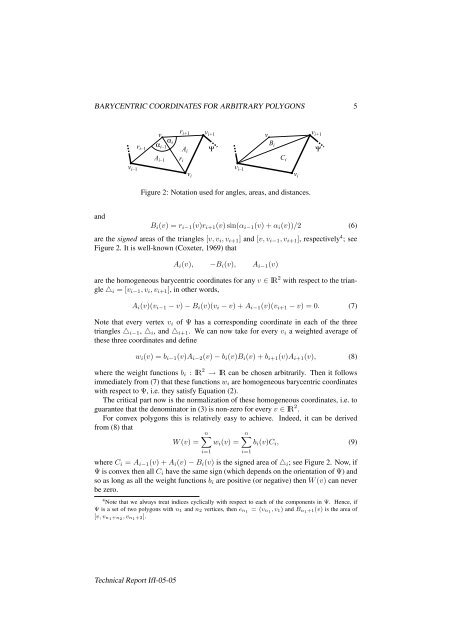
Figure 2: Notation used for angles, areas, and distances.
and
B i (v) = r i−1 (v)r i+1 (v) sin(α i−1 (v) + α i (v))/2 (6)
are the signed areas of the triangles [v, v i , v i+1 ] and [v, v i−1 , v i+1 ], respectively 4 ; see
Figure 2. It is well-known (Coxeter, 1969) that
A i (v), −B i (v), A i−1 (v)
are the homogeneous barycentric coordinates for any v ∈ IR 2 with respect to the triangle
△ i = [v i−1 , v i , v i+1 ], in other words,
A i (v)(v i−1 − v) − B i (v)(v i − v) + A i−1 (v)(v i+1 − v) = 0. (7)
Note that every vertex v i of Ψ has a corresponding coordinate in each of the three
triangles △ i−1 , △ i , and △ i+1 . We can now take for every v i a weighted average of
these three coordinates and define
w i (v) = b i−1 (v)A i−2 (v) − b i (v)B i (v) + b i+1 (v)A i+1 (v), (8)
where the weight functions b i : IR 2 → IR can be chosen arbitrarily. Then it follows
immediately from (7) that these functions w i are homogeneous barycentric coordinates
with respect to Ψ, i.e. they satisfy Equation (2).
The critical part now is the normalization of these homogeneous coordinates, i.e. to
guarantee that the denominator in (3) is non-zero for every v ∈ IR 2 .
For convex polygons this is relatively easy to achieve. Indeed, it can be derived
from (8) that
n∑
n∑
W (v) = w i (v) = b i (v)C i , (9)
i=1
where C i = A i−1 (v) + A i (v) − B i (v) is the signed area of △ i ; see Figure 2. Now, if
Ψ is convex then all C i have the same sign (which depends on the orientation of Ψ) and
so as long as all the weight functions b i are positive (or negative) then W (v) can never
be zero.
4 Note that we always treat indices cyclically with respect to each of the components in Ψ. Hence, if
Ψ is a set of two polygons with n 1 and n 2 vertices, then e n1 = (v n1 , v 1 ) and B n1 +1(v) is the area of
[v, v n1 +n 2
, v n1 +2].
i=1
Technical Report IfI-05-05