Breaking the Stick

G13 BREAKING A STICK #1 

G 1 3 

Capsule Lesson Summary 

Given two line segments, construct as many essentially different triangles as possible 

with each side the same length as one of the line segments. Discover a rule relating the 

length of the segments to the number of different triangles. Repeat this activity with 

three given line segments and discover the Triangle Inequality. 

Materials 

Teacher 

• Chalkboard compass 

• Straightedge 

Student 

• Unlined paper 


• Compass 

• Metric ruler 

• Worksheets G13(a) and (b) 

Exercise 1 

Draw two line segments on the board, one about 25 cm long and the other about 40 cm long. 

T: These two line segments have different lengths. Let’s draw a triangle using a compass and 

a straightedge. Each side of the triangle must be the same length as one of these line 

segments. 

Invite students to perform the construction at the board while the class discusses the technique. 

(See IG-V Lesson G12 Polygons #2 for a description of compass and straightedge constructions.) 

Be sure students realize that although a meter stick or metric ruler may be used for drawing straight 

line segments in these constructions, it should not be used for measuring. There are four essentially 

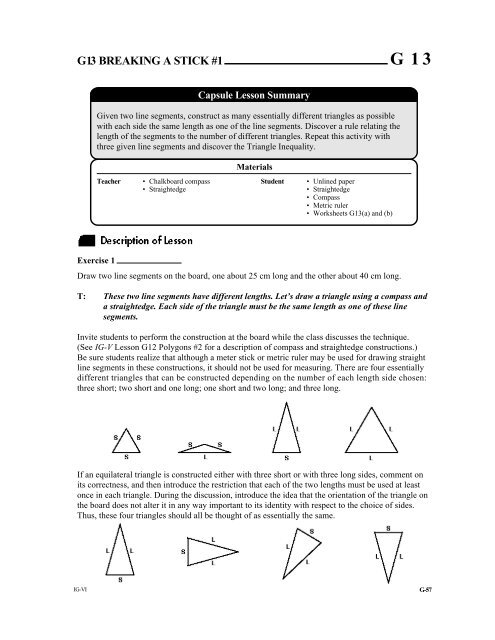
different triangles that can be constructed depending on the number of each length side chosen: 

three short; two short and one long; one short and two long; and three long. 

If an equilateral triangle is constructed either with three short or with three long sides, comment on 

its correctness, and then introduce the restriction that each of the two lengths must be used at least 

once in each triangle. During the discussion, introduce the idea that the orientation of the triangle on 

the board does not alter it in any way important to its identity with respect to the choice of sides. 

Thus, these four triangles should all be thought of as essentially the same. 

IG-VI 

G-57

G 1 3 

Once the class is secure in the triangle construction technique and there are two triangles (short-short-long 

and long-long-short) on the board, distribute copies of Worksheet G13(a) which shows five pairs of 

line segments. Direct students to construct as many triangles as possible with each pair of segments. 

Each side of a triangle must be the same length as one member of the pair, and each line segment of 

the related pair must be used at least once. The pairs are denoted A, B, C, D, and E. Instruct students 

to mark each triangle with the same letter as the pair of segments used to construct it. As students fill 

up the space on the worksheet, provide them with unlined paper. 

Encourage accurate and careful constructions. Allow time for experimentation and for the conviction 

to grow that in no case are more than two triangles possible. Encourage students to try to formulate 

a rule to predict the possibility of one or two triangles, and then to draw pairs of segments to test the 

rule. Now students may use the metric rulers for measuring in order to further test a rule based on 

relative lengths of the line segments. 

Ask several students to measure, to the nearest centimeter, the lengths of the line segments on the 

worksheet. Collect the results in a table on the board. 

Lead the discussion to elicit a rule for deciding when two triangles are possible. There are at least 

two good ways to state this rule: 

• The short segment must be more than half as long as the long segment. 

OR 

• Twice the length of the short segment must be more than the length of the long segment. 

Check students’ understanding of the rule by 

listing several pairs of lengths and asking for 

the number of possible triangles. 

Exercise 2 

Pose a triangle construction problem where three different length line segments are given. 

T: Now let’s use three segments of different lengths to draw triangles. Each segment must be 

used once in a triangle. 

G-58 IG-VI

G 1 3 

Distribute copies of Worksheet G13(b). Proceed as with Worksheet G13(a). After the individual 

work, collect the results on the board. 

Discuss the results with the purpose of formulating a rule for the possibility of constructing a 

triangle. This is a good formulation: 

• The sum of the lengths of the two shorter segments must be more than the length of the 

longest segment. (Triangle Inequality) 

Ask students to apply the rule to several sets of lengths, deciding whether or not a triangle can be 

formed. 

IG-VI 

G-59

G 1 3 

G-60 IG-VI


G 1 4 


Pose the problem of finding the probability that if we break a stick in two places, 

choosing those places randomly, the three resulting pieces will form a triangle. Examine 

many cases to decide conditions for success and for failure. Rephrase the Triangle Inequality. 

Materials 

Teacher 

• 20 cm stick or straw 


• Blacklines G14(a) and (b) 

Student 

• Colored pencils 

• Compass 

• Metric ruler 

• Unlined paper 

• Sheet with 20-cm line segments 

• Breaking points table 

• Worksheets G14* and ** 

Advance Preparation: Locate a stick that breaks easily (e.g., balsa wood) or a straw that you can cut to 

20 cm length. For students, use Blackline G14(a) to make copies of a sheet with 20 cm line segments; use 

Blackline G14(b) to makes copies of the table for recording broken points. 

Display a stick (or straw) 20 cm long. 

T: Suppose we have a stick 20 cm long and we break it in two places. What is the probability 

of being able to make a triangle with the three pieces if the breaking points are chosen at 

random? 

Let students freely discuss the problem. Some students may wish to estimate the probability; 

others to suggest ways of finding the probability; and others to discuss how to break the stick 

at two randomly chosen breaking points. At some time during the discussion, let the class select 

two breaking points on the stick. Make the breaks and try to make a triangle with the three pieces. 

Distribute copies of Blackline G14(a) to pairs of students. 

T: There are five 20-centimeter line segments drawn on this sheet. Each has two breaking 

points indicated by dots. In each case, decide whether a triangle can be formed with the 

three pieces. Use a compass and a ruler for a straightedge. 

Note: If you prefer, give student pairs five 20-cm pieces of string to cut at the breaking points indicated 

on the sheet. Then they can attempt to make triangles with the pieces of string straightened as line 

segments. 

Help students who have difficulty getting started. When most student pairs have completed four or 

five of the problems, discuss them collectively. The following illustrations show two similar methods 

of construction. 

IG-VI 

G-61

G 1 4 

T: You were able to make triangles with the segments in A and in C but not with those in B, in 

D, and in E. Why? 

S: The two short pieces together must be longer than the longest piece. 

T: Let’s measure to check what you are saying. 

S: The three lengths in A are 5 cm, 8 cm, and 7 cm. 5 + 7 is more than 8, so we can make a 

triangle. 

S: The three lengths in B are 3 cm, 5 cm, and 12 cm. 3 + 5 is less than 12, so we cannot make 

a triangle. 

T: In C, what are the three lengths? 

S: 9 cm, 2 cm, and 9 cm. 

T: So we have to compare 9 + 2 to 9 since we must use a 9 cm segment as a longest piece. 

S: 9 + 2 is more than 9, so we can make a triangle. It will be a narrow triangle. 

In a similar manner, discuss D and E. 

Begin a table on the board similar to that on Blackline G14(b). Provide students with a copy. Draw a 

20-cm line segment on the board, marking one end 0 and the other 20. Then place breaking points at 

5 cm and 14 cm. 

T: If we break the 20-cm stick 

at 5 cm and at 14 cm, what 

would the lengths of the three 

pieces be? 

S: 5 cm, 9 cm, and 6 cm. 

T: Could we make a triangle? 

S: Yes, 5 + 6 > 9. 

Record the information in the table. Instruct students to use the five 20-cm line segments on 

Blackline G14(a) to enter data in the table. Continue the table with other choices for the breaking 

points, occasionally letting students choose them. Be sure to include an example in which one of the 

three lengths is 10 cm and, hence, the sum of the lengths of the other two segments is exactly 10 cm, 

giving a failure. 

G-62 IG-VI

Your table should resemble this one. 

G 1 4 

T: If one of the breaks is at 4 cm, where could the other break be so that we could form a 

triangle? Why? 

S: At 11 cm. The lengths would be 4 cm, 7 cm, and 9 cm. 4 + 7 > 9. 

S: At 12 cm. The lengths would be 4 cm, 8 cm, and 8 cm. 4 + 8 > 8. 

Any breaking point between 10 cm and 14 cm would be a correct answer. Record this information 

in the table. 

Consider other possibilities such as having one breaking point at 13 cm, one at 10.5 cm, one at 

10.25 cm, and one at 10 cm. Only in the last case is it impossible to construct a triangle. Record 

the information in separate lines in the table, as illustrated here. 

T: When can we make a triangle? How long can the pieces be for success? 

Students may state the Triangle Inequality (as given in Lesson G13), but lead them to also notice the 

equivalent rule that each piece must be shorter than half the length of the stick (in this case, 10 cm). 

To elicit this rule, direct attention to the list of lengths in the table. Successes occur when exactly all 

lengths are less than 10 cm. In the discussion, someone might comment that if the longest length is 

more than 10 cm, then the other two pieces together must be shorter than 10 cm since the stick is 

20 cm long. So if one piece is longer than 10 cm, there is a failure. 

IG-VI 

G-63

G 1 4 

Worksheets G14* and ** are available for individual work to provide practice determining 

successful choices of points. On the ** worksheet, explain that the open dots in the example are 

used only to indicate that certain points cannot be breaking points, whereas every point between 

the open dots could be a second breaking point. 

G-64 IG-VI


G 1 5 


Establish the one-to-one correspondence between a point in a triangle and two breaking 

points on a stick. Use this correspondence to provide a means for randomly choosing two 

breaking points. 

Materials 

Teacher 

• Colored chalk 

• Meter stick 

• Grid board 

Student 

• Worksheets G15* and** 

Advance Preparation: Use Blackline G15 to make a grid, or prepare your grid board as indicated on this 

Blackline. 

On the board, draw a line segment about 1 m long, and refer to this as a “stick” in the following 

discussion. 

T: What is the “breaking a stick” problem? 

S: If you break a stick in two places to make three pieces, sometimes the three pieces can be 

used to form a triangle, and sometimes they cannot. We want to know the probability of 

forming a triangle if the breaking points are chosen at random. 

T: When will the three pieces form a triangle? 

S: When the shorter two pieces together are longer than the longest piece. 

T: With our 20-cm stick, could one of the pieces be 10 cm long? 

S: No, because together the other two pieces would be 10 cm long. When we try to make a 

triangle, the two shorter pieces collapse to a line segment 10 cm long. 

Students often use their hands in describing this situation. 

T: Could one of the pieces be longer than 10 cm? 

S: No, there would be even less of the stick left 

for the other two pieces. 

T: So what can we say about the length of each of three pieces that will form a triangle? 

S: Each is shorter than 10 cm. 

Write this requirement on the board for emphasis. Mark the midpoint of the line segment on the 

board and label it 10 cm. 

T: The problem involves a question about probability. If we choose the breaking points at 

random, what is the probability that the three pieces will make a triangle? How can we 

choose two breaking points at random? 

IG-VI G-65

G 1 5 

Let students suggest and discuss devices (spinners, darts, and so on) that might be used for choosing 

the breaking points. 

T: Name some possible breaking points and let’s see if they are successes or failures. 

S: 5 cm and 13 cm. 

Locate the breaking points. Mark them with s and label them. 

T: If we break the stick at 5 cm and at 13 cm, would the resulting pieces form a triangle? 

S: Yes; the three pieces would be 5 cm, 8 cm, and 7 cm long—each is less than 10 cm long. 

Continue the activity until five or six pairs of breaking points have been suggested and discussed. In 

the next illustration, success (you can form a triangle) is shown in blue and failure (you cannot form 

a triangle) is shown in red. 

Refer to the first pair of breaking points (5 cm and 13 cm) listed on the board. 

T: Let’s record this pair of breaking points with a blue dot at a point on the grid. Where 

should we put the dot? 

There are two points that would be natural to 

use: (5, 13) and (13, 5). Mark each with a blue 

dot and connect the two dots with a segment. 

Continue by graphing the other examples of 

breaking points listed on the board. Use blue 

or red dots according to whether the points 

give a success or a failure. 

G-66 

IG-VI

Choose a student to come to the board. Every time 

you touch a point in the picture, ask the student to 

touch the other point that could represent the same 

breaking points. Repeat the activity several times. 

G 1 5 

T: What do you notice about these pairs of points? 

S: The picture is symmetrical. 

T: Where would we place a mirror to see the symmetry? 

Invite a student to show where one would place a mirror. 

The student should trace the diagonal line segment that 

passes through the points (0, 0) and (20, 20). 

T: So that we have only one point for each pair of 

breaking points, let the first coordinate be for the 

rightmost breaking point (label the horizontal axis) 

and let the second coordinate be for the leftmost 

breaking point (label the vertical axis). Where then 

would dots for pairs of breaking points for the stick be? 

S: Below the diagonal line. 

T: But what about points on the diagonal line? 

S: They would be for the two breaking points being the same. 

T: That could happen if the breaking points were chosen at random. When the breaking 

points are the same, can we form a triangle with the pieces? 

S: No, there are only two pieces. 

Redraw the diagonal line in red. Erase the connecting cords and the dots above the diagonal. 

Trace the line segment from (20, 0) to (20, 20). 

T: What can we say about these points? 

S: They are for the rightmost breaking point being 20 cm. There would really be no break, 

because 20 cm is at the end of the stick. Also, there would not be three pieces to form a 

triangle. 

Draw the line segment from (20, 0) to (20, 20) in red. 

Likewise, conclude that all points along the line segment 

from (0, 0) to (20, 0) should be red. 

Point to a grid point below the diagonal. 

T: How can we find which breaking points this 

point on the graph represents? 

IG-VI G-67

G 1 5 

Invite a student to demonstrate the technique at the board. 

The student should project the point onto each axis. 

For example: 

T: The stick is broken at 12 cm and at 15 cm. Let 

me show you a way in which we can represent 

the stick in the picture and show the breaking 

points on the stick. (Erase the labels of the axes.) 

Let this be the stick. 

Project down as we did before to get one breaking 

point. Project to the left, but stop at the diagonal 

line and project straight down to get the second 

breaking point. 

Be sure the students observe that this method of projection 

gives the same set of breaking points. 

T: Does this dot represent a success or a failure for 

making a triangle? 

S: Failure (the answer depends on the example). 

Color the dot red for failure or blue for success. 

Demonstrate the technique with several other points, tracing without drawing. 

T: Now let’s take two breaking points on the stick 

and find the point in the triangle that represents 

them. 

Mark two breaking points on the stick, and ask for a 

volunteer to show how to find the corresponding 

point in the red triangle, or do so yourself. 

Repeat the activity with another pair of breaking points. 

T: For every pair of breaking points we can find a point in the triangle, and for every point in 

the triangle we can find a pair of breaking points. This gives us a way to choose two 

breaking points randomly—we just need to choose one point in the triangle randomly. 

How could we choose a point in the triangle randomly? 

Let students make suggestions. 

G-68 

IG-VI

Shade the interior of the (red) triangle as you say, 

G 1 5 

T: We could smear honey everywhere inside the 

triangle and set a fly loose in the room. The fly 

will choose a point at random on which to land. 

Worksheets G15* and ** are available for individual practice making projections. 

IG-VI G-69

G 1 5 

G-70 

IG-VI


G 1 6 


Recall the problem of breaking a stick. Using the fact that no piece can be as long or 

longer than half of the stick and the idea of the “honey triangle” from Lesson G15, find 

the probability of getting three pieces that form a triangle when two breaking points are 

selected at random. 

Materials 

Teacher 

• Colored chalk 

• Meter stick 

• Grid board 

Student 

• Colored pencils 

• Worksheet G16 

Advance Preparation: Use Blackline G15 to make a grid, or prepare your grid board as indicated on this 

Blackline. 

Draw the “honey triangle” from the end of Lesson G15 on the grid. 

T: Who can recall the “breaking the stick” problem? 

S: If we break a stick at two points chosen at random, what is the probability that we can 

make a triangle with the resulting three pieces? 

T: How can we choose the two points at random? 

S: By letting a fly land on the honey triangle. 

Refer students to their copies of Worksheet G16 and as you give these directions. 

T: Last week we found a one-to-one correspondence between pairs of breaking points on a 

stick and points in the honey triangle. Let’s mark some points in the triangle with blue or 

red dots. If a point corresponds to breaks resulting in three pieces that will form a triangle, 

draw a blue dot. Otherwise, draw a red dot. 

Allow about ten minutes for the students to 

mark red and blue points in the triangle on 

their worksheets. Invite students to mark red 

and blue points in the graph on the board. 

General areas of red dots and blue dots will 

become obvious. Encourage students to 

comment. If a point looks to be incorrectly 

colored, question the student who drew it and 

make necessary changes in color. After a while 

your picture will look similar to this one. 

T: Let’s look closer at the situation. If one 

of the breaking points is at 10 cm, can 

we ever make a triangle? 

IG-VI G-71

G 1 6 

S: No, because one of the pieces would be 10 cm long. 

Illustrate the two possible situations on the board. 

T: Which points in the triangle correspond to having one breaking point at 10 cm? 

Ask a student to show them in the picture on the board. 

The points lie on two line segments. Draw them in red. 

Point to the small triangle at the lower left. 

T: There are red dots in this region. Could there be 

a blue dot in this region? 

S: No; the rightmost break would be at a number 

less than 10, making the piece on the right longer 

than 10 cm. 

S: If we are to get a triangle, both breaks cannot be 

on the same half of the stick. 

Illustrate the situation. 

If some students are having difficulty, choose several points in the lower left triangular region and 

illustrate where the breaks would be in each case. When the class is convinced that no blue dot 

belongs in that small triangle, color its interior red. 

Refer to the small triangle at the upper right. 

T: There are red dots in this region. Could there be a blue dot in this region? 

S: No; the leftmost break would be at a number more than 10, making the piece on the left 

longer than 10 cm. 

S: Again, if we are to get a triangle, both breaks cannot be on the same half of the stick. 

G-72 

IG-VI

When the class is convinced that no blue dot belongs 

in the small upper-right triangle, color its interior red. 

G 1 6 

Point to the square inside the large triangle. 

T: In this region, we have some blue dots and 

some red dots. Is there any pattern? 

Perhaps a student will indicate that blue dots seem to fall 

above the diagonal from (10, 0) to (20, 0) and red dots fall 

below it. 

T: Let’s look at the situation more carefully. If the rightmost 

break is at 15, where could the leftmost break be to yield a success? 

Illustrate the cases as students discuss them. 

S: The leftmost breaking point cannot be at 5 

because then the middle segment would be 

10 cm long. 

S: The leftmost breaking point cannot be at a 

number less than 5 because then the middle 

segment would be longer than 10 cm. 

S: The leftmost breaking point can be at any 

number more than 5 but less than 10. For 

example, if the leftmost break were at 8, 

then the three pieces would be 8 cm, 7 cm, 

and 5 cm long. 

T: Why does the leftmost breaking point have to be at a number less than 10? Why not at 10? 

Why not at 12? 

S: The leftmost breaking point cannot be at 10 because then the left piece would be 10 cm 

long. 

S: The leftmost break cannot be at 12 because 

then the left piece would be longer than 10 cm. 

For a situation in which the rightmost break is at 15, 

indicate success and failure points with a line segment 

partially red and partially blue. Mark the point (15, 5) 

with a red dot. 

IG-VI G-73

G 1 6 

Continue the activity, considering each whole number between 10 and 20 as a possible rightmost 

break. Your picture should look like the one below. 

Try other choices for the rightmost breaking point, such as 12.5 and 16.25. 

A success when the rightmost break is at 12.5 results if the choice for the leftmost break is a number 

more than 2.5 but less than 10. 

The leftmost break can be anywhere between 2.5 and 10. 

A success when the righmost break is at 16.25 results if the choice for the leftmost break is a number 

more than 6.25 but less than 10. 

The leftmost break can be anywhere between 6.25 and 10. 

Add the information to the graph. 

Point to the red dots at (11, 1), (12, 2), (12.5, 2.5), (13, 3), (14, 4), …, and (20, 10). 

T: Why are the dots along this diagonal red? 

S: They are for the cases where the middle piece of the stick would be exactly 10 cm long. 

G-74 

IG-VI

G 1 6 

By now the class should suspect that solid areas of red and blue, as suggested by the red-blue 

segments, can be colored in. Shade the appropriate regions. 

T: What is the probability that a fly landing in the honey triangle will land in the blue region? 

What is the probability that if two breaking points are chosen randomly, the pieces will 

form a triangle? 

S: 1 

⁄4; the area of the blue region is one fourth of the area of the honey triangle. 

IG-VI G-75

G-76 

IG-VI

Breaking the Stick

Create successful ePaper yourself

Delete template?

Save as template?